
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




a: 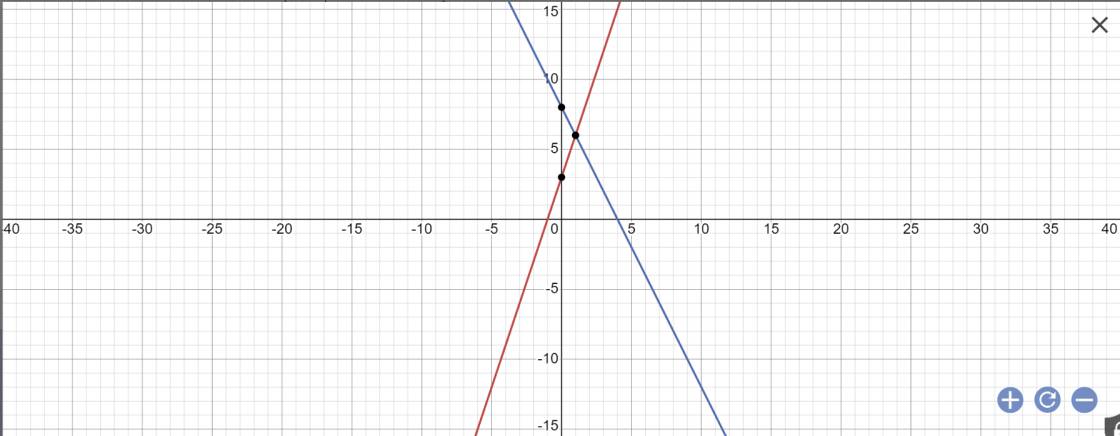
b: Tọa độ A là:
\(\left\{{}\begin{matrix}3x+3=-2x+8\\y=-2x+8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5x=5\\y=-2x+8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=1\\y=-2+8=6\end{matrix}\right.\)
Vậy: A(1;6)
Tọa độ B là:
\(\left\{{}\begin{matrix}y=0\\3x+3=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+1=0\\y=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-1\\y=0\end{matrix}\right.\)
Tọa độ C là:
\(\left\{{}\begin{matrix}y=0\\-2x+8=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=0\\-2x=-8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=4\\y=0\end{matrix}\right.\)
Vậy: A(1;6); B(-1;0); C(4;0)
\(AB=\sqrt{\left(-1-1\right)^2+\left(0-6\right)^2}=2\sqrt{10}\)
\(AC=\sqrt{\left(4-1\right)^2+\left(0-6\right)^2}=3\sqrt{5}\)
\(BC=\sqrt{\left(4+1\right)^2+\left(0-0\right)^2}=5\)
Xét ΔABC có \(cosBAC=\dfrac{AB^2+AC^2-BC^2}{2\cdot BA\cdot AC}=\dfrac{40+45-25}{2\cdot2\sqrt{10}\cdot3\sqrt{5}}=\dfrac{\sqrt{2}}{2}\)
=>\(sinBAC=\sqrt{1-\left(\dfrac{\sqrt{2}}{2}\right)^2}=\dfrac{\sqrt{2}}{2}\)
\(S_{ABC}=\dfrac{1}{2}\cdot AB\cdot AC\cdot sinBAC\)
\(=\dfrac{1}{2}\cdot\dfrac{\sqrt{2}}{2}\cdot2\sqrt{10}\cdot3\sqrt{5}=15\)

a: Tọa độ giao điểm là:
\(\left\{{}\begin{matrix}2x=1-3x\\y=2x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5x=1\\y=2x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{5}\\y=\dfrac{2}{5}\end{matrix}\right.\)
b: Thay x=1/5 và y=2/5 vào y=kx+1, ta được:
1/5k+1=2/5
=>1/5k=-3/5
hay k=-3

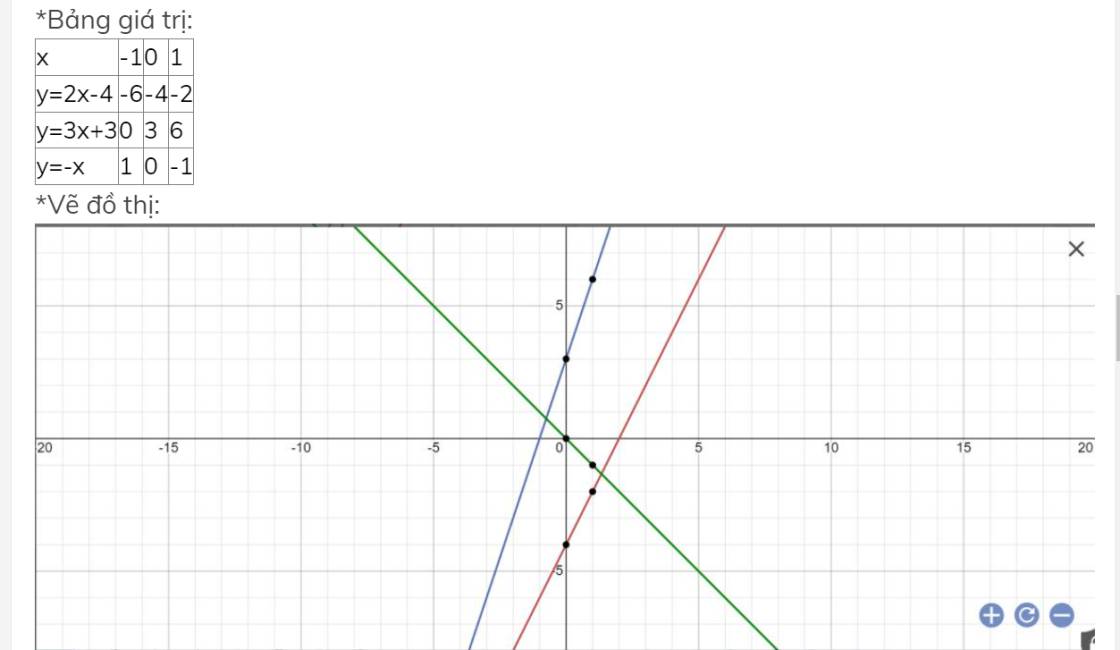
*Bảng giá trị:
| x | -1 | 0 | 1 |
| y=2x-4 | -6 | -4 | -2 |
| y=3x+3 | 0 | 3 | 6 |
| y=-x | 1 | 0 | -1 |
*Vẽ đồ thị:
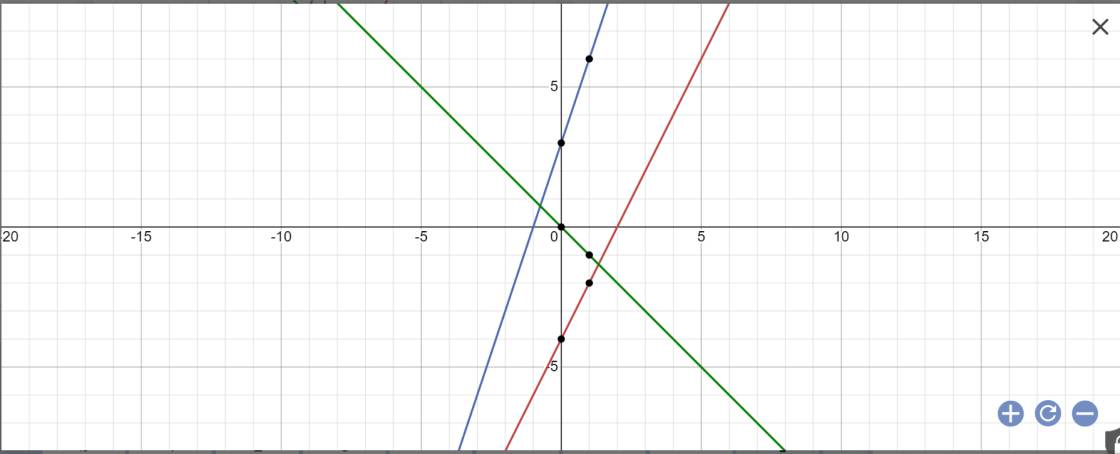

- Xét hàm số y = −2x+3
Cho x = 0 thì y = 3, ta được giao điểm của đồ thị với trục Oy là A(0;3)
Cho x = 1 thì y = 1, ta được B (1;1)
- Xét hàm số \(y = \frac{1}{2}x\)
Cho x = 0 thì y = 0, ta được điểm ở gốc tọa độ O (0;0)
x = 2 thì y = 1, ta được C(2;1)
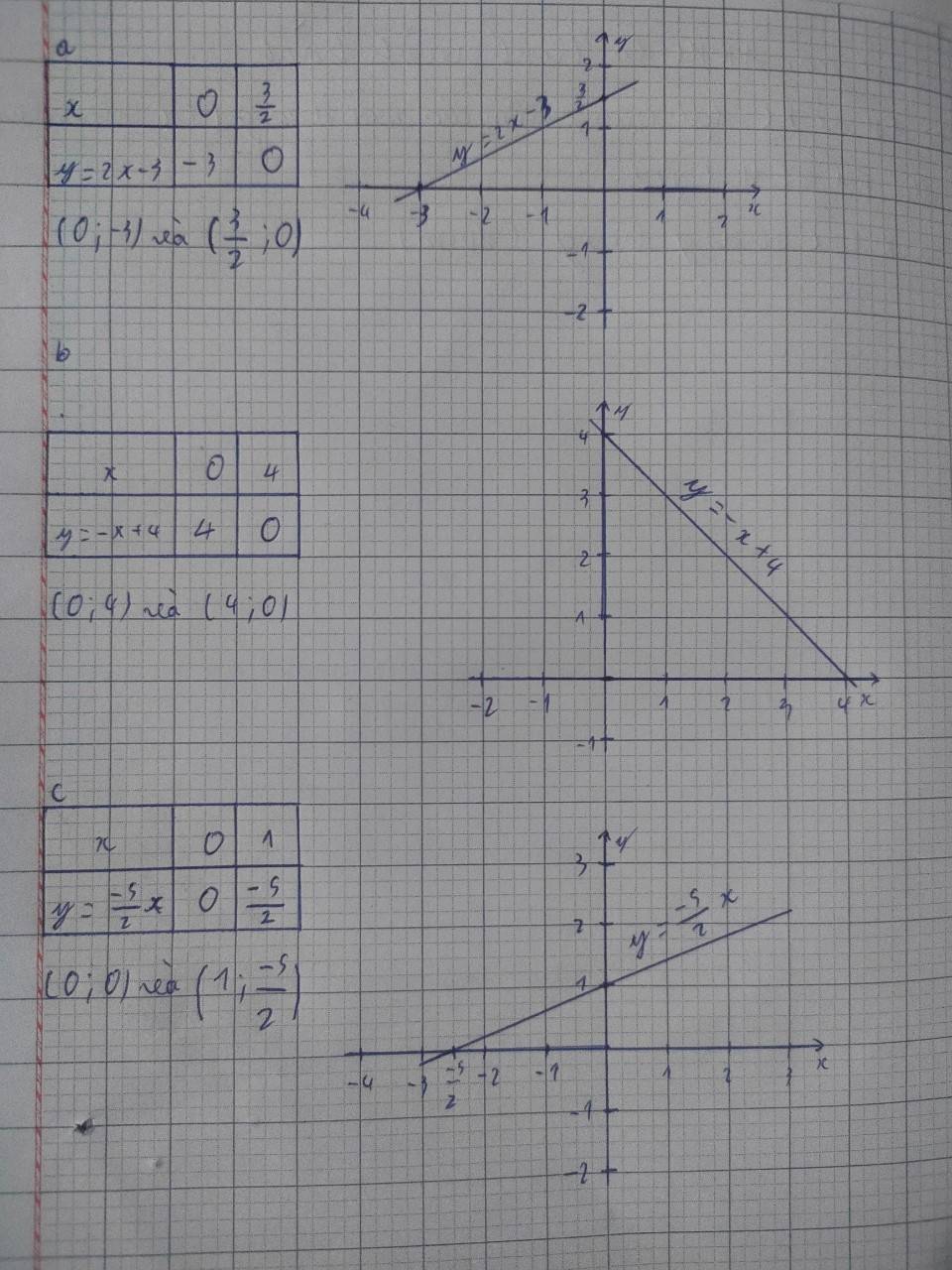
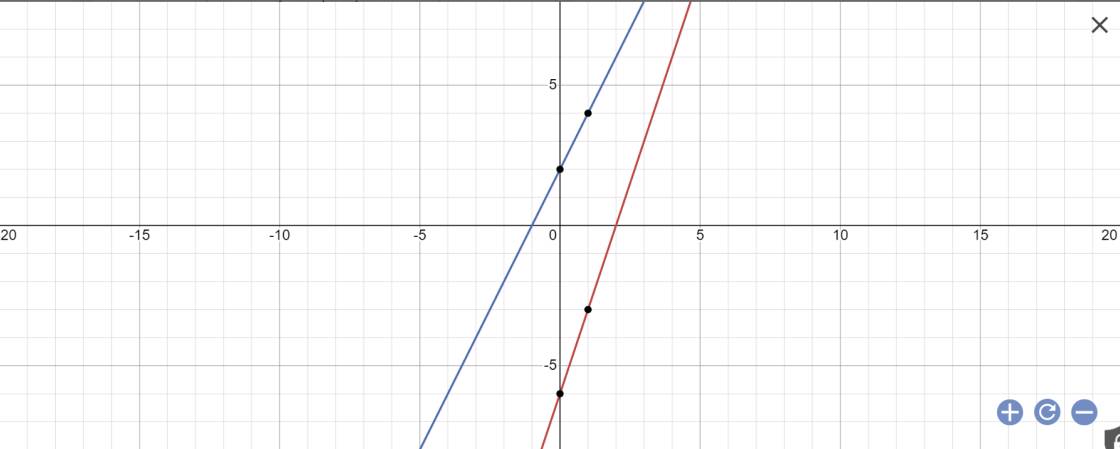
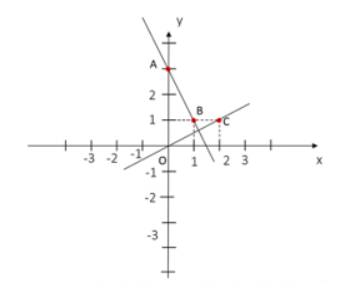
mỗi đồ thị bạn chọn ra hai điểm rồi nối lại là xong.( hai điểm đó thay vào thỏa đẳng thức )
giúp mk giải rõ vs