
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1.
a. ĐKXĐ : x lớn hơn hoặc bằng 1/2
b. A\(\sqrt{2}\)= \(\sqrt{2x+2\sqrt{2x-1}}-\sqrt{2x-2\sqrt{2x-1}}\)
= \(\sqrt{2x-1+1+2\sqrt{2x-1}}-\sqrt{2x-1+1-2\sqrt{2x-1}}\)
=\(\sqrt{\left(\sqrt{2x-1}+1\right)^2}-\sqrt{\left(\sqrt{2x-1}-1\right)^2}\)
= \(\sqrt{2x-1}+1-\left|\sqrt{2x-1}-1\right|\)
Nếu \(x\ge1thìA\sqrt{2}=\sqrt{2x-1}+1-\left(\sqrt{2x-1}-1\right)=2\)
\(\Rightarrow A=2\)
Nếu 1/2 \(\le x< 1thìA\sqrt{2}=\sqrt{2x-1}+1-\left(1-\sqrt{2x-1}\right)=2\sqrt{2x-1}\)
Do đó : A= \(\sqrt{4x-2}\)
Vậy ............
2.
a. \(x\ge2\)hoặc x<0
b. A= \(2\sqrt{x^2-2x}\)
c. A<2 \(\Leftrightarrow\)\(2\sqrt{x^2-2x}< 2\Leftrightarrow\sqrt{x^2-2x}< 1\Leftrightarrow x^2-2x< 1\Leftrightarrow\left(x-1\right)^2< 2\)
\(-\sqrt{2}< x-1< \sqrt{2}\Leftrightarrow1-\sqrt{2}< x< 1+\sqrt{2}\)
Kết hợp vs đk câu a , ta đc : \(1-\sqrt{2}< x< 0và2\le x< 1+\sqrt{2}\)
Vậy...........




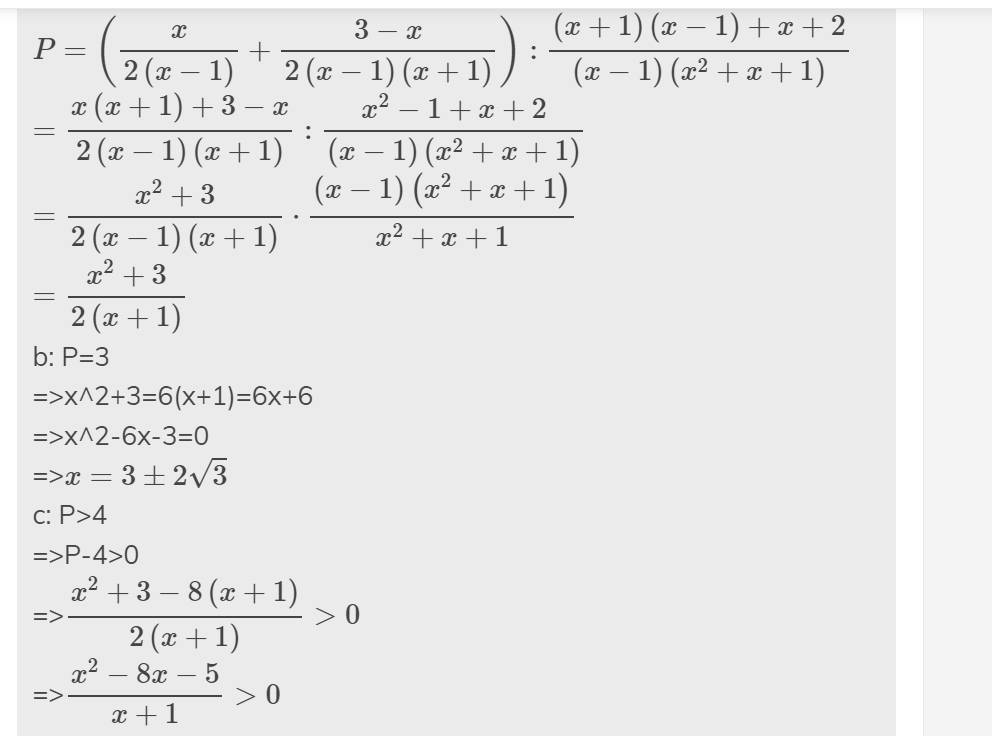
a: Sửa đề: \(P=\left(\dfrac{x}{2x-2}+\dfrac{3-x}{2x^2-2}\right):\left(\dfrac{x+1}{x^2+x+1}+\dfrac{x+2}{x^3-1}\right)\)\(P=\left(\dfrac{x}{2\left(x-1\right)}+\dfrac{3-x}{2\left(x-1\right)\left(x+1\right)}\right):\dfrac{\left(x+1\right)\left(x-1\right)+x+2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x\left(x+1\right)+3-x}{2\left(x-1\right)\left(x+1\right)}:\dfrac{x^2-1+x+2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^2+3}{2\left(x-1\right)\left(x+1\right)}\cdot\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{x^2+x+1}\)
\(=\dfrac{x^2+3}{2\left(x+1\right)}\)
b: P=3
=>x^2+3=6(x+1)=6x+6
=>x^2-6x-3=0
=>\(x=3\pm2\sqrt{3}\)
c: P>4
=>P-4>0
=>\(\dfrac{x^2+3-8\left(x+1\right)}{2\left(x+1\right)}>0\)
=>\(\dfrac{x^2-8x-5}{x+1}>0\)
TH1: x^2-8x-5>0 và x+1>0
=>x>-1 và (x<4-căn 21 hoặc x>4+căn 21)
=>-1<x<4-căn 21 hoặc x>4+căn 21
Th2: x^2-8x-5<0 và x+1<0
=>x<-1 và (4-căn 21<x<4+căn 21)
=>Vô lý

a) \(\left|2x-3\right|=\left|1-x\right|\)
\(\Leftrightarrow\orbr{\begin{cases}2x-3=1-x\\2x-3=x-1\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=\frac{4}{3}\\x=-2\end{cases}}\)
b) \(x^2-4x\le5\)
\(\Leftrightarrow x^2-4x-5\le0\)
\(\Leftrightarrow x^2-5x+x-5\le0\)
\(\Leftrightarrow x\left(x-5\right)+\left(x-5\right)\le0\)
\(\Leftrightarrow\left(x+1\right)\left(x-5\right)\le0\)
Đến đây dễ r
c) \(2x\left(2x-1\right)\le2x-1\)
\(\Leftrightarrow2x\left(2x-1\right)-\left(2x-1\right)\le0\)
\(\Leftrightarrow\left(2x-1\right)^2\le0\)
Mà \(\left(2x-1\right)^2\ge0\)nên 2x - 1=0

\(\left(2x-1\right)^2=\left|1-2x\right|=\left|2x-1\right|\left(1\right)\)
Trường hợp thứ nhất:
\(x\ge\frac{1}{2}\Rightarrow\left|2x-1\right|=2x-1\)
\(\left(1\right)\Leftrightarrow\left(2x-1\right)^2=2x-1\Leftrightarrow\left(2x-1\right)\left(x-1\right)=0\Rightarrow x\in\left\{1;\frac{1}{2}\right\}\)
Trường hợp thứ hai:
\(x< \frac{1}{2}\Rightarrow\left|2x-1\right|=1-2x\)
\(\left(1\right)\Leftrightarrow\left(2x-1\right)^2=1-2x\Leftrightarrow\left(2x-1\right)x=0\Rightarrow x=0\)
Vậy nghiệm của phương trình là \(S=\left\{0;\frac{1}{2};1\right\}\)