
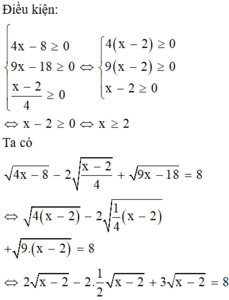
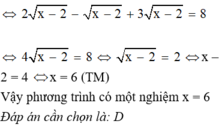
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

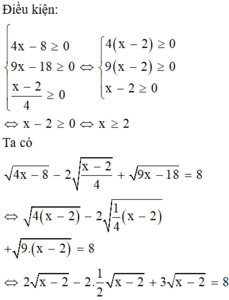
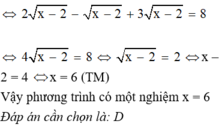

c: Ta có: \(\sqrt{x-1}+\sqrt{9x-9}-\sqrt{4x-4}=4\)
\(\Leftrightarrow2\sqrt{x-1}=4\)
\(\Leftrightarrow x-1=4\)
hay x=5
e: Ta có: \(\sqrt{4x^2-28x+49}-5=0\)
\(\Leftrightarrow\left|2x-7\right|=5\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-7=5\\2x-7=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=6\\x=1\end{matrix}\right.\)
a. ĐKXĐ: $x\in\mathbb{R}$
PT $\Leftrightarrow \sqrt{(x-2)^2}=2-x$
$\Leftrightarrow |x-2|=2-x$
$\Leftrightarrow 2-x\geq 0$
$\Leftrightarrow x\leq 2$
b. ĐKXĐ: $x\geq 2$
PT $\Leftrightarrow \sqrt{4}.\sqrt{x-2}-\frac{1}{5}\sqrt{25}.\sqrt{x-2}=3\sqrt{x-2}-1$
$\Leftrightarrow 2\sqrt{x-2}-\sqrt{x-2}=3\sqrt{x-2}-1$
$\Leftrightarrow 1=2\sqrt{x-2}$
$\Leftrightarrow \frac{1}{2}=\sqrt{x-2}$
$\Leftrightarrow \frac{1}{4}=x-2$
$\Leftrightarrow x=\frac{9}{4}$ (tm)

Câu c;d giải \(\Delta\)
Các câu còn lại là phương trình trùng phương, mình chỉ làm 1 câu thôi. Các câu sau tương tự
a/ \(x^4-2x^2-8=0\left(1\right)\)
Đặt: \(x^2=t\left(t\ge0\right)\)
\(\left(1\right)\Rightarrow t^2-2t-8=0\)
( a = 1; b = -2; c = -8 )
\(\Delta=b^2-4ac\)
\(=\left(-2\right)^2-4.1.\left(-8\right)\)
\(=36>0\)
\(\sqrt{\Delta}=\sqrt{36}=6\)
Pt có 2 nghiệm phân biệt:
\(t_1=\frac{-b-\sqrt{\Delta}}{2a}=\frac{2-6}{2.1}=-2\left(l\right)\)
\(t_2=\frac{-b+\sqrt{\Delta}}{2a}=\frac{2+6}{2.1}=4\left(n\right)\Rightarrow x^2=4\Leftrightarrow x=2hayx=-2\)
Vậy: S = {-2;2}

a) ĐK: \(x^2+7x+7\ge0\)
Đặt \(a=\sqrt{x^2+7x+7}\) \(\left(a\ge0\right)\)
PT \(\Rightarrow3a^2-3+2a=2\) \(\Leftrightarrow\left[{}\begin{matrix}a=1\\a=-\dfrac{5}{3}\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow x^2+7x+7=1\) \(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-6\end{matrix}\right.\) (Thỏa mãn)
Vậy ...
b) ĐK: \(x^2-6x+6\ge0\)
Đặt \(a=\sqrt{x^2-6x+6}\) \(\left(a\ge0\right)\)
PT \(\Rightarrow a^2+3=4a\) \(\Leftrightarrow\left[{}\begin{matrix}a=3\\a=1\end{matrix}\right.\) (Thỏa mãn)
+) Với \(a=3\) \(\Rightarrow x^2-6x+6=9\) \(\Leftrightarrow\left[{}\begin{matrix}x=3+2\sqrt{3}\\x=3-2\sqrt{3}\end{matrix}\right.\) (Thỏa mãn)
+) Với \(a=1\) \(\Rightarrow x^2-6x+6=1\) \(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=1\end{matrix}\right.\) (Thỏa mãn)
Vậy ...
c)C1: Áp dụng bđt AM-GM \(\Rightarrow VT\ge2>\dfrac{7}{4}\)
=> Dấu = ko xảy ra hay pt vô nghiệm
C2: Đk:\(x>0\)
Đặt \(a=\sqrt{\dfrac{x^2+x+1}{x}}\left(a>0\right)\) \(\Rightarrow\dfrac{1}{a}=\sqrt{\dfrac{x}{x^2+x+1}}\)
Pttt: \(a+\dfrac{1}{a}=\dfrac{7}{4}\Leftrightarrow4a^2-7a+4=0\)
\(\Delta =-15<0 \) => Pt vô nghiệm
Vậy...
d) Đk: \(x\le-8;x\ge0\)
Đặt \(t=\sqrt{x\left(8+x\right)}\left(t\ge0\right)\)
Pttt: \(t^2-3=2t\Leftrightarrow t^2-2t-3=0\Leftrightarrow\left[{}\begin{matrix}t=3\left(tm\right)\\t=-1\left(ktm\right)\end{matrix}\right.\)
\(\Rightarrow\sqrt{x\left(8+x\right)}=3\Leftrightarrow x^2+8x-9=0\) \(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-9\end{matrix}\right.\) (tm)
Vậy...

c, \(\sqrt{9x-9}-2\sqrt{x-1}=8\left(đk:x\ge1\right)\)
\(< =>\sqrt{9\left(x-1\right)}-2\sqrt{x-1}=8\)
\(< =>\sqrt{9}.\sqrt{x-1}-2\sqrt{x-1}=8\)
\(< =>3\sqrt{x-1}-2\sqrt{x-1}=8\)
\(< =>\sqrt{x-1}=8< =>\sqrt{x-1}=\sqrt{8}^2=\left(-\sqrt{8}\right)^2\)
\(< =>\orbr{\begin{cases}x-1=8\\x-1=-8\end{cases}< =>\orbr{\begin{cases}x=9\left(tm\right)\\x=-7\left(ktm\right)\end{cases}}}\)
d, \(\sqrt{x-1}+\sqrt{9x-9}-\sqrt{4x-4}=4\left(đk:x\ge1\right)\)
\(< =>\sqrt{x-1}+\sqrt{9\left(x-1\right)}-\sqrt{4\left(x-1\right)}=4\)
\(< =>\sqrt{x-1}+\sqrt{9}.\sqrt{x-1}-\sqrt{4}.\sqrt{x-1}=4\)
\(< =>\sqrt{x-1}+3\sqrt{x-1}-2\sqrt{x-1}=4\)
\(< =>\sqrt{x-1}\left(1+3-2\right)=4< =>2\sqrt{x-1}=4\)
\(< =>\sqrt{x-1}=\frac{4}{2}=2=\sqrt{2}^2=\left(-\sqrt{2}\right)^2\)
\(< =>\orbr{\begin{cases}x-1=2\\x-1=-2\end{cases}< =>\orbr{\begin{cases}x=3\left(tm\right)\\x=-1\left(ktm\right)\end{cases}}}\)

b: Ta có: \(\sqrt{x^2-6x+9}-\dfrac{\sqrt{6}+\sqrt{3}}{\sqrt{2}+1}=0\)
\(\Leftrightarrow x^2-6x+9=3\)
\(\Leftrightarrow x^2-6x+6=0\)
\(\text{Δ}=\left(-6\right)^2-4\cdot1\cdot6=36-24=12\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{6-2\sqrt{3}}{2}=3-\sqrt{3}\\x_2=3+\sqrt{3}\end{matrix}\right.\)

a) \(x^4-x^2+\dfrac{1}{4}-\dfrac{225}{4}=0\\ \left(x^2-\dfrac{1}{2}\right)^2-\dfrac{15}{2}^2=0\\ \left(x+7\right)\left(x-8\right)=0\\ \left[{}\begin{matrix}x=8\\x=-7\end{matrix}\right.\)
Vậy x = 8 hoặc x = -7
a: Ta có: \(x^4-x^2-56=0\)
\(\Leftrightarrow x^4-8x^2+7x^2-56=0\)
\(\Leftrightarrow\left(x^2-8\right)\left(x^2+7\right)=0\)
\(\Leftrightarrow x^2-8=0\)
hay \(x\in\left\{2\sqrt{2};-2\sqrt{2}\right\}\)