
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ps : Bn tự vẽ hình nhé, mk chỉ giải thôi ạ.
a) Xét \(\Delta ABC\)và \(\Delta HAB\)
\(\widehat{BAC}=\widehat{BHA}=90^O\)
\(\widehat{ABC}chung\)
\(\Rightarrow\Delta ABC~\Delta HBA\)( g - g )
b) Xét \(\Delta AHD\)và \(\Delta CED\)
\(\widehat{AHD}=\widehat{CED}=90^O\)
\(\widehat{ADH}=\widehat{CDE}\)( đối đỉnh )
\(\Rightarrow\Delta AHD~\Delta CED\left(g-g\right)\)
\(\Rightarrow\frac{AH}{AD}=\frac{CE}{CD}\Rightarrow AH.CD=AD.CE\)
c) Vì H là trung điểm của BD mà \(AH\perp BD\)
=> AH là đường trung trực của BD
\(\Rightarrow AB=AD\)
Mà : \(\frac{AH}{AD}=\frac{CE}{CD}\)
\(\Rightarrow\frac{AH}{AB}=\frac{CE}{CD}\)
Vì \(\Delta ABC~\Delta HBA\Rightarrow\frac{AH}{AB}=\frac{CA}{CB}\)
Do đó : \(\frac{CE}{CD}=\frac{CA}{CB}=\frac{8}{10}=\frac{4}{5}\)
Vì \(\Delta CED\)vuông
\(\Rightarrow S_{CED}=\frac{CE.ED}{2}\)
\(AB//FK\Rightarrow\widehat{BAH}=\widehat{KFH}\)
\(\widehat{AHB}=\widehat{FHK}=90^O\)
\(BA=HD\)
\(\Rightarrow\Delta AHB=\Delta FHK\)
\(\Rightarrow HA=HF\)mà \(CH\perp AF\)
=> CH là đường trung trực AF \(\Rightarrow\Delta ACF\)cân tại C
Do đó : D là trọng tâm \(\Delta ACF\)
\(\Rightarrow CD=\frac{2}{3}CH\)
Mà \(\cos ACB=\frac{AC}{BC}=\frac{CH}{CA}=\frac{4}{5}\Rightarrow CH=\frac{32}{5}\Rightarrow CD=\frac{64}{15}\)
\(\Rightarrow\frac{CE}{CD}=\frac{4}{5}\Rightarrow CE=\frac{256}{75}\)
\(ED=\sqrt{CD^2-CE^2}=\frac{64}{25}\)
\(\Rightarrow S_{CED}=\frac{8192}{1875}\)
d) Vì \(\Delta ACF\)cân tại C \(\Rightarrow KE//AF\Rightarrow\widehat{EKF}=\widehat{AFK}\)
Vì HK là trung tuyến \(\Delta AFK\)\(\Rightarrow\widehat{AFK}=\widehat{HKF}\)
Do đó : \(\widehat{HKF}=\widehat{EKF}\)
=> KD là phân giác \(\widehat{HKE}\)
# Aeri #

a) ĐKXĐ: \(a^2-1\ne0\Rightarrow\left(a-1\right)\left(a+1\right)\ne0\Rightarrow a\ne\pm1\)
b) ta có \(P=\frac{2a^2}{a^2-1}+\frac{a}{a+1}-\frac{a}{a-1}=\frac{2a^2+a\left(a-1\right)-a\left(a+1\right)}{a^2-1}\)
\(=\frac{2a^2+a^2-a-a^2-a}{a^2-1}=\frac{2a^2-2}{a^2-1}=\frac{2\left(a^2-1\right)}{a^2-1}=2\)

Ta có 3x^2-x+1=3x^2+2x-3x-2+3=(3x-2)(x-1)+3
D có giá trị nguyên\(\) khi 3\(⋮\)(3x+2)\(\Leftrightarrow\)3x+2 là ước của 3\(\Leftrightarrow\)3x+2\(\in\){-3;-1;1;3} suy ra x\(\in\){-5/3;-1;-1/3;1/3}mà x nguyên nên ta tìm được x=-1

a) \(x\ne2\) ; \(x\ne-2\)
b) Ta có
\(C=\frac{x^3}{x^2-4}-\frac{x}{x-2}-\frac{2}{x+2}=\frac{x^3}{\left(x-2\right)\left(x+2\right)}-\frac{x}{x-2}-\frac{2}{x+2}\)
\(=\frac{x^3-x.\left(x+2\right)-2.\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}=\frac{x^3-x^2-2x-2x+4}{\left(x-2\right)\left(x+2\right)}=\frac{x^2.\left(x-1\right)-4.\left(x-1\right)}{x^2-4}\)
\(=\frac{\left(x-1\right)\left(x^2-4\right)}{x^2-4}=x-1\)
Để C = 0 thì x-1 = 0 =>>> x=1(tm)
c) Để C nhận giá trị dương thì C thuộc Z+ = >>>>>>>> \(x-1\ge0\)=>>> \(x\ge1\)






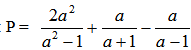




\(C\in Z\Leftrightarrow\left\{{}\begin{matrix}\dfrac{n-2}{2n+3}\in Z\left(1\right)\\\dfrac{n^2+2n+4}{2n+3}\in Z\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow n-2⋮2n+3\\ \Leftrightarrow2n-4⋮2n+3\\ \Leftrightarrow2n+3\inƯ\left(7\right)=\left\{-7;-1;1;7\right\}\\ \Leftrightarrow n\in\left\{-5;-2;-1;2\right\}\left(3\right)\\ \left(2\right)\Leftrightarrow2n^2+4n+8⋮2n+3\\ \Leftrightarrow2n\left(n+3\right)-\left(2n+3\right)+11⋮2n+3\\ \Leftrightarrow2n+3\inƯ\left(11\right)=\left\{-11;-1;1;11\right\}\\ \Leftrightarrow n\in\left\{-7;-2;-1;4\right\}\left(4\right)\\ \left(3\right)\left(4\right)\Leftrightarrow n\in\left\{-2;-1\right\}\)
Vậy ...