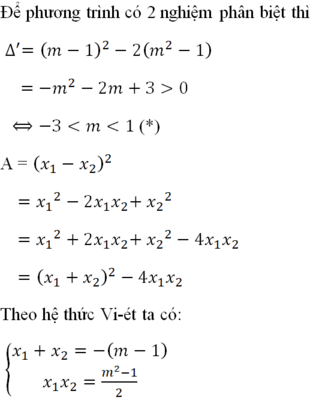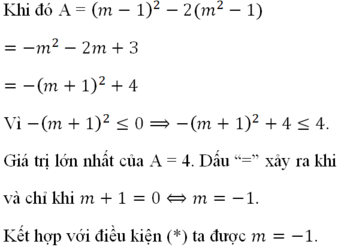Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


có :
\(\Delta'=1^2-\left(-m^2+1\right)=m^2\)
pt có \(2\) nghiệm phân biệt \(\Leftrightarrow m^2>0\Leftrightarrow m\ne0\)
\(\Rightarrow x_1=2+m;x_2=2-m\)
theo đề :
\(x_2=x^2_1\Leftrightarrow2-m=\left(2+m\right)^2\)
\(\Leftrightarrow\left(m=\dfrac{-5+\sqrt{17}}{2}\left(ktm\right)\right);\left(m=\dfrac{-5-\sqrt{17}}{2}\left(ktm\right)\right)\)
vậy không có \(m\) thỏa mãn

\(mx^2+\left(m-1\right)x+3-4m=0\left(1\right)\)
\(m=0\Rightarrow\)\(\left(1\right)\Leftrightarrow-x+3=0\Leftrightarrow x=3\left(ktm\right)\)
\(m\ne0\Rightarrow x1< 2< x2\Leftrightarrow\left\{{}\begin{matrix}\Delta>0\\\left(x1-2\right)\left(x2-2\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(m-1\right)^2-4m\left(3-4m\right)>0\\x1x2-2\left(x1+x2\right)+4< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>\dfrac{7+4\sqrt{2}}{17}\\m< \dfrac{7-4\sqrt{2}}{17}\end{matrix}\right.\\\dfrac{3-4m}{m}-2.\left(\dfrac{1-m}{m}\right)+4< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m>\dfrac{7+4\sqrt{2}}{17}\\m< \dfrac{7-4\sqrt{2}}{17}\end{matrix}\right.\\-\dfrac{1}{2}< m< 0\\\end{matrix}\right.\)\(\Rightarrow m\in\phi\)

\(\Leftrightarrow\left\{{}\begin{matrix}m+1\ne0\\\Delta'=m^2-\left(m+1\right)\left(m+6\right)>0\\x_1+x_2=\dfrac{2m}{m+1}>0\\x_1x_2=\dfrac{m+6}{m+1}>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne1\\-7m-6>0\\\dfrac{2m}{m+1}>0\\\dfrac{m+6}{m+1}>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< -\dfrac{6}{7}\\\left[{}\begin{matrix}m>0\\m< -1\end{matrix}\right.\\\left[{}\begin{matrix}m>-1\\m< -6\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow m< -6\)

Bước 1: Tìm điều kiện của tham số để phương trình có hai nghiệm phân biệt.
Bước 2: Khi phương trình đã có hai nghiệm phân biệt, ta áp dụng Vi-ét để tìm các giá trị của tham số.
Bước 3. Đối chiếu với điều kiện và kết luận bài toán.
xem tr sách của anh
Bài 1:
PT có 2 nghiệm \(\Leftrightarrow\Delta=\left(m+2\right)^2-4\cdot2\ge0\Leftrightarrow m^2+4m-8\ge0\Leftrightarrow\left[{}\begin{matrix}m\le-2-2\sqrt{3}\\m\ge-2+2\sqrt{3}\end{matrix}\right.\)
Áp dụng Viét: \(\left\{{}\begin{matrix}x_1+x_2=m+2\\x_1x_2=2\end{matrix}\right.\)
Ta có \(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}=\dfrac{9}{2}\Leftrightarrow2\left(x_1^2+x_2^2\right)=9x_1x_2\)
\(\Leftrightarrow2\left[\left(x_1+x_2\right)^2-2x_1x_2\right]=18\\ \Leftrightarrow2\left(m+2\right)^2-8=18\\ \Leftrightarrow2m^2+8m+8-8=18\\ \Leftrightarrow m^2+4m-9=0\\ \Leftrightarrow\left[{}\begin{matrix}m=-2+\sqrt{13}\\m=-2-\sqrt{13}\end{matrix}\right.\left(tm\right)\)

Để PT có 2 nghiệm \(\Leftrightarrow\Delta=\left[2\left(m+2\right)\right]^2-4\left(m^2+4\right)\ge0\)
\(\Leftrightarrow4m^2+16m+16-4m^2-16\ge0\\ \Leftrightarrow m\ge0\)
Áp dụng Viét: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+2\right)\\x_1x_2=m^2+4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=2\left(m+2\right)\left(1\right)\\x_1x_2=m^2+4\left(2\right)\\x_1+2x_2=7\left(3\right)\end{matrix}\right.\)
\(\left(3\right)-\left(1\right)=x_2=3-2m\)
Thay vào \(\left(1\right)\Leftrightarrow x_1=2\left(m+2\right)-x_2=2m+4-3+2m=4m+1\)
Thay vào \(\left(2\right)\Leftrightarrow\left(3-2m\right)\left(4m+1\right)=m^2+4\)
\(\Leftrightarrow10m+3-8m^2=m^2+4\\ \Leftrightarrow9m^2-10m+1=0\\ \Leftrightarrow\left[{}\begin{matrix}m=1\\m=\dfrac{1}{9}\end{matrix}\right.\left(tm\right)\)