Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Giá trị lớn nhất:
\(A=2x-3x^2-4=-3\left(x^2-\frac{2}{3}x+\frac{4}{3}\right)=-3\left[x^2-2.x.\frac{1}{3}+\left(\frac{1}{3}\right)^2+\frac{35}{9}\right]=-3\left(x-\frac{1}{3}^2\right)-\frac{35}{3}\)
Vì \(\left(x-\frac{1}{3}\right)^2\ge0\left(x\in R\right)\)
Nên \(-3\left(x-\frac{1}{3}\right)^2\le0\left(x\in R\right)\)
do đó \(-3\left(x-\frac{1}{3}\right)^2-\frac{35}{3}\le-\frac{35}{3}\left(x\in R\right)\)
Vậy \(Max_A=-\frac{35}{3}\)khi \(x-\frac{1}{3}=0\Rightarrow x=\frac{1}{3}\)
\(B=-x^2-4x=-\left(x^2+4x\right)=-\left(x^2+2.x.2+2^2-2^2\right)=-\left(x+2\right)^2+4\)
Vì \(\left(x+2\right)^2\ge0\left(x\in R\right)\)
nên \(-\left(x+2\right)^2\le0\left(x\in R\right)\)
do đó \(-\left(x+2\right)^2+4\le4\left(x\in R\right)\)
Vậy \(Max_B=4\)khi \(x+2=0\Rightarrow x=-2\)
b) Giá trị nhỏ nhất
\(A=x^2-2x-1=x^2-2.x.+1-2=\left(x-1\right)^2-2\)
Vì \(\left(x-1\right)^2\ge0\left(x\in R\right)\)
nên \(\left(x-1\right)^2-2\ge-2\left(x\in R\right)\)
Vậy \(Min_A=-2\)khi \(x-1=0\Rightarrow x=1\)
\(B=4^2+4x+5=\left(2x\right)^2+2.2x.1+1+4=\left(2x+1\right)^2+4\)
vì \(\left(2x+1\right)^2\ge0\left(x\in R\right)\)
nên \(\left(2x+1\right)^2+4\ge4\left(x\in R\right)\)
Vậy \(Min_B=4\)khi \(2x+1=0\Rightarrow x=-\frac{1}{2}\)

a: \(A=2x^2-8x+1\)
\(=2\left(x^2-4x+\dfrac{1}{2}\right)\)
\(=2\left(x^2-4x+4-\dfrac{7}{2}\right)\)
\(=2\left(x-2\right)^2-7>=-7\)
Dấu = xảy ra khi x=2
b: \(B=\left(x-3\right)^2+\left(x-1\right)^2\)
\(=x^2-6x+9+x^2-2x+1\)
\(=2x^2-8x+10\)
\(=2x^2-8x+8+2\)
\(=2\left(x-2\right)^2+2>=2\)
Dấu = xảy ra khi x=2

c: \(-x^2+2x-2=-\left(x-1\right)^2-1\le-1\forall x\)
\(\Leftrightarrow V\ge-1\forall x\)
Dấu '=' xảy ra khi x=1

a) \(A=-x^2+2x=-\left(x^2-2x+1\right)+1=-\left(x-1\right)^2+1\le1\)
\(maxA=1\Leftrightarrow x=1\)
b) \(B=\left(2-3x\right)\left(3+2x\right)=-6x^2-5x+6=-6\left(x^2+\dfrac{5}{6}x+\dfrac{25}{144}\right)+\dfrac{169}{24}=-6\left(x+\dfrac{5}{12}\right)^2+\dfrac{169}{24}\le\dfrac{169}{24}\)
\(minB=\dfrac{169}{24}\Leftrightarrow x=-\dfrac{5}{12}\)
c) \(C=4xy-4x-2y-4x^2-2y^2-3=-\left[4x^2-4x\left(y-1\right)+\left(y-1\right)^2\right]+\left(y^2-4y+4\right)-6=\left(2x-y+1\right)^2+\left(y-2\right)^2-6\le-6\)
\(minC=-6\Leftrightarrow\)\(\left\{{}\begin{matrix}x=\dfrac{1}{2}\\y=2\end{matrix}\right.\)

Tính giá trị nhỏ nhất:
\(A=x^2-4x+1=(x^2-4x+4)-3=(x-2)^2-3\)
Vì $(x-2)^2\geq 0, \forall x\in\mathbb{R}$ nên $A=(x-2)^2-3\geq 0-3=-3$
Vậy $A_{\min}=-3$
Giá trị này đạt tại $(x-2)^2=0\Leftrightarrow x=2$
$B=4x^2+4x+11=(4x^2+4x+1)+10=(2x+1)^2+10\geq 0+10=10$
Vậy $B_{\min}=10$
Giá trị này đạt tại $(2x+1)^2=0\Leftrightarrow x=-\frac{1}{2}$
$C=(x-1)(x+3)(x+2)(x+6)$
$=(x-1)(x+6)(x+3)(x+2)$
$=(x^2+5x-6)(x^2+5x+6)$
$=(x^2+5x)^2-36\geq 0-36=-36$
Vậy $C_{\min}=-36$. Giá trị này đạt $x^2+5x=0\Leftrightarrow x=0$ hoặc $x=-5$
Tìm giá trị lớn nhất:
$D=5-8x-x^2=21-(x^2+8x+16)=21-(x+4)^2$
Vì $(x+4)^2\geq 0, \forall x\in\mathbb{R}$ nên $D=21-(x+4)^2\leq 21$
Vậy $D_{\max}=21$. Giá trị này đạt tại $(x+4)^2=0\Leftrightarrow x=-4$
$E=4x-x^2+1=5-(x^2-4x+4)=5-(x-2)^2\leq 5$
Vậy $E_{\max}=5$. Giá trị này đạt tại $(x-2)^2=0\Leftrightarrow x=2$

\(I=-\left(x-1\right)\left(x+6\right)\left(x+2\right)\left(x+3\right)+2021\)
\(=-\left(x^2+5x-6\right)\left(x^2+5x+6\right)+2021\)
\(=-\left[\left(x^2+5x\right)^2-6^2\right]+2021\)
\(=-\left(x^2+5x\right)^2+2057\le2057\)
\(I_{max}=2057\) khi \(x^2+5x=0\)
\(K=-\left(x-2\right)\left(x-7\right)\left(x-5\right)\left(x-4\right)+102\)
\(=-\left(x^2-9x+14\right)\left(x^2-9x+20\right)+102\)
\(=-\left(x^2-9x+14\right)\left(x^2+9x+14+6\right)+102\)
\(=-\left[\left(x^2-9x+14\right)^2+6\left(x^2-9x+14\right)\right]+102\)
\(=-\left[\left(x^2-9x+14\right)+6\left(x^2-9x+14\right)+9-9\right]+102\)
\(=-\left(x^2-9x+17\right)^2+111\le111\)
\(K_{max}=111\) khi \(x^2-9x+17=0\)
\(M=-\left(4x^2+4x+1\right)\left(16x^2+16x+3\right)-11\)
Đặt \(4x^2+4x+1=t\Rightarrow16x^2+16x=4t-4\)
\(\Rightarrow M=-t\left(4t-4+3\right)-11\)
\(M=-4t^2+t-11\)
\(M=-4\left(t-\dfrac{1}{8}\right)^2-\dfrac{175}{16}\le-\dfrac{175}{16}\)
\(M_{max}=-\dfrac{175}{16}\) khi \(t=\dfrac{1}{8}\)

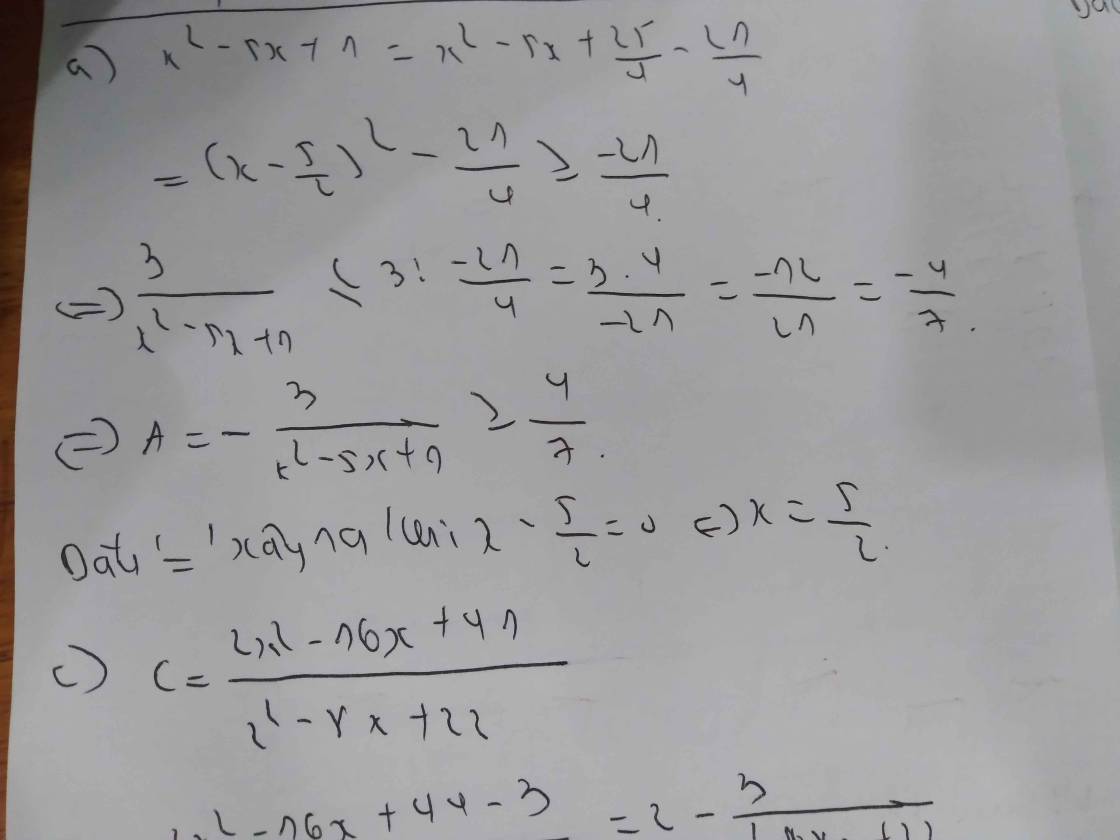
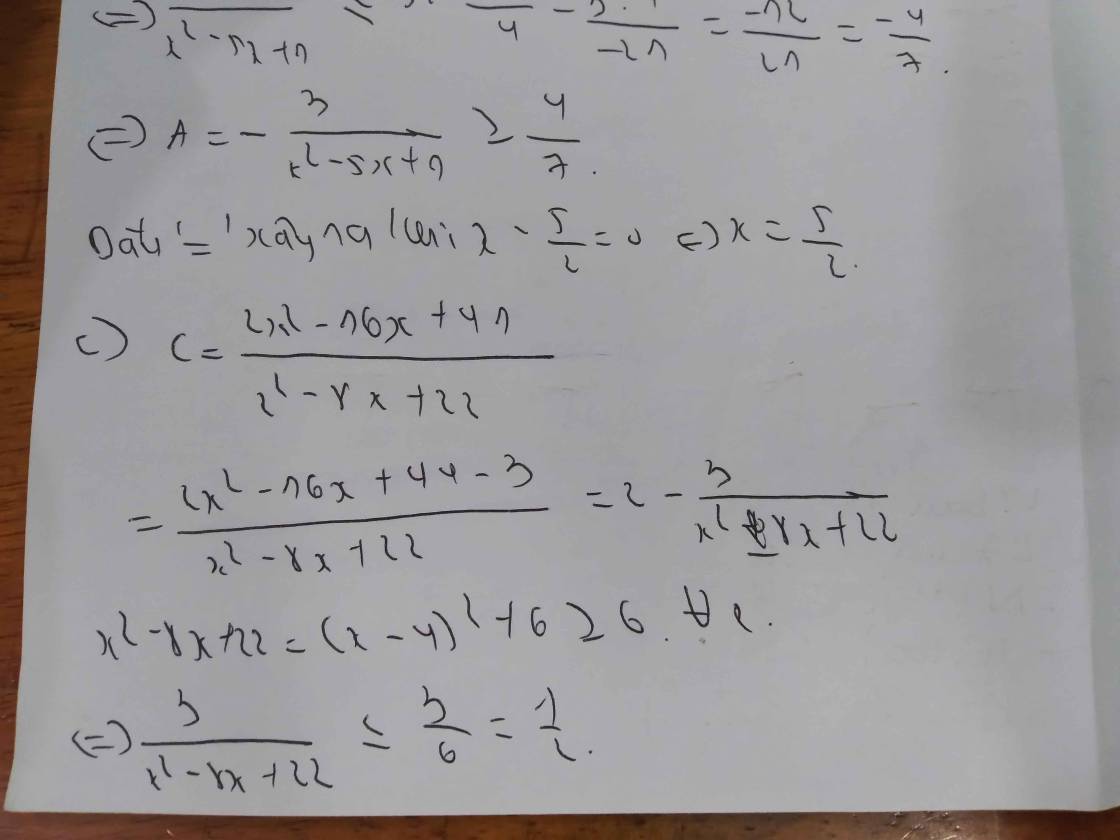
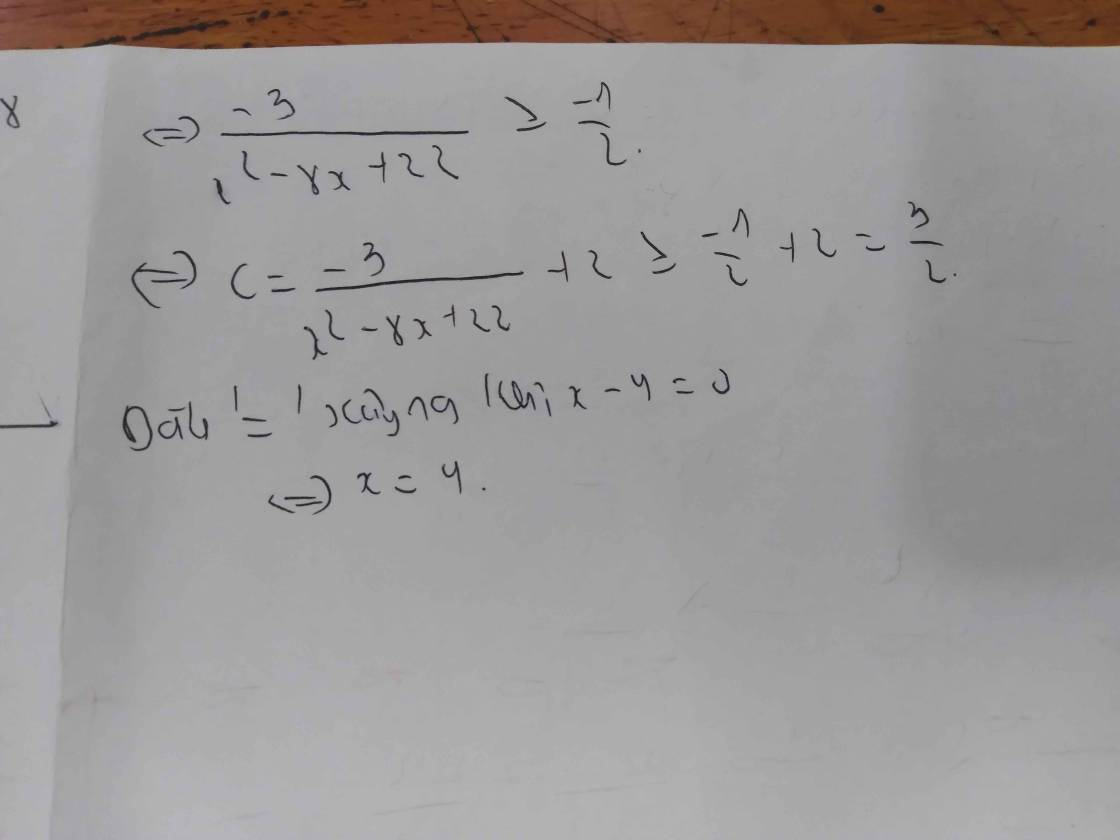
a) \(P=4x^2-4x-1=\left(4x^2-4x+1\right)-2=\left(2x-1\right)^2-2\ge-2\)
Vậy Min P = - 2 khi x = 1/2
b) \(P=3x^2-12x-3=3\left(x^2-4x+4\right)-15=3\left(x-2\right)^2-15\ge-15\)
Vậy Min P = -15 khi x = 2
c) \(P=\left(x+1\right)\left(2x-1\right)=2x^2+x-1=2\left(x+\frac{1}{4}\right)^2-\frac{9}{8}\ge-\frac{9}{8}\)
Vậy Min P = -9/8 khi x = -1/4