Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(A=\left(a^4-2a^3+a^2\right)+2\left(a^2-2a+1\right)+3\)
\(A=\left(a^2-a\right)^2+2\left(a-1\right)^2+3\ge3\)
\(A_{min}=3\) khi \(a=1\)
https://hoc24.vn/cau-hoi/co-the-dung-mot-can-dia-co-hai-dia-can-voi-nam-qua-cancac-qua-can-chi-de-o-mot-dia-can-de-can-tat-ca-cac-vat-co-khoi-luong-la-mot-so-tu-nhien-tu-1kg-den-30kg-duoc-khongcac-ban-giai-giup-mk-voi.341565384997
Thầy giải giúp e với ạ,e cảm ơn thầy ạ! <3

Bài 1:
\(\left\{{}\begin{matrix}a=5c+1\\b=5d+2\end{matrix}\right.\)
\(a^2+b^2=\left(5c+1\right)^2+\left(5d+2\right)^2\)
\(=25c^2+10c+1+25d^2+20d+4\)
\(=25c^2+25d^2+10c+20d+5\)
\(=5\left(5c^2+5d^2+2c+4d+1\right)⋮5\)
Bài 3:
a: \(4x^2+12x+15=4x^2+12x+9+6=\left(2x+3\right)^2+6>=6\forall x\)
Dấu '=' xảy ra khi x=-3/2
b: \(9x^2-6x+5=9x^2-6x+1+4=\left(3x-1\right)^2+4>=4\forall x\)
Dấu '=' xảy ra khi x=1/3

Bài 1:
a: \(A=x^2-30x+225-114=\left(x-15\right)^2-114>=-114\forall x\)
Dấu '=' xảy ra khi x=15
b: \(B=4a^2+4a+1+1=\left(2a+1\right)^2+1>=1\forall a\)
Dấu '=' xảy ra khi a=-1/2
Bài 2:
a: \(A=-\left(x^2-4x-3\right)=-\left(x^2-4x+4-7\right)=-\left(x-2\right)^2+7< =7\forall x\)
Dấu '=' xảy ra khi x=2

A = (2x)2 - 4x +1 +4 = (2x-1)2 +4
GTNN A = 4
(hic,đăng 5h rùi mà k ai giúp ,đơn giản k, dễ hiu k, bn hiền)

\(A=a^4-2a^3+3a^2-4a+5\)
\(\Leftrightarrow A=a^4-2a^3+a^2+2a^2-4a+2+3\)
\(\Leftrightarrow A=\left(a^4-2a^3+^2\right)+2\left(a^2-2a+1\right)+3\)
\(\Leftrightarrow A=\left(a^2-a\right)^2+2\left(a-1\right)^2+3\)
Có:\(\hept{\begin{cases}\left(a^2-a\right)^2\ge0\forall x\\2\left(a-1\right)^2\ge0\forall x\end{cases}}\)
\(\Rightarrow A\ge3\). Dấu "=" \(\Leftrightarrow\hept{\begin{cases}a^2-a=0\\a-1=0\end{cases}\Leftrightarrow\hept{\begin{cases}a^2=a\\a=1\end{cases}}}\)
Vậy Min A=3 đạt được khi a=1
Nguồn: DORAEMON (lazi.vn)

Câu hỏi của Edogawa Conan - Toán lớp 8 - Học toán với OnlineMath
Em tham khảo.
Phân tích thành tích: x^4+2015x^2+2014x+2015
Tìm giá trị nhỏ nhất của biểu thức A =a^4-2a^3+3a^2-4a+5

Câu 1 nha bạn
x^4 + x^3 + x^2 + 2014x^2 + 2014x + 2014 + 1 - x^3
=> x^4 + x^3 + x^2 + 2014x^2 + 2014x + 2014 - x^3 - 1
=> x^2 ( x^2 + x + 1 ) + 2014 ( x^2 + x + 1 ) - ( x - 1 )( x^2 + x + 1 )
=> ( x^2 + x + 1 )( x^2 + 2014 - x - 1)

\(A=4x^2-12x+9-\left(x^2+6x+5\right)+2\)
\(=3x^2-18x+6\)
\(=3\left(x^2-6x+9\right)-21\)
\(=3\left(x-3\right)^2-21\ge-21\)
\(A_{min}=-21\) khi \(x=3\)
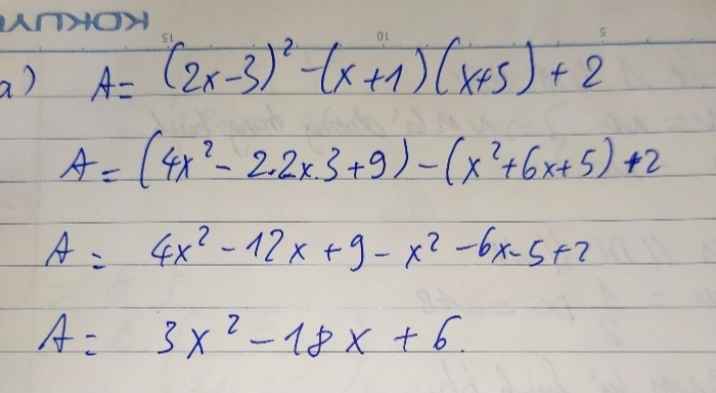

có A = \(a^4-2a^3+3a^2-4a+5\)
\(\Leftrightarrow A=\left(a^2\right)^2-2a^2.a+a^2+2a^2-4a+2+3\)
\(\Leftrightarrow A=\left(a^2-a\right)^2+\left(\sqrt{2}.a-\sqrt{2}\right)^2+3\)
\(\Rightarrow\) A luôn luôn lớn hơn hoặc bằng 3 với mọi giá trị của x
=> giá trị nhỏ nhất của A = 3 khi
( \(\left(a^2-a\right)^2=0\) \(\Leftrightarrow a^2-a=0\Leftrightarrow a\left(a-1\right)=0\) )
\(\Rightarrow\) a= 0 hoặc a= 1