Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a, \(\dfrac{4x+13}{5x\left(x-7\right)}-\dfrac{x-48}{5x\left(7-x\right)}\)
\(=\dfrac{4x+13}{5x\left(x-7\right)}+\dfrac{x-48}{5x\left(x-7\right)}\)
\(=\dfrac{4x+13+x-48}{5x\left(x-7\right)}\)
\(=\dfrac{5x-35}{5x\left(x-7\right)}\)
\(=\dfrac{5\left(x-7\right)}{5x\left(x-7\right)}=\dfrac{1}{x}\)
b, \(\dfrac{1}{x-5x^2}-\dfrac{25x-15}{25x^2-1}\)
\(=\dfrac{1}{x\left(1-5x\right)}+\dfrac{25x-15}{\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x}{x\left(x-5x\right)\left(1+5x\right)}+\dfrac{x\left(25x-15\right)}{x\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{1+5x+25x^2-15x}{x\left(1-5x\right)\left(1+5x\right)}\)\(=\dfrac{25x^2-10x+1}{x\left(1-5x\right)\left(1+5x\right)}=\dfrac{\left(5x-1\right)^2}{x.\left(1-5x\right)\left(1+5x\right)}\)
\(=\dfrac{\left(5x-1\right)^2}{-x\left(5x-1\right)\left(1+5x\right)}\) \(=\dfrac{-\left(5x-1\right)}{x\left(1+5x\right)}\)

b: \(=\dfrac{-1}{x\left(5x-1\right)}-\dfrac{25x-15}{\left(5x-1\right)\left(5x+1\right)}\)
\(=\dfrac{-5x-1-25x^2+15x}{x\left(5x-1\right)\left(5x+1\right)}\)
\(=\dfrac{-25x^2-10x-1}{x\left(5x-1\right)\left(5x+1\right)}=\dfrac{-\left(5x+1\right)}{x\left(5x-1\right)}\)
c: \(=\dfrac{x+9y}{\left(x-3y\right)\left(x+3y\right)}-\dfrac{3y}{x\left(x-3y\right)}\)
\(=\dfrac{x^2+9xy-3xy-9y^2}{x\left(x-3y\right)\left(x+3y\right)}\)
\(=\dfrac{x^2+6xy-9y^2}{x\left(x-3y\right)\left(x+3y\right)}\)
d: \(=\dfrac{3x+1}{\left(x-1\right)^2}-\dfrac{1}{x+1}+\dfrac{x+3}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{3x^2+4x+1-x^2+2x-1+x^2+2x-3}{\left(x-1\right)^2\cdot\left(x+1\right)}\)
\(=\dfrac{3x^2+8x-3}{\left(x-1\right)^2\cdot\left(x+1\right)}=\dfrac{3x^2+9x-x-3}{\left(x-1\right)^2\cdot\left(x+1\right)}\)
\(=\dfrac{3x+1}{\left(x-1\right)\left(x+1\right)}\)

a) \(x^2-16=0\Rightarrow x^2=16\Rightarrow x^2=\pm4\)
b) \(4x^2-9=0\Rightarrow\left(2x-3\right)\left(2x+3\right)=0\Rightarrow x=\pm1,5\)
c) \(25x^2-1=0\Rightarrow\left(5x-1\right)\left(5x+1\right)=0\Rightarrow x=\pm0,2\)
d) \(4\left(x-1\right)^2-9=0\Rightarrow\left(2x-2-3\right)\left(2x-2+3\right)=0\Rightarrow\left[{}\begin{matrix}2x-5=0\Rightarrow x=2,5\\2x+1=0\Rightarrow x=-0,5\end{matrix}\right.\)
e) \(25x^2-\left(5x+1\right)^2=0\Rightarrow\left(5x+5x+1\right)\left(5x-5x-1\right)=0\Rightarrow10x+1=0\Rightarrow x=-0,1\)
f) \(\dfrac{1}{4}-9\left(x-1\right)^2=0\Rightarrow\left(\dfrac{1}{2}+3x-3\right)\left(\dfrac{1}{2}-3x+3\right)=0\Rightarrow\left[{}\begin{matrix}x=\dfrac{5}{6}\\x=\dfrac{7}{6}\end{matrix}\right.\)
g) \(\dfrac{1}{16}-\left(2x+\dfrac{3}{4}\right)^2=0\Rightarrow\left(\dfrac{1}{4}+2x+\dfrac{3}{4}\right)\left(\dfrac{1}{4}-2x-\dfrac{3}{4}\right)=0\Rightarrow\left[{}\begin{matrix}x=-0,5\\x=-0,25\end{matrix}\right.\)
h) \(\dfrac{1}{9}x^2-\dfrac{2}{3}x+1=0\Rightarrow\left(\dfrac{1}{3}x-1\right)^2=0\Rightarrow\dfrac{1}{3}x=1\Rightarrow x=3\)
k) \(4\left(x-3\right)^2-\left(2-3x\right)^2=0\Rightarrow\left(2x-6+2-3x\right)\left(2x-6-2+3x\right)=0\Rightarrow\left[{}\begin{matrix}-x-4=0\Rightarrow x=-4\\5x-8=0\Rightarrow x=1,6\end{matrix}\right.\)
l) \(x^2-x-12=0\Rightarrow x^2-4x+3x-12=0\Rightarrow x\left(x-4\right)+3\left(x-4\right)=0\Rightarrow\left(x+3\right)\left(x-4\right)=0\Rightarrow\left[{}\begin{matrix}x=-3\\x=4\end{matrix}\right.\)

Bài 2 .
a) \(\dfrac{2x}{x^2+2xy}+\dfrac{y}{xy-2y^2}+\dfrac{4}{x^2-4y^2}\)
\(=\dfrac{2x}{x\left(x+2y\right)}+\dfrac{y}{y\left(x-2y\right)}+\dfrac{4}{\left(x-2y\right)\left(x+2y\right)}\)
\(=\dfrac{2xy\left(x-2y\right)+xy\left(x+2y\right)+4xy}{xy\left(x+2y\right)\left(x-2y\right)}\)
\(=\dfrac{2x^2y-2xy^2+x^2y+2xy^2+4xy}{xy\left(x+2y\right)\left(x-2y\right)}\)
\(=\dfrac{3x^2y+4xy}{xy\left(x+2y\right)\left(x-2y\right)}\)
b) Sai đề hay sao ý
c) \(\dfrac{2x+y}{2x^2-xy}+\dfrac{16x}{y^2-4x^2}+\dfrac{2x-y}{2x^2+xy}\)
\(=\dfrac{2x+y}{x\left(2x-y\right)}+\dfrac{-16x}{\left(2x-y\right)\left(2x+y\right)}+\dfrac{2x-y}{x\left(2x+y\right)}\)
\(=\dfrac{\left(2x+y\right)^2-16x^2+\left(2x-y\right)^2}{x\left(2x-y\right)\left(2x+y\right)}\)
\(=\dfrac{4x^2+4xy+y^2-16x^2+4x^2-4xy+y^2}{x\left(2x-y\right)\left(2x+y\right)}\)
\(=\dfrac{-8x^2}{x\left(2x-y\right)\left(2x+y\right)}\)
d) \(\dfrac{1}{1-x}+\dfrac{1}{1+x}+\dfrac{2}{1+x^2}+\dfrac{4}{1+x^4}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{2}{1-x^2}+\dfrac{2}{1+x^2}+\dfrac{4}{1+x^4}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{4}{1-x^4}+\dfrac{4}{1+x^4}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
.....
\(=\dfrac{16}{1-x^{16}}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{32}{1-x^{32}}\)

1) \(\dfrac{x^2}{x+1}+\dfrac{2x}{x^2-1}-\dfrac{1}{1-x}+1\)
\(=\dfrac{x^2}{x+1}+\dfrac{2x}{x^2-1}+\dfrac{1}{x-1}+1\)
\(=\dfrac{x^2}{x+1}+\dfrac{2x}{\left(x-1\right)\left(x+1\right)}+\dfrac{1}{x-1}+1\) MTC: \(\left(x-1\right)\left(x+1\right)\)
\(=\dfrac{x^2\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}+\dfrac{2x}{\left(x-1\right)\left(x+1\right)}+\dfrac{x+1}{\left(x-1\right)\left(x+1\right)}+\dfrac{\left(x-1\right)\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x^2\left(x-1\right)+2x+\left(x+1\right)+\left(x-1\right)\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x^3-x^2+2x+x+1+x^2-1}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x\left(x^2+3\right)}{\left(x-1\right)\left(x+1\right)}\)
b) \(\dfrac{1}{x^3-x}-\dfrac{1}{\left(x-1\right)x}+\dfrac{2}{x^2-1}\)
\(=\dfrac{1}{x\left(x^2-1\right)}-\dfrac{1}{\left(x-1\right)x}+\dfrac{2}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{1}{x\left(x-1\right)\left(x+1\right)}-\dfrac{1}{\left(x-1\right)x}+\dfrac{2}{\left(x-1\right)\left(x+1\right)}\) MTC: \(x\left(x-1\right)\left(x+1\right)\)
\(=\dfrac{1}{x\left(x-1\right)\left(x+1\right)}-\dfrac{x+1}{x\left(x-1\right)\left(x+1\right)}+\dfrac{2x}{x\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{1-\left(x+1\right)+2x}{x\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{1-x-1+2x}{x\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x}{x\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{1}{\left(x-1\right)\left(x+1\right)}\)

a: \(\Leftrightarrow-12x-4=8x-2-8-6x\)
=>-12x-4=2x-10
=>-14x=-6
hay x=3/7
b: \(\Leftrightarrow3\left(5x-3\right)-2\left(5x-1\right)=-4\)
=>15x-9-10x+2=-4
=>5x-7=-4
=>5x=3
hay x=3/5(loại)
c: \(\Leftrightarrow x^2-4+3x+3=3+x^2-x-2\)
\(\Leftrightarrow x^2+3x-1=x^2-x+1\)
=>4x=2
hay x=1/2(nhận)

\(1.\)
\(a.\)
\(\dfrac{8}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{2}{x^2+3}+\dfrac{1}{x+1}\)
\(=\dfrac{8}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{2\left(x^2-1\right)}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{1\left(x-1\right)\left(x^2+3\right)}{\left(x^2-1\right)\left(x^2+3\right)}\)
\(=\dfrac{8}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{2x^2-2}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{x^3-x^2+3x-3}{\left(x^2-1\right)\left(x^2+3\right)}\)
\(=\dfrac{8+2x^2-2+x^3-x^2+3x-3}{\left(x^2+3\right)\left(x^2-1\right)}\)
\(=\dfrac{x^3+x^2+3x+3}{\left(x^2+3\right)\left(x^2-1\right)}\)
\(=\dfrac{x^2\left(x+1\right)+3\left(x+1\right)}{\left(x^2+3\right)\left(x^2-1\right)}\)
\(=\dfrac{\left(x^2+3\right)\left(x+1\right)}{\left(x^2+3\right)\left(x^2-1\right)}\)
\(=x-1\)
\(b.\)
\(\dfrac{x+y}{2\left(x-y\right)}-\dfrac{x-y}{2\left(x+y\right)}+\dfrac{2y^2}{x^2-y^2}\)
\(=\dfrac{x+y}{2\left(x-y\right)}-\dfrac{x-y}{2\left(x+y\right)}+\dfrac{2y^2}{\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{\left(x+y\right)^2}{2\left(x^2-y^2\right)}-\dfrac{\left(x-y\right)^2}{2\left(x^2-y^2\right)}+\dfrac{4y^2}{2\left(x^2-y^2\right)}\)
\(=\dfrac{x^2+2xy+y^2}{2\left(x^2-y^2\right)}-\dfrac{x^2-2xy+y^2}{2\left(x^2-y^2\right)}+\dfrac{4y^2}{2\left(x^2-y^2\right)}\)
\(=\dfrac{x^2+2xy+y^2-x^2+2xy-y^2+4y^2}{2\left(x^2-y^2\right)}\)
\(=\dfrac{4xy+4y^2}{2\left(x^2-y^2\right)}\)
\(=\dfrac{4y\left(x+y\right)}{2\left(x^2-y^2\right)}\)
\(=\dfrac{2y}{\left(x-y\right)}\)
Tương tự các câu còn lại

a) \(\dfrac{5x-1}{3x+2}=\dfrac{5x-7}{3x-1}\)
Áp dụng tính chất dãy tỉ số bằng nhau ta được:
\(\dfrac{5x-1}{3x+2}=\dfrac{5x-7}{3x-1}\)
\(=\dfrac{5x-1-5x+7}{3x+2-3x+1}\)
\(=\dfrac{-1+7}{2+1}\)
\(=\dfrac{6}{3}\)
\(=2\)
Với \(\dfrac{5x-1}{3x+2}=2\)
\(\Rightarrow5x-1=2\left(3x+2\right)\)
\(\Rightarrow5x-1-2\left(3x+2\right)=0\)
\(\Rightarrow5x-1-6x-4=0\)
\(\Rightarrow-x-5=0\)
\(\Rightarrow x=-5\)
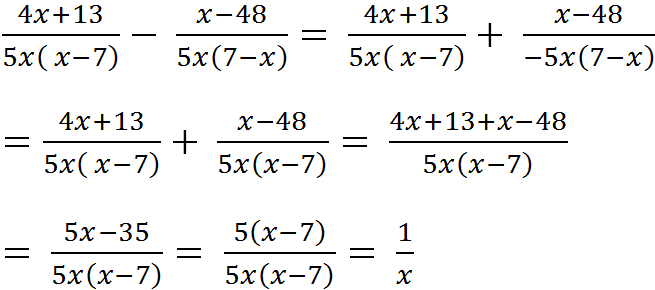



`a)1/[x-5x^2]-[25x-15]/[25x^2-1]`
`=[-(5x+1)-x(25x-15)]/[x(5x-1)(5x+1)]`
`=[-5x-1-25x^2+15x]/[x(5x-1)(5x+1)]`
`=[-25x^2+10x-1]/[x(5x-1)(5x+1)]`
`=[-(5x-1)^2]/[x(5x-1)(5x+1)]`
`=[1-5x]/[x(5x+1)]`
________________________________________________-
`b)(-1/[x^2-4x]+2/[16-x^2]-[-1]/[4x+16]):1/[4x]`
`=[-4(x+4)-8x+x(x-4)]/[4x(x-4)(x+4)].4x`
`=[-4x-16-8x+x^2-4x]/[(x-4)(x+4)]`
`=[x^2-16x-16]/[x^2-16]`