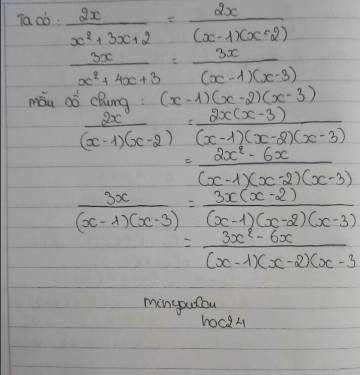K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H
19 tháng 9 2023
\(\dfrac{4}{x^2-3x+2}\) và \(\dfrac{1}{x^2-x}\)
\(\dfrac{4}{x^2-3x+2}=\dfrac{4}{\left(x-1\right)\left(x-2\right)}\)
\(\dfrac{1}{x^2-x}=\dfrac{1}{x\left(x-1\right)}\)
`MSC: x(x-1)(x-2)`
\(\dfrac{4}{\left(x-1\right)\left(x-2\right)}=\dfrac{4\cdot x}{x\left(x-1\right)\left(x-2\right)}=\dfrac{4x}{x\left(x-1\right)\left(x-2\right)}\)
\(\dfrac{1}{x\left(x-1\right)}=\dfrac{1\cdot\left(x-2\right)}{x\left(x-1\right)\left(x-2\right)}=\dfrac{x-2}{x\left(x-1\right)\left(x-2\right)}\)
NG
2
28 tháng 11 2021
\(\frac{x-1}{x^2+5x};\frac{x+1}{x^2-25}\)
ta có: \(\frac{x-1}{x^2+5x}=\frac{x-1}{x\left(x+5\right)}\)
\(\frac{x+1}{x^2-25}=\frac{x+1}{\left(x-5\right)\left(x+5\right)}\)
MTC: x(x-5).(x+5)
\(\frac{x-1}{x\left(x+5\right)_{\left(x-5\right)}}=\frac{\left(x-1\right)\left(x-5\right)}{x\left(x+5\right)\left(x-5\right)}=\frac{x^2-5x-x+5}{x\left(x+5\right)\left(x-5\right)}=\frac{x^2-4x+5}{x\left(x+5\right)\left(x-5\right)}\)
\(\frac{x+1}{\left(x-5\right)\left(x+5\right)_{\left(x\right)}}=\frac{x^2+x}{x\left(x-5\right)\left(x+5\right)}\)
Vậy ....
HN
0
7 tháng 12 2021
d: \(\dfrac{x}{x+3}=\dfrac{x^2-3x}{\left(x+3\right)\left(x-3\right)}\)
\(\dfrac{1}{3-x}=\dfrac{-1}{x-3}=\dfrac{-x-3}{\left(x-3\right)\left(x+3\right)}\)
\(\dfrac{1}{x^2-9}=\dfrac{1}{\left(x+3\right)\left(x-3\right)}\)
28 tháng 7 2023
a: 1/x^2y=1/x^2y
3/xy=3x/x^2y
b: \(\dfrac{x}{x^2+2xy+y^2}=\dfrac{x}{\left(x+y\right)^2}\)
\(\dfrac{2x}{x^2+xy}=\dfrac{2}{x+y}=\dfrac{2x+2y}{\left(x+y\right)^2}\)
7 tháng 12 2021
Bài 2:
a: \(\dfrac{1}{2x^3y}=\dfrac{6yz^3}{12x^3y^2z^3}\)
\(\dfrac{2}{3xy^2z^3}=\dfrac{2\cdot4x^2}{12x^3y^2z^3}=\dfrac{8x^2}{12x^3y^2z^3}\)
LY
3
23 tháng 11 2020
MTC : ( x - 1 )( x2 + x + 1 )
Ta có : \(\frac{4x^2-3x+5}{x^3-1}=\frac{4x^2-3x+5}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{2x}{x^2+x+1}=\frac{2x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\frac{2x^2-2x}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{6}{x-1}=\frac{6\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\frac{6x^2+6x+6}{\left(x-1\right)\left(x^2+x+1\right)}\)
25 tháng 11 2020
Hnay mới học thì hnay trả lời nhá :P
\(\frac{4x^2-3x+5}{x^3-1};\frac{2x}{x^2+x+1}\)
Ta có : \(x^3-1=\left(x-1\right)\left(x^2+x+1\right)\)
\(x^2+x+1=x^2+x+1\)
MTC : \(\left(x-1\right)\left(x^2+x+1\right)\)
\(\frac{4x^2-3x+5}{x^3-1}=\frac{4x^2-3x+5}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\frac{2x}{x^2+x+1}=\frac{2x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\frac{2x^2-2x}{\left(x-1\right)\left(x^2+x+1\right)}\)
18 tháng 11 2023
a: ĐKXĐ: \(x\notin\left\{4;-4\right\}\)
\(\dfrac{7}{4x+16}=\dfrac{7}{4\left(x+4\right)}=\dfrac{7\left(x-4\right)}{4\left(x+4\right)\left(x-4\right)}\)
\(\dfrac{11}{x^2-16}=\dfrac{11\cdot4}{4\left(x^2-16\right)}=\dfrac{44}{4\left(x-4\right)\left(x+4\right)}\)
b: \(\dfrac{6}{x\left(x+3\right)^2};\dfrac{x-3}{2x\left(x+3\right)^2}\)
ĐKXĐ: \(x\notin\left\{0;-3\right\}\)
\(\dfrac{6}{x\left(x+3\right)^2}=\dfrac{6\cdot2}{2x\left(x+3\right)^2}=\dfrac{12}{2x\left(x+3\right)^2}\)
\(\dfrac{x-3}{2x\left(x+3\right)^2}=\dfrac{x-3}{2x\left(x+3\right)^2}\)
c: \(\dfrac{-6}{1-x};\dfrac{3x}{x^2+x+1};\dfrac{x^2-3x+5}{x^3-1}\)
ĐKXĐ: \(x\ne1\)
\(-\dfrac{6}{1-x}=\dfrac{6}{x-1}=\dfrac{6\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{6x^2+6x+6}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\dfrac{3x}{x^2+x+1}=\dfrac{3x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{3x^2-3x}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\dfrac{x^2-3x+5}{x^3-1}=\dfrac{x^2-3x+5}{\left(x-1\right)\left(x^2+x+1\right)}\)
d: \(\dfrac{17}{5x};\dfrac{24}{x-2y};\dfrac{x-y}{8y^2-2x^2}\)
ĐKXĐ: \(x\ne0;x\ne\pm2y\)
\(\dfrac{17}{5x}=\dfrac{17\cdot2\left(x-2y\right)\left(x+2y\right)}{5x\cdot2\cdot\left(x-2y\right)\left(x+2y\right)}=\dfrac{34\left(x^2-4y^2\right)}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\dfrac{24}{x-2y}=\dfrac{24\cdot10x\left(x+2y\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\dfrac{240x\left(x+2y\right)}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\dfrac{x-y}{8y^2-2x^2}=\dfrac{-\left(x-y\right)}{2x^2-8y^2}=\dfrac{-\left(x-y\right)}{2\left(x-2y\right)\left(x+2y\right)}\)
\(=\dfrac{-5x\left(x-y\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\dfrac{-5x^2+5xy}{10x\left(x-2y\right)\left(x+2y\right)}\)