Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
ĐK: \(x\ge-2\)
Bất phương trình <=> \(\left(x^3+6x^2+12x+8\right)+2\sqrt{\left(x+2\right)^3}+1-9x^2-18x-9\ge0\)
<=> \(\left(\sqrt{\left(x+2\right)^3}+1\right)^2-\left(3x+3\right)^2\ge0\)
<=> \(\left(\sqrt{\left(x+2\right)^3}-3x-2\right)\left(\sqrt{\left(x+2\right)^3}+3x+4\right)\ge0\). Hướng dẫn đến đây nhé! Dạng này quen thuộc rồi, em chia trường hợp rồi làm nha!
1.
\(\Leftrightarrow3x=k\pi\Leftrightarrow x=\frac{k\pi}{3}\)
2.
\(\Leftrightarrow cos5x=0\Leftrightarrow5x=\frac{\pi}{2}+k\pi\Leftrightarrow x=\frac{\pi}{10}+\frac{k\pi}{5}\)
4.
\(cos3x+cosx+cos2x=0\)
\(\Leftrightarrow2cos2x.cosx+cos2x=0\)
\(\Leftrightarrow cos2x\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\cosx=-\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+\frac{k\pi}{2}\\x=\pm\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)
5.
\(sin6x+sin2x+sin4x=0\)
\(\Leftrightarrow2sin4x.cos2x+sin4x=0\)
\(\Leftrightarrow sin4x\left(2cos2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin4x=0\\cos2x=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{k\pi}{4}\\x=\pm\frac{\pi}{3}+k\pi\end{matrix}\right.\)
6. ĐKXĐ; ...
\(\Leftrightarrow tanx+tan2x=1-tanx.tan2x\)
\(\Leftrightarrow\frac{tanx+tan2x}{1-tanx.tan2x}=1\)
\(\Leftrightarrow tan3x=1\)
\(\Leftrightarrow x=\frac{\pi}{12}+\frac{k\pi}{3}\)
10. ĐKXĐ: \(x\ne\frac{\pi}{2}+k\pi\)
\(2cos2x+tanx=\frac{4}{5}\)
\(\Leftrightarrow4cos^2x-2+tanx=\frac{4}{5}\)
\(\Leftrightarrow\frac{4}{1+tan^2x}+tanx-\frac{14}{5}=0\)
Đặt \(tanx=t\)
\(\Rightarrow\frac{20}{1+t^2}+5t-14=0\)
\(\Leftrightarrow5t^3-14t^2+5t+6=0\)
\(\Leftrightarrow\left(t-2\right)\left(5t^2-4t-3\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}t=2\\t=\frac{2+\sqrt{19}}{5}\\t=\frac{2-\sqrt{19}}{5}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=2=tana\\tanx=\frac{2+\sqrt{19}}{5}=tanb\\tanx=\frac{2-\sqrt{19}}{5}=tanc\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=a+k\pi\\x=b+k\pi\\x=c+k\pi\end{matrix}\right.\)
9.
\(\Leftrightarrow cos2x-3cosx=2\left(cosx+1\right)\)
\(\Leftrightarrow2cos^2x-1-3cosx=2cosx+2\)
\(\Leftrightarrow2cos^2x-5cosx-3=0\)
\(\Rightarrow\left[{}\begin{matrix}cosx=3\left(l\right)\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Rightarrow x=\pm\frac{2\pi}{3}+k2\pi\)
bình phương 2 vế ra bạn ak
ta sẽ đc 64x4+48x3-8x2-6x=0
=) x=0
còn lại là bậc 3 phân tích ra đc : (8x2-1).(4x+3)=0
phần còn lại tự giải nhé
1d.
Đề ko rõ
1e.
\(\Leftrightarrow\left(4cos^3x-3cosx\right)^2.cos2x-cos^2x=0\)
\(\Leftrightarrow cos^2x\left(4cos^2x-3\right)^2.cos2x-cos^2x=0\)
\(\Leftrightarrow cos^2x\left(2cos2x-1\right)^2cos2x-cos^2x=0\)
\(\Leftrightarrow cos^2x\left[\left(2cos2x-1\right)^2.cos2x-1\right]=0\)
\(\Leftrightarrow cos^2x\left(4cos^32x-4cos^22x+cos2x-1\right)=0\)
\(\Leftrightarrow cos^2x\left(cos2x-1\right)\left(4cos^22x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cos2x=1\end{matrix}\right.\) \(\Leftrightarrow...\)
2b.
Đề thiếu
2c.
Nhận thấy \(cos2x=0\) ko phải nghiệm, chia 2 vế cho \(cos^32x\)
\(\frac{8sin^22x}{cos^22x}=\frac{\sqrt{3}sin2x}{cos2x}.\frac{1}{cos^22x}+\frac{1}{cos^22x}\)
\(\Leftrightarrow8tan^22x=\sqrt{3}tan2x\left(1+tan^22x\right)+1+tan^22x\)
\(\Leftrightarrow\sqrt{3}tan^32x-7tan^22x+\sqrt{3}tan2x+1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=\frac{1}{\sqrt{3}}\\tanx=\sqrt{3}-2\\tanx=\sqrt{3}+2\end{matrix}\right.\)
\(\Leftrightarrow...\)
Do quá làm biếng dùng Hoocne tách nhân tử nên chúng ta sẽ sử dụng L'Hopital:
\(\lim\limits_{x\rightarrow1}\frac{4x^6-5x^5+x}{x^2-2x+1}=\lim\limits_{x\rightarrow1}\frac{24x^5-25x^4+1}{2x-2}=\lim\limits_{x\rightarrow1}\frac{120x^4-100x^3}{2}=\frac{120-100}{2}=10\)
\(\lim\limits_{x\rightarrow-3}\frac{x^4-6x^2-27}{x^3+3x^2+x+3}=\lim\limits_{x\rightarrow-3}\frac{4x^3-12x}{3x^2+6x+1}=\frac{-36}{5}\)
\(\lim\limits_{x\rightarrow-2}\frac{2x^3+x^2+12}{-x^2-6x-8}=\lim\limits_{x\rightarrow-2}\frac{6x^2+2x}{-2x-6}=-10\)
\(\lim\limits_{x\rightarrow-2}\frac{-2x^3+x-14}{-2x^3-x^2-12}=\lim\limits_{x\rightarrow-2}\frac{-6x^2+1}{-6x^2-2x}=\frac{23}{20}\)
Con cuối ko phải tích phân dạng vô định \(\frac{0}{0}\) bạn cứ thế thẳng -2 vào là được
Chọn D.
Sử dụng công thức đạo hàm của hàm hợp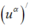
với u = 2x3 – 6x + 1
y' = 2(2x3 – 3x2 + 6x + 1)(2x3 – 3x2 + 6x + 1)’ = 2(2x3 – 3x2 + 6x + 1)(6x2 – 6x + 6).