Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\Leftrightarrow2sin^3x+1-sin^2x-1=0\)
\(\Leftrightarrow sin^2x\left(2sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\sinx=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)

ĐK: \(x\ne k\pi\)
\(\dfrac{1+sin2x+cos2x}{1+cot^2x}=sinx.\left(sin2x+2sin^2x\right)\)
\(\Leftrightarrow\dfrac{1+sin2x+cos2x}{\dfrac{cos^2x+sin^2x}{sin^2x}}=sinx.\left(2sinx.cosx+2sin^2x\right)\)
\(\Leftrightarrow\dfrac{1+sin2x+cos2x}{\dfrac{1}{sin^2x}}=2sin^2x.\left(cosx+sinx\right)\)
\(\Leftrightarrow1+sin2x+cos2x=2cosx+2sinx\)
\(\Leftrightarrow1+2sinx.cosx+2cos^2x-1=2cosx+2sinx\)
\(\Leftrightarrow\left(cosx-1\right).\left(sinx+cosx\right)=0\)
\(\Leftrightarrow\left(cosx-1\right).sin\left(x+\dfrac{\pi}{4}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\sin\left(x+\dfrac{\pi}{4}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x+\dfrac{\pi}{4}=k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{\pi}{4}+k\pi\end{matrix}\right.\)

Đáp án D
Dùng công thức cos a.cos b+ sin a. sin b= cos (a-b) để biến đổi phương trình không chứa
α
về dạng giống phương trình có chứa
α
Ta có

Đáp án D
Ta có
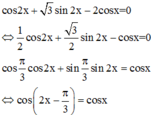
Do đó để phương trình ![]() tương đương với phương trình
tương đương với phương trình
![]()

\(\Leftrightarrow2\left(1-cos^22x\right)=2+\left(1-2sin^2x\right)\)
\(\Leftrightarrow2-2cos^22x=2+cos2x\)
\(\Leftrightarrow2cos^22x+cos2x=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\cos2x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{2}+k\pi\\2x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)


Pt \(\Leftrightarrow\left(2sinx-1\right)\left(2sin2x-1\right)=3-4\left(1-sin^2x\right)\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2sinx+1=-1+4sin^2x\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-\left(4sin^2x+2sinx-2\right)=0\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2\left(2sinx-1\right)\left(sinx+1\right)=0\)
\(\Leftrightarrow2\left(2sinx-1\right)\left(sin2x-sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\dfrac{1}{2}\left(1\right)\\sin2x=sinx+1\left(2\right)\end{matrix}\right.\)
Từ (1) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\),\(k\in Z\)
Từ (2)\(\Leftrightarrow2sinx.cosx-sinx-1=0\)
(Cái này tạm thời nghĩ ko ra,tối làm :)
\(sin2x=sinx+1\)
\(\Rightarrow\left\{{}\begin{matrix}sin2x\ge0\\sin^22x=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\4sin^2x.cos^2x=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\4sin^2x\left(1-sin^2x\right)=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\\left(sinx+1\right)\left(4sin^2x-4sin^3x-sinx-1\right)=0\end{matrix}\right.\)
Bấm máy thấy pt \(-4sin^3x+4sin^2x-sinx-1=0\) có một nghiệm \(sinx< 0\) không thỏa mãn \(sin2x\ge0\)
(Hoặc thử sd phương pháp cardano xem, chắc sẽ tìm được cụ thể nghiệm)
\(\Rightarrow sinx=-1\Leftrightarrow x=-\dfrac{\pi}{2}+k2\pi\) (\(k\in Z\))
Vậy...
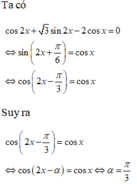
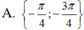
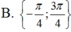

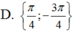

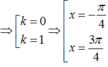
TH1: Xét cox = 0 ( có p là nghiệm ko)
TH2: Xét \(\cos x\ne0\). Ta chia cả hai vế \(\cos^2x\)
Pt trở thành \(2\tan^2x-4\tan x+4-1\left(1+\tan^2x\right)=0\)
\(\Leftrightarrow\tan^2x-4\tan x+3=0\)
Đặt \(\tan x=t\). Giải pt nữa là xg ạ
\(2sin^2x-4sinx.cosx+4cos^2x=1\)
\(\Leftrightarrow2\left(sin^2x+cos^2x\right)-4sinx.cosx+2cos^2x-1=0\)
\(\Leftrightarrow2-2sin2x+cos2x=0\)
\(\Leftrightarrow2sin2x-cos2x=2\)
\(\Leftrightarrow\sqrt{5}\left(\dfrac{2}{\sqrt{5}}sin2x-\dfrac{1}{\sqrt{5}}cos2x\right)=2\)
\(\Leftrightarrow sin\left(2x-arccos\dfrac{2}{\sqrt{5}}\right)=\dfrac{2}{\sqrt{5}}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-arccos\dfrac{2}{\sqrt{5}}=arcsin\dfrac{2}{\sqrt{5}}+k2\pi\\2x-arccos\dfrac{2}{\sqrt{5}}=\pi-arcsin\dfrac{2}{\sqrt{5}}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}arccos\dfrac{2}{\sqrt{5}}+\dfrac{1}{2}arcsin\dfrac{2}{\sqrt{5}}+k\pi\\x=\dfrac{\pi}{2}+\dfrac{1}{2}arccos\dfrac{2}{\sqrt{5}}-\dfrac{1}{2}arcsin\dfrac{2}{\sqrt{5}}+k\pi\end{matrix}\right.\)