K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PL
2
T
25 tháng 8 2023
\(3x\cdot\left(x-y\right)^2-6\cdot\left(y-x\right)\)
\(=3x\left(x-y\right)^2+6\left(x-y\right)\)
\(=\left(x-y\right)\left[3x\left(x-y\right)+6\right]\)
\(=\left(x-y\right)\left(3x^2-3xy+6\right)\)
KV
25 tháng 5 2023
x⁸ + x⁴ + 1
= x⁸ + 2x⁴ + 1 - x⁴
= (x⁴ + 1)² - x⁴
= (x⁴ + 1)² - (x²)²
= (x⁴ + 1 + x²)(x⁴ + 1 - x²)
= (x⁴ + x² + 1)(x⁴ - x² + 1)
H
24 tháng 8 2023
\(\left(x-y\right)^3-\left(x+y\right)^3\\ =\left(x-y-x-y\right)\left(\left(x-y\right)^2+\left(x-y\right)\left(x+y\right)+\left(x+y\right)^2\right)\\ =-2y\left(x^2-2xy+y^2+x^2-y^2+x^2+2xy+y^2\right)\\ =-2y\left(3x^2+y^2\right)\)
H
24 tháng 8 2023
\(\left(x-y\right)^3+\left(x+y\right)^3\\ =\left(x-y+x+y\right)\left(\left(x-y\right)^2-\left(x-y\right)\left(x+y\right)+\left(x+y\right)^2\right)\\ =2x\left(x^2-2xy+y^2-\left(x^2-y^2\right)+x^2+2xy+y^2\right)\\ =2x\left(x^2-2xy+y^2-x^2+y^2+x^2+2xy+y^2\right)\\ =2x\left(x^2+3y^2\right)\)
24 tháng 8 2023
=(x-y-2y)[(x-y)^2+2y(x-y)+4y^2]
=(x-3y)(x^2-2xy+y^2+2xy-2y^2+4y^2)
=(x-3y)(x^2+3y^2)
H9
HT.Phong (9A5)
CTVHS
24 tháng 8 2023
\(\left(x-y\right)^3-8y^3\)
\(=\left(x-y\right)^3-\left(2y\right)^3\)
\(=\left[\left(x-y\right)-2y\right]\left[\left(x-y\right)^2+2y\left(x-y\right)+\left(2y\right)^2\right]\)
\(=\left(x-y-2y\right)\left(x^2-2xy+y^2+2xy-2y^2+4y^2\right)\)
\(=\left(x-3y\right)\left(x^2+3y^2\right)\)
DG
4
DG
13 tháng 8 2016
(x^2+1)^2 - 4x(1-x^2)
=(x^2-1)^2 + 4x^2 + 4x(x^2-1)
(=(x^2-1+2x)^2
=((x-1)^2)^2
=(x-1)^4
16 tháng 8 2021
casio fx 570 thì ấn mode => 5 => 3 sau điền hệ số a;b;c
casio fx 580 thì ấn mode => 9 => 2 => 2 => điền hệ số a;b;c
16 tháng 8 2021
có cả cách này à =)))
menu setup -> 9 -> 2 - > 2 (pt cần phân tích) -> nhập hệ số của pt vào từng biến thích hợp -> ''=''
VD : \(A=x^2+4x-5\)có nghiệm \(x_1=1;x_2=-5\)
vậy đa thức cần phân tích là : \(\left(x-1\right)\left(x+5\right)=x^2+5x-x-5\)
Vậy \(A=x^2+4x-5=x^2+5x-x-5=\left(x-1\right)\left(x+5\right)\)
tương tự nhé
31 tháng 7 2021
19) Ta có: \(-x^2-4x-4\)
\(=-\left(x^2+4x+4\right)\)
\(=-\left(x+2\right)^2\)
20) Ta có: \(-4x^2-12x-9\)
\(=-\left(4x^2+12x+9\right)\)
\(=-\left(2x+3\right)^2\)
21) Ta có: \(-4x^2-4x-1\)
\(=-\left(4x^2+4x+1\right)\)
\(=-\left(2x+1\right)^2\)
22) Ta có: \(-x^2+6x-9\)
\(=-\left(x^2-6x+9\right)\)
\(=-\left(x-3\right)^2\)
23) Ta có: \(-x^2+10x-25\)
\(=-\left(x^2-10x+25\right)\)
\(=-\left(x-5\right)^2\)
24) Ta có: \(-x^2+8x-16\)
\(=-\left(x^2-8x+16\right)\)
\(=-\left(x-4\right)^2\)
25) Ta có: \(-4x^2+12x-9\)
\(=-\left(4x^2-12x+9\right)\)
\(=-\left(2x-3\right)^2\)
26) Ta có: \(a^2-a+b-b^2\)
\(=\left(a-b\right)\left(a+b\right)-\left(a-b\right)\)
\(=\left(a-b\right)\left(a+b-1\right)\)
31 tháng 7 2021
13) Ta có: \(y^2-2xy+2x-y\)
\(=y\left(y-2x\right)-\left(y-2x\right)\)
\(=\left(y-2x\right)\left(y-1\right)\)
14) Ta có: \(x-2xy+4y-2\)
\(=x\left(1-2y\right)-2\left(1-2y\right)\)
\(=\left(1-2y\right)\left(x-2\right)\)
15) Ta có: \(x^2-2xy+x-2y\)
\(=x\left(x-2y\right)+\left(x-2y\right)\)
\(=\left(x-2y\right)\left(x+1\right)\)
16) Ta có: \(xy-z-y+xz\)
\(=x\left(y+z\right)-\left(y+z\right)\)
\(=\left(y+z\right)\left(x-1\right)\)
17) Ta có: \(2xy+3z-6y-xz\)
\(=\left(2xy-xz\right)+\left(3z-6y\right)\)
\(=x\left(2y-z\right)-3\left(2y-z\right)\)
\(=\left(2y-z\right)\left(x-3\right)\)
18) Ta có: \(2xy-2z+4y-xz\)
\(=\left(2xy+4y\right)+\left(xz+2z\right)\)
\(=2y\left(x+2\right)+z\left(x+2\right)\)
\(=\left(x+2\right)\left(2y+z\right)\)
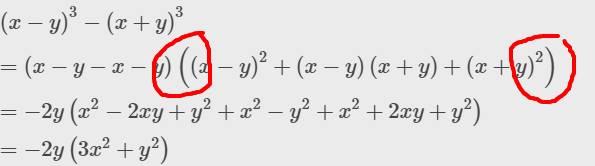
\(x^2+x-6=x^2-2x+3x-6=x\left(x-2\right)+3\left(x-2\right)=\left(x-2\right)\left(x+3\right)\)
x2 + x - 6
= x2 - 2x + 3x - 6
= x ( x - 2 ) + 3 ( x - 2 )
= ( x - 2 ) ( x + 3 )