Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Cách 1: Tách một hạng tử thành tổng hai hạng tử để xuất hiện nhân tử chung.
a) x2 – 3x + 2
= x2 – x – 2x + 2 (Tách –3x = – x – 2x)
= (x2 – x) – (2x – 2)
= x(x – 1) – 2(x – 1) (Có x – 1 là nhân tử chung)
= (x – 1)(x – 2)
Hoặc: x2 – 3x + 2
= x2 – 3x – 4 + 6 (Tách 2 = – 4 + 6)
= x2 – 4 – 3x + 6
= (x2 – 22) – 3(x – 2)
= (x – 2)(x + 2) – 3.(x – 2) (Xuất hiện nhân tử chung x – 2)
= (x – 2)(x + 2 – 3) = (x – 2)(x – 1)
b) x2 + x – 6
= x2 + 3x – 2x – 6 (Tách x = 3x – 2x)
= x(x + 3) – 2(x + 3) (có x + 3 là nhân tử chung)
= (x + 3)(x – 2)
c) x2 + 5x + 6 (Tách 5x = 2x + 3x)
= x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2) (Có x + 2 là nhân tử chung)
= (x + 2)(x + 3)
Cách 2: Đưa về hằng đẳng thức (1) hoặc (2)
a) x2 – 3x + 2
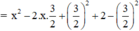
(Vì có x2 và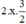 
nên ta thêm bớt 
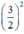để xuất hiện HĐT)
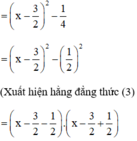
= (x – 2)(x – 1)
b) x2 + x - 6
= (x – 2)(x + 3).
c) x2 + 5x + 6
= (x + 2)(x + 3).
`@` `\text {Ans}`
`\downarrow`
`a)`
`6x^2 - 3xy`
`= 3x(2x - y)`
`b)`
Bạn ghi lại đầy đủ thông tin
`c)`
`x^2 - 5x + 6`
`= x^2 - 2x - 3x +6`
`= (x^2 - 2x) - (3x - 6)`
`= x(x - 2) - 3(x - 2)`
`= (x - 3)(x - 2)`
a: =3x*2x-3x*y
=3x(2x-y)
c: =x^2-2x-3x+6
=x(x-2)-3(x-2)
=(x-2)(x-3)
b: Bạn ghi đầy đủ đề nha bạn
a) (x - 2)(x - 3). b) 3(x - 2)(x + 5).
c) (x - 2)(3x + 1). d) (x-2y)(x - 5y).
e) (x + l)(x + 2)(x - 3). g) (x-1)(x + 3)( x 2 + 3).
h) (x + y - 3)(x - y + 1).
a: \(x^2-6x+5=\left(x-5\right)\left(x-1\right)\)
b: \(x^2-x-12=\left(x-4\right)\left(x+3\right)\)
c: \(x^2+8x+15=\left(x+5\right)\left(x+3\right)\)
d: \(2x^2-5x-12=\left(x-4\right)\left(2x+3\right)\)
e: \(x^2-13x+36=\left(x-9\right)\left(x-4\right)\)
-Đặt \(t=\left(x^2-x+1\right)\)
\(\left(x^2-x+1\right)^2-5x\left(x^2-x+1\right)+4x^2\)
\(=t^2-5xt+4x^2\)
\(=t^2-4xt-xt+4x^2\)
\(=t\left(t-4x\right)-x\left(t-4x\right)\)
\(=\left(t-4x\right)\left(t-x\right)\)
\(=\left(x^2-x+1-4x\right)\left(x^2-x+1-x\right)\)
\(=\left(x^2-5x+1\right)\left(x^2-2x +1\right)\)
\(=\left(x^2-5x+1\right)\left(x-1\right)^2\)
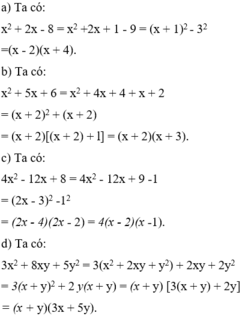
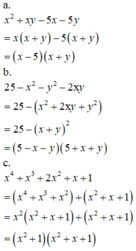
Giải:
a) \(x^2+5x=6\)
\(\Leftrightarrow x^2+5x-6=0\)
\(\Leftrightarrow x^2+6x-x-6=0\)
\(\Leftrightarrow\left(x^2+6x\right)-\left(x+6\right)=0\)
\(\Leftrightarrow x\left(x+6\right)-\left(x+6\right)=0\)
\(\Leftrightarrow\left(x+6\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+6=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-6\\x=1\end{matrix}\right.\)
Vậy ...
b) \(x^2-2015x+2014=0\)
\(\Leftrightarrow x^2-2014x-x+2014=0\)
\(\Leftrightarrow\left(x^2-2014x\right)-\left(x-2014\right)=0\)
\(\Leftrightarrow x\left(x-2014\right)-\left(x-2014\right)=0\)
\(\Leftrightarrow\left(x-2014\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2014=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2014\\x=1\end{matrix}\right.\)
Vậy ...
Chúc bạn học tốt!
\(a,x^2+5x=6\)
\(\Rightarrow x^2+5x-6=0\)
\(\Rightarrow x^2+6x-x-6=0\)
\(\Rightarrow\left(x^2-6x\right)-\left(x+6\right)=0\)
\(\Rightarrow x\left(x+6\right)-\left(x+6\right)=0\)
\(\Rightarrow\left(x+6\right)\left(x-1\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x+6=0\\x-1=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=-6\\x=1\end{matrix}\right.\)
\(b,x^2+2015x+2014=0\)
\(\Rightarrow x^2+2015x+2015-1=0\)
\(\Rightarrow\left(x^2-1\right)+\left(2015x+2015\right)=0\)
\(\Rightarrow\left(x-1\right)\left(x+1\right)+2015\left(x+1\right)=0\)
\(\Rightarrow\left(x+1\right)\left(x-1+2015\right)=0\)
\(\Rightarrow\left(x+1\right)\left(x+2014\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x+1=0\\x+2014=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=-1\\x=-2014\end{matrix}\right.\)