Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Mấy này bạn quy đồng lên cùng mẫu xong khử mẫu rồi giải. Dễ mà.

a) \(6x-3=4x+5\)
\(\Leftrightarrow6x-4x=5+3\)
\(\Leftrightarrow2x=8\)
\(\Leftrightarrow x=4\)
Vậy nghiệm là \(x=4\)
b) \(\dfrac{2x+3}{x+1}-\dfrac{6}{x}=2\)
\(\Leftrightarrow\dfrac{x\left(2x+3\right)}{x\left(x+1\right)}-\dfrac{6\left(x+1\right)}{x\left(x+1\right)}=\dfrac{2x\left(x+1\right)}{x\left(x+1\right)}\)
\(\Leftrightarrow x\left(2x+3\right)-6\left(x+1\right)=2x\left(x+1\right)\)
\(\Leftrightarrow2x^2+3x-6x-6=2x^2+2x\)
\(\Leftrightarrow2x^2-2x^2+3x-6x-2x-6=0\)
\(\Leftrightarrow-5x=6\)
\(\Leftrightarrow x=-\dfrac{5}{6}\)
Vậy nghiệm là \(x=-\dfrac{5}{6}\)
c) TH1:\(3x-1\ge0\Leftrightarrow x\ge\dfrac{1}{3}\)
\(\Rightarrow\left|3x-1\right|=3x-1\)
Thay vào phương trình:
Được: \(3x-1=3x\)
\(\Leftrightarrow3x-3x=1\)
\(\Leftrightarrow0x=1\Rightarrow\)Vô nghiệm
TH2: \(3x-1< 0\Rightarrow x< \dfrac{1}{3}\)
\(\Rightarrow\left|3x-1\right|=-\left(3x-1\right)=1-3x\)
Thay vào phương trình:
Được: \(1-3x=3x\)
\(\Leftrightarrow1-3x-3x=0\)
\(\Leftrightarrow-6x=-1\)
\(\Leftrightarrow x=\dfrac{1}{6}\)(Nhận)
Vậy nghiệm là \(x=\dfrac{1}{6}\)
a) 6x - 3 = 4x +5
<=> 6x - 4x = 5 + 3
<=> 2x = 8
<=> x = 4
Vậy tập nghiệm phương trình có S= {4}
b) \(\dfrac{2x+3}{x+1}-\dfrac{6}{x}=2\)
<=> \(\dfrac{x\left(2x+3\right)}{x\left(x+1\right)}-\dfrac{6\left(x+1\right)}{x\left(x+1\right)}=2\)
<=> \(\dfrac{2x^2+3x-6x-6}{x\left(x+1\right)}=2\)
<=> \(2x^2-3x-6=2x^2+2x\)
<=>\(2x^2-2x^2-3x-2x=6\)
<=>\(-5x=6\)
<=> \(x=\dfrac{-6}{5}\)
Vậy tập nghiệm của phương trình S={\(\dfrac{-6}{5}\)}
c) |\(3x-1\)|=\(3x\)
+ \(3x-1>=0\) <=> x>=\(\dfrac{1}{3}\)
=> \(3x-1=3x\)
<=> \(0=1\) ( Vô nghiệm)
+ \(3x-1< 0\) <=> \(x< \dfrac{1}{3}\)
=> \(3x-1=-3x\)
<=> \(6x=1\)
<=> \(x=\dfrac{1}{6}\) ( TM)
Vậy tập nghiệm của phương trình S={\(\dfrac{1}{6}\)}

Câu 1:
a) \(x-\dfrac{5x+2}{6}=\dfrac{7-3x}{4}\)
\(\Leftrightarrow\dfrac{12x-2\left(5x+2\right)}{12}=\dfrac{3\left(7-3x\right)}{12}\)
\(\Leftrightarrow12x-10x-4=21-9x\)
\(\Leftrightarrow11x=25\)
\(\Leftrightarrow x=\dfrac{25}{11}\)
b) \(\left(3x-1\right)\left(x-3\right)\left(7-2x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\Leftrightarrow x=\dfrac{1}{3}\\x-3=0\Leftrightarrow x=3\\7-2x=0\Leftrightarrow x=3,5\end{matrix}\right.\)
c) \(\left|3x\right|=4x+8\) (1)
Ta có: \(\left|3x\right|=3x\Leftrightarrow3x\ge0\Leftrightarrow x\ge0\)
\(\left|3x\right|=-3x\Leftrightarrow3x< 0\Leftrightarrow x< 0\)
Với \(x\ge0\), phương trình (1) có dạng:
\(3x=4x+8\Leftrightarrow-x=8\Leftrightarrow x=-8\)
(không thoả mãn điều kiện) \(\rightarrow\) loại
Với \(x< 0\), phương trình (1) có dạng:
\(-3x=4x+8\Leftrightarrow-7x=8\Leftrightarrow x=-\dfrac{8}{7}\)
(thoả mãn điều kiện) \(\rightarrow\) nhận
Vậy phương trình đã cho có 1 nghiệm \(x=-\dfrac{8}{7}\)
Câu 2:
\(2x\left(6x-1\right)\ge\left(3x-2\right)\left(4x+3\right)\)
\(\Leftrightarrow12x^2-2x\ge12x^2+9x-8x-6\)
\(\Leftrightarrow-3x\ge-6\)
\(\Leftrightarrow x\le2\)
Vậy bất phương trình đã cho có nghiệm \(x\le2\)

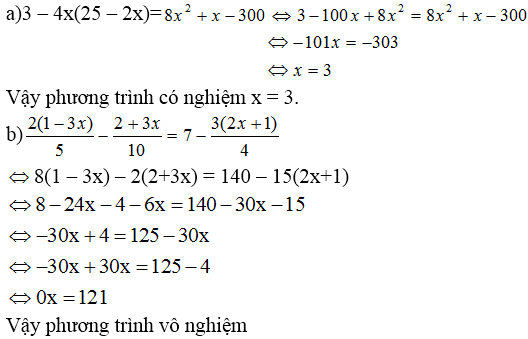




a)\(6x-3=4x+5\)
\(\Rightarrow6x-3-4x-5=0\)
\(\Rightarrow2x-8=0\)
\(\Rightarrow x=4\)
Vậy x=4
b)\(\frac{2x+3}{x+1}-\frac{6}{x}=2\left(ĐKXĐ:x\ne-1;0\right)\)
\(\Rightarrow\frac{2x^2+3x}{x\left(x+1\right)}-\frac{6x+6}{x\left(x+1\right)}=2\)
\(\Rightarrow\frac{2x^2+3x-6x-6}{x\left(x+1\right)}=2\)
\(\Rightarrow2x^2-3x-6=2\left(x^2+x\right)\)
\(\Rightarrow2x^2-3x-6-2x^2-2x=0\)
\(\Rightarrow-5x-6=0\)
\(\Rightarrow x=-\frac{6}{5}\)
Vậy \(x=-\frac{6}{5}\)
c)\(\left|3x-1\right|=3x\left(1\right)\)
TH1:\(x\ge\frac{1}{3}\).PT(1) có dạng:3x-1=3x
0x=1
PT vô nghiệm
TH2:\(x< \frac{1}{3}\).PT(1) có dạng:1-3x=3x
\(\Rightarrow6x=1\)
\(\Rightarrow x=\frac{1}{6}\left(TM\right)\)
Vậy PT có nghiệm là \(\frac{1}{6}\)
a, \(6x-3=4x+5 \)
\(\Leftrightarrow6x-4x=5+3\)
\(\Leftrightarrow2x=8\)
\(\Leftrightarrow x=4\)
vậy no của pt là : x = 4
b, \(\frac{2x+3}{x+1}-\frac{6}{x}=2\)
ĐKXĐ : \(\hept{\begin{cases}x\ne-1\\x\ne0\end{cases}}\)
\(\Leftrightarrow\frac{2x^2+3x-6x-6}{x\left(x+1\right)}=2\)
\(\Leftrightarrow\frac{2x^2-3x-6}{x\left(x+1\right)}=2\)
\(\Leftrightarrow2x^2-3x-6=2x^2+2x\)
\(\Leftrightarrow-5x=6\)
\(\Leftrightarrow x=\frac{-6}{5}\)
vậy no của pt là x=-6/5
c, \(\left|3x-1\right|=3x\)
Với \(3x-1\ge0\)
\(\Rightarrow3x-1=3x\Leftrightarrow-1=0\)( vô lí )