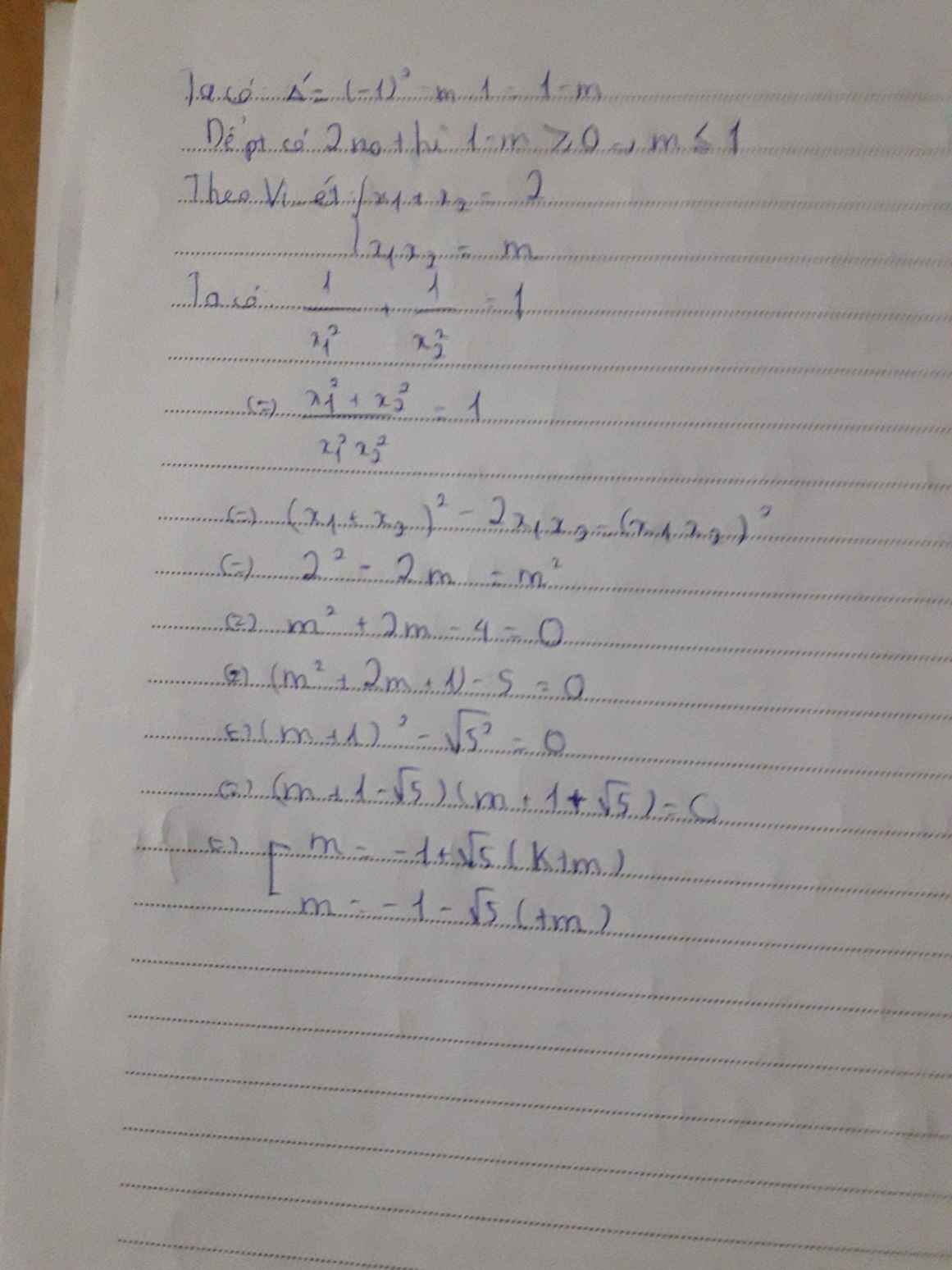Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

=>căn 2x1=x2-1
=>2x1=x2^2-2x2+1
=>x2^2-2(x1+x2)+1=0
=>x2^2-2(2m+1)+1=0
=>x2^2=4m+2-1=4m+1
=>\(x_2=\pm\sqrt{4m+1}\)
=>\(x_1=2m+1\pm\sqrt{4m+1}\)
x1*x2=m^2-m
=>m^2-m=4m+1\(\pm2m+1\)
=>m^2-5m-1=\(\pm2m+1\)
TH1: m^2-5m-1=2m+1
=>m^2-7m-2=0
=>\(m=\dfrac{7\pm\sqrt{57}}{2}\)
TH2: m^2-5m-1=-2m-1
=>m^2-3m=0
=>m=0; m=3

\(\Delta^'=\left(-1\right)^2-\left(m-1\right)=2-m\)
Để PT có nghiệm thì: \(m\le2\)
Khi đó theo hệ thức viet ta có: \(\hept{\begin{cases}x_1+x_2=2\\x_1x_2=m-1\end{cases}}\)
Ta có: \(x_1^4-x_1^3=x_2^4-x_2^3\)
\(\Leftrightarrow\left(x_1^4-x_2^4\right)-\left(x_1^3-x_2^3\right)=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left(x_1+x_2\right)\left(x_1^2+x_2^2\right)-\left(x_1-x_2\right)\left(x_1^2+x_1x_2+x_2^2\right)=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left[2\left(x_1^2+x_2^2\right)-x_1^2-x_1x_2-x_2^2\right]=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left(x_1^2-x_1x_2+x_2^2\right)=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left[\left(x_1+x_2\right)^2-3x_1x_2\right]=0\)
\(\Leftrightarrow\left(x_1-x_2\right)\left[4-3\left(m-1\right)\right]=0\)
Nếu \(x_1-x_2=0\Rightarrow x_1=x_2=1\Rightarrow m=1\left(tm\right)\)
Nếu \(4-3\left(m-1\right)=0\Rightarrow m=\frac{7}{3}\left(ktm\right)\)
Vậy m = 1

Phương trình có nghiệm \(\Leftrightarrow\Delta'\ge0\Leftrightarrow1-m\ge0\Leftrightarrow m\le1\)
Theo hệ thức Vi-ét: \(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=m\end{matrix}\right.\) (1)
Ta có: \(\dfrac{1}{x^2}+\dfrac{1}{x^2}=1\Leftrightarrow\dfrac{x^2_1+x^2_2}{x^2_1x^2_2}=1\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-2x_1x_2}{\left(x_1x_2\right)^2}=1\) (2)
Từ (1) và (2) \(\Rightarrow4-2m=m^2\Leftrightarrow m^2+2m-4=0\)
\(\Delta'=1+4=5\Rightarrow\sqrt{\Delta'}=\sqrt{5}\Rightarrow\left[{}\begin{matrix}m=-1+\sqrt{5}\left(\text{loại}\right)\\m=-1-\sqrt{5}\left(\text{nhận}\right)\end{matrix}\right.\)
Vậy \(m=-1-\sqrt{5}\)

=>căn 2x1=x2-1
=>2x1=x2^2-2x2+1
=>x2^2-2(x1+x2)+1=0
=>x2^2-2(2m+1)+1=0
=>x2^2=4m+2-1=4m+1
=>\(x_2=\pm\sqrt{4m+1}\)
=>\(x_1=2m+1\pm\sqrt{4m+1}\)
x1*x2=m^2-m
=>m^2-m=4m+1\(\pm2m+1\)
=>m^2-5m-1=\(\pm2m+1\)
TH1: m^2-5m-1=2m+1
=>m^2-7m-2=0
=>\(m=\dfrac{7\pm\sqrt{57}}{2}\)
TH2: m^2-5m-1=-2m-1
=>m^2-3m=0
=>m=0; m=3

\(x^2-mx-2=0\)
có \(\Delta=\left(-m\right)^2-4.\left(-2\right)=m^2+8>0\forall m\)
theo định lí vi - ét \(\hept{\begin{cases}x_1+x_2=m\\x_1.x_2=-2\end{cases}}\)
theo bài ra \(2x_1-x^2_1-x_2^2+2x_2\)
\(=2\left(x_1+x_2\right)-\left(x^2_1+x_2^2\right)\)
\(=2\left(x_1+x_2\right)-\left[\left(x_1+x_2\right)^2-2x_1.x_2\right]\)
\(=2m-\left[m^2-2.\left(-2\right)\right]\)
\(=2m-\left(m^2+4\right)\)
\(=2m-m^2-4\)
\(=-\left(m^2-2m+4\right)\)
\(=-\left[\left(m-1\right)^2+3\right]\)
Điều kiện để phương trình có 2 nghiệm phân biệt thì tự làm nha.
Áp dụng vi-et ta được
\(\hept{\begin{cases}x_1+x_2=m\\x_1x_2=-2\end{cases}}\)
\(\Rightarrow P=2\left(x_1+x_2\right)-\left[\left(x_1+x_2\right)^2-2x_1x_2\right]\)
\(=2m-\left(m^2+4\right)=-3-\left(m-1\right)^2\le-3\)

\(\Delta=\left(m-1\right)^2+8>0;\forall m\) nên pt luôn có 2 nghiệm pb với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m-1\\x_1x_2=-2\end{matrix}\right.\)
\(\left(1-\dfrac{2}{x_1+1}\right)^2+\left(1-\dfrac{2}{x_2+1}\right)^2=1\)
\(\Leftrightarrow\left(\dfrac{x_1-1}{x_1+1}\right)^2+\left(\dfrac{x_2-1}{x_2+1}\right)^2=1\)
\(\Leftrightarrow\left(\dfrac{x_1-1}{x_1+1}+\dfrac{x_2-1}{x_2+1}\right)^2-2\left(\dfrac{x_1-1}{x_1+1}\right)\left(\dfrac{x_2-1}{x_2+1}\right)=1\)
\(\Leftrightarrow\left(\dfrac{\left(x_1-1\right)\left(x_2+1\right)+\left(x_1+1\right)\left(x_2-1\right)}{\left(x_1+1\right)\left(x_2+1\right)}\right)^2-2\left(\dfrac{x_1x_2-\left(x_1+x_2\right)+1}{x_1x_2+x_1+x_2+1}\right)=1\)
\(\Leftrightarrow\left(\dfrac{2x_1x_2-2}{x_1x_2+x_1+x_2+1}\right)^2-2\left(\dfrac{x_1x_2-\left(x_1+x_2\right)+1}{x_1x_2+x_1+x_2+1}\right)=1\)
\(\Leftrightarrow\left(\dfrac{-6}{m-2}\right)^2+2\left(\dfrac{m}{m-2}\right)=1\)
\(\Leftrightarrow36\left(\dfrac{1}{m-2}\right)^2+4\left(\dfrac{1}{m-2}\right)+1=0\)
Pt trên vô nghiệm nên ko tồn tại m thỏa mãn yêu cầu
Tới đó đặt \(\dfrac{1}{m-2}=t\) là thành 1 pt bậc 2 bình thường, bấm máy thấy nó vô nghiệm là đủ kết luận rồi em

a) để phương trình có 2 nghiệm : \(\Leftrightarrow\left\{{}\begin{matrix}m-3\ne0\\\Delta'\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m-3\ne0\\\left(m+2\right)^2-\left(m-3\right)\left(m+1\right)\ge0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m\ne3\\6m+7\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne3\\m\ge\dfrac{7}{6}\end{matrix}\right.\)
thay \(x_1=2\) vào phương trình ta có :
\(4\left(m-3\right)-4\left(m+2\right)+m+1=0\Leftrightarrow m=19\)
áp dụng hệ thức vi ét ta có : \(x_1+x_2=\dfrac{2\left(m+2\right)}{m-3}=\dfrac{2\left(21\right)}{16}=\dfrac{21}{8}\)
\(\Rightarrow x_2=\dfrac{21}{8}-x_1=\dfrac{21}{8}-2=\dfrac{5}{8}\)
vậy ....................................................................................................
b) áp dụng hệ thức vi ét ta có : \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2\left(m+2\right)}{m-3}\\x_1x_2=\dfrac{m+1}{m-3}\end{matrix}\right.\)
ta có : \(\dfrac{1}{x_1}+\dfrac{1}{x_2}=10\Leftrightarrow\dfrac{x_1+x_2}{x_1x_2}=10\Leftrightarrow\dfrac{2\left(m+2\right)}{m-3}:\dfrac{m+1}{m-3}=10\)
\(\Leftrightarrow\dfrac{2m+4}{m+1}=10\Leftrightarrow2m+4=10m+10\Leftrightarrow m=\dfrac{-3}{4}\left(L\right)\)
vậy không có m thỏa mãn điều kiện bài toán .
câu 2) a) để phương trình có 2 nghiệm cùng dấu \(\Leftrightarrow\left\{{}\begin{matrix}m-2\ne0\\\Delta'\ge0\\p>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m-2\ne0\\\left(m+1\right)^2-\left(m-2\right)\left(m-1\right)\ge0\\\dfrac{m-1}{m-2}>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne2\\5m-1\ge0\\\left(m-1\right)\left(m-2\right)>0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m\ne2\\m\ge\dfrac{1}{5}\\\left[{}\begin{matrix}m>2\\m< 1\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow m>2\) vậy \(m>2\)
b) áp dụng hệ thức vi ét ta có : \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{-2\left(m+1\right)}{m-2}\\x_1x_2=\dfrac{m-1}{m-2}\end{matrix}\right.\)
ta có : \(x_1^3+x_2^3=64\Leftrightarrow\left(x_1+x_2\right)^3-3\left(x_1x_2\right)\left(x_1+x_2\right)=64\)
\(\left(\dfrac{2m+2}{2-m}\right)^3+6\left(\dfrac{m-1}{m-2}\right)\left(\dfrac{m+1}{m-2}\right)=64\)
\(\Leftrightarrow\dfrac{\left(-2m-2\right)^3}{\left(m-2\right)^3}+\dfrac{6\left(m-1\right)\left(m+1\right)\left(m-2\right)}{\left(m-2\right)^3}=64\)
\(\Leftrightarrow\dfrac{-8m^3-24m^2-24m-8+6m^2-12m^3-6m+12}{m^2-6m^2+12m-8}=64\)
\(\Leftrightarrow\dfrac{-20m^3-18m^2-30m+4}{m^3-6m^2+12m-8}=64\)
\(\Leftrightarrow84m^3-402m^2+798m-516=0\)
giải nốt nha .

a: Khi m = -4 thì:
\(x^2-5x+\left(-4\right)-2=0\)
\(\Leftrightarrow x^2-5x-6=0\)
\(\Delta=\left(-5\right)^2-5\cdot1\cdot\left(-6\right)=49\Rightarrow\sqrt{\Delta}=\sqrt{49}=7>0\)
Pt có 2 nghiệm phân biệt:
\(x_1=\dfrac{5+7}{2}=6;x_2=\dfrac{5-7}{2}=-1\)

1/
Phương trình \(x^2-2\left(k+3\right)x+2k-1=0\left(1\right)\)
Xét phương trình (1) có:
\(\Delta=4\left(k+3\right)^2-4\left(2k-1\right)\)
= \(4k^2+24k+36-8k+4\)
= \(4k^2+16k+40\)
= \(\left(2k+4\right)^2+24\)
Ta có: \(\left(2k+4\right)^2\ge0\) với mọi k
\(\Rightarrow\left(2k+4\right)^2+24>0\) với mọi k
\(\Rightarrow\Delta>0\) với mọi k
\(\Rightarrow\) Phương trình (1) có 2 nghiệm phân biệt với mọi k
Áp dụng hệ thức Vi-ét ta có:
\(\left\{{}\begin{matrix}x_1+x_2=2k+6\\x_1.x_2=2k-1\end{matrix}\right.\)
Theo đề bài ta có:
\(\dfrac{1}{x_1}+\dfrac{1}{x_2}+\dfrac{3}{x_1x_2}=2\)
\(\Leftrightarrow\dfrac{x_2+x_1+3}{x_1x_2}=\dfrac{2x_1x_2}{x_1x_2}\)
\(\Leftrightarrow x_1+x_2+3-2x_1x_2=0\)
\(\Leftrightarrow2k+6+3-2\left(2k-1\right)=0\)
\(\Leftrightarrow-2k=-11\)
\(\Leftrightarrow k=\dfrac{11}{2}\)
Vậy để phương trình (1) có 2 nghiệm \(x_1,x_2\) thỏa mãn \(\dfrac{1}{x_1}+\dfrac{1}{x_2}+\dfrac{3}{x_1x_2}=2\) thì \(k=\dfrac{11}{2}\)