TC
4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
HQ
Hà Quang Minh
Giáo viên
16 tháng 8 2023
\(a,5x\left(x^2-9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x^2=9\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\\ b,3\left(x+3\right)-x^2-3x=0\\ \Leftrightarrow3\left(x+3\right)-x\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left(3-x\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\\ c,x^2-9x-10=0\\ \Leftrightarrow x^2+x-10x-10=0\\ \Leftrightarrow x\left(x+1\right)-10\left(x+1\right)=0\\ \Leftrightarrow\left(x-10\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\\x=10\end{matrix}\right.\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
a, 5\(x\)(\(x^2\) - 9) = 0
\(\left[{}\begin{matrix}x=0\\x^2-9=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\)
Vậy \(x\) \(\in\) { -3; 0; 3}
b, 3.(\(x+3\)) - \(x^2\) - 3\(x\) = 0
3.(\(x+3\)) - \(x\).( \(x\) + 3) = 0
(\(x+3\))( 3 - \(x\)) = 0
\(\left[{}\begin{matrix}x=-3\\x=3\end{matrix}\right.\)
Vậy \(x\) \(\in\){ -3; 3}
c, \(x^2\) - 9\(x\) - 10 = 0
\(x^2\) + \(x\) - 10\(x\) - 10 = 0
\(x.\left(x+1\right)\) - 10.( \(x-1\)) = 0
(\(x+1\))(\(x-10\)) = 0
\(\left[{}\begin{matrix}x+1=0\\x-10=0\end{matrix}\right.\)
\(\left[{}\begin{matrix}x=-1\\x=10\end{matrix}\right.\)
Vậy \(x\) \(\in\){ -1; 10}
TC
1
15 tháng 12 2022
a.\(\left(x-1\right)^2+x\left(4-x\right)=11\)
\(\Leftrightarrow x^2-2x+1+4x-x^2=11\)
\(\Leftrightarrow2x=10\)
\(\Leftrightarrow x=5\)
b. \(x^2-9-2\left(x+3\right)=0\)
\(\Leftrightarrow x^2-9-2x-6=0\)
\(\Leftrightarrow x^2-2x-15=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=5\\x=-3\end{matrix}\right.\)
TC
Thầy Cao Đô
Giáo viên
VIP
15 tháng 12 2022
Em chú ý, dấu tương đương cuối cùng mình dùng ngoặc vuông (hoặc) chứ không dùng ngoặc nhọn (và) nhé.
QN
5
20 tháng 7 2017
\(\text{a , (x-3).(x^2+3x+9)+x(x+2).(2-x)=1 }\)
=(x3-33)+x(4-x2)=1
=x3-27+4x-x3=1
4x-27=1
4x=28
x=7
\(\text{b, (x+1)^3-(x-1)^3-6.(x-1)^2=-10}\)
=-0,5
N
2 tháng 11 2018
\(x^2-3x+2.\left(x-3\right)=0\)
\(x.\left(x-3\right)+2.\left(x-3\right)=0\)
\(\left(x-3\right).\left(x+2\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x=3\\x=-2\end{cases}}\)
\(x.\left(x-3\right)-3x+9=0\)
\(x.\left(x-3\right)-3.\left(x-3\right)=0\)
\(\left(x-3\right)^2=0=>x=3\)
2 tháng 11 2018
a,\(x^2-3x+2\left(x-3\right)=0.\)
\(\Leftrightarrow x^2-3x+2x-6=0\)
\(\Leftrightarrow x^2+x-6=0\)
\(\Leftrightarrow\left(x^2-2x\right)+\left(3x-6\right)=0\)
\(\Leftrightarrow x\left(x-2\right)+3\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-2=0\\x+3=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=2\\x=-3\end{cases}}\)
27 tháng 12 2015
b)(x+3)\(^2\)-x\(^2\)=9
(x+3-x)(x+3+x)=9
3(2x+3)=9
2x+3=3
2x=0
x=0
c)\(x^2+4x+4+x^2-6x+9-2x^2+2=9\)
-2x+15=9
-2x=-6
x=3
DN
27 tháng 12 2015
a) x(x-2) - 5x + 10 = 0
x(x - 2) - 5(x - 2)=0
(x - 2)(x - 5)=0
=> x=2 ; x=5
TT
2
10 tháng 8 2018
a) ta có : \(\left(x-1\right)^3+3x\left(x-4\right)+1=0\)
\(\Leftrightarrow x^3-3x^2+3x-1+3x^2-12x+1=0\)
\(\Leftrightarrow x^3-9x=0\Leftrightarrow x\left(x-3\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-3=0\\x+3=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\) vậy \(x=0;x=\pm3\)
b) \(x^2-25=6x-9\Leftrightarrow x^2-6x-16=0\)
\(\Leftrightarrow x^2+2x-8x-16=0\Leftrightarrow x\left(x+2\right)-8\left(x+2\right)=0\)
\(\Leftrightarrow\left(x-8\right)\left(x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}x-8=0\\x+2=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-2\end{matrix}\right.\)
vậy \(x=8;x=-2\)
10 tháng 8 2018
a )
\(\left(x-1\right)^3+3x\left(x-4\right)+1=0\)
\(\Leftrightarrow x^3-3x^2+3x-1+3x^2-12x+1=0\)
\(\Leftrightarrow x^3-9x=0\)
\(\Leftrightarrow x\left(x^2-9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2-9=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2=9\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\pm3\end{matrix}\right.\)
Vậy \(x\in\left\{0;\pm3\right\}\)
b )
\(x^2-25=6x-9\)
\(\Leftrightarrow x^2-6x=25-9\)
\(\Leftrightarrow x^2-6x=16\)
\(\Leftrightarrow x^2-6x-16=0\)
\(\Leftrightarrow x^2-2.x.3+9-25=0\)
\(\Leftrightarrow\left(x-3\right)^2=25\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=5\\x-3=-5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-2\end{matrix}\right.\)
Vậy ...
9 tháng 1 2022
\(a,\left(x-3\right)\left(x^2+3x+9\right)+x\left(x+2\right)\left(2-x\right)=0\\ \Rightarrow\left(x^3-27\right)+x\left(4-x^2\right)=0\\ \Rightarrow x^3-27+4x-x^3=0\\ \Rightarrow4x-27=0\\ \Rightarrow4x=27\\ \Rightarrow x=\dfrac{27}{4}\)
\(b,\left(x+1\right)^3-\left(x-1\right)^3-6\left(x-1\right)^2=-10\\ \Rightarrow\left(x^3+3x^2+3x+1\right)-\left(x^3-3x^2+3x-1\right)-6\left(x^2-2x+1\right)=-10\\ \Rightarrow x^3+3x^2+3x+1-x^3+3x^2-3x+1-6x^2+12x-6+10=0\)
\(\Rightarrow12x+6=0\\ \Rightarrow12x=-6\\ \Rightarrow x=-\dfrac{1}{2}\)
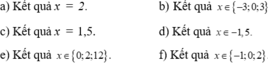
\(a,\\ \left(x+2\right)^2-x.\left(x-1\right)=10\\ \Leftrightarrow x^2+4x+4-x^2+x=10\\ \Leftrightarrow\left(x^2-x^2\right)+4x+x=10-4\\ \Leftrightarrow5x=6\\ \Leftrightarrow x=\dfrac{6}{5}\\ b,\\ x^3-6x^2+9x=0\\ \Leftrightarrow x.\left(x^2-6x+9\right)=0\\ \Leftrightarrow x.\left(x-3\right)^2=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)