Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Áp dụng BĐT Holder:
\(\left(\dfrac{a^2}{b+c}+\dfrac{b^2}{c+a}+\dfrac{c^2}{a+b}\right)^2\left[a^2\left(b+c\right)^2+b^2\left(c+a\right)^2+c^2\left(a+b\right)^2\right]\ge\left(a^2+b^2+c^2\right)^3\)
Mặt khác:
\(\left(a^2+b^2+c^2\right)^2\ge3\left(a^2b^2+b^2c^2+c^2a^2\right)\ge\dfrac{3}{2}\left(a^2b^2+b^2c^2+c^2a^2+abc\left(a+b+c\right)\right)\)
\(\Rightarrow\left(a^2+b^2+c^2\right)^2\ge\dfrac{3}{4}\left[a^2\left(b+c\right)^2+b^2\left(c+a\right)^2+c^2\left(a+b\right)^2\right]\)
\(\Rightarrow\left(\dfrac{a^2}{b+c}+\dfrac{b^2}{c+a}+\dfrac{c^2}{a+b}\right)^2\ge\dfrac{3}{4}\left(a^2+b^2+c^2\right)\)
\(\Rightarrow\dfrac{a^2}{b+c}+\dfrac{b^2}{c+a}+\dfrac{c^2}{a+b}\ge\dfrac{\sqrt{3}}{2}\sqrt{a^2+b^2+c^2}\)
\(\Rightarrow P\ge\dfrac{\sqrt{3}}{2}\sqrt{a^2+b^2+c^2}+\dfrac{4}{\sqrt{a^2+b^2+c^2+1}}\)
Đặt \(\sqrt{\dfrac{a^2+b^2+c^2}{3}}=x>0\)
\(\Rightarrow P\ge\dfrac{3x}{2}+\dfrac{4}{\sqrt{3x^2+1}}\)
Ta sẽ chứng minh \(P\ge\dfrac{7}{2}\)
Thật vậy, với \(x\ge\dfrac{7}{3}\Rightarrow P>\dfrac{3x}{2}\ge\dfrac{7}{2}\) (đúng)
Với \(0< x\le\dfrac{7}{3}\) ta cần chứng minh:
\(\dfrac{3x}{2}+\dfrac{4}{\sqrt{3x^2+1}}\ge\dfrac{7}{2}\Leftrightarrow\dfrac{4}{\sqrt{3x^2+1}}\ge\dfrac{7-3x}{2}\)
\(\Leftrightarrow64\ge\left(7-3x\right)^2\left(3x^2+1\right)\)
\(\Leftrightarrow3\left(x-1\right)^2\left(-9x^2+24x+5\right)\ge0\)
\(\Leftrightarrow\left(x-1\right)^2\left[3x\left(7-3x\right)+3x+5\right]\ge0\) (đúng)
Vậy \(P_{min}=\dfrac{7}{2}\) khi \(x=1\) hay \(a=b=c=1\)

Áp dụng bđt Schwarz ta có:
\(P=\dfrac{a^4}{2ab+3ac}+\dfrac{b^4}{2cb+3ab}+\dfrac{c^4}{2ac+3bc}\ge\dfrac{\left(a^2+b^2+c^2\right)^2}{5\left(ab+bc+ca\right)}\ge\dfrac{\left(a^2+b^2+c^2\right)^2}{5\left(a^2+b^2+c^2\right)}=\dfrac{1}{5}\).
Đẳng thức xảy ra khi và chỉ khi \(a=b=c=\dfrac{\sqrt{3}}{3}\).

Bạn xem lại đề, với a;b;c dương thì biểu thức P không tồn tại max nếu đề hoàn toàn đúng
Muốn P tồn tại max thì a;b;c cần không âm (nghĩa là có thể bằng 0)

\(\dfrac{a^5}{b^3+c^2}+\dfrac{b^3+c^2}{4}+\dfrac{a^4}{2}\ge3\sqrt[3]{\dfrac{a^9.\left(b^3+c^2\right)}{8\left(b^3+c^2\right)}}=\dfrac{3a^3}{2}\)
Tương tự và cộng lại:
\(\Rightarrow M-\dfrac{a^4+b^4+c^4}{2}+\dfrac{a^3+b^3+c^3}{4}+\dfrac{a^2+b^2+c^2}{4}\ge\dfrac{3}{2}\left(a^3+b^3+c^3\right)\)
\(\Rightarrow M\ge\dfrac{a^4+b^4+c^4}{2}+\dfrac{5}{4}\left(a^3+b^3+c^3\right)-\dfrac{3}{4}\)
Mặt khác ta có:
\(\dfrac{1}{2}\left(a^4+b^4+c^4\right)\ge\dfrac{1}{6}\left(a^2+b^2+c^2\right)^2=\dfrac{3}{2}\)
\(\left(a^3+a^3+1\right)+\left(b^3+b^3+1\right)+\left(c^3+c^3+1\right)\ge3\left(a^2+b^2+c^2\right)=9\)
\(\Rightarrow2\left(a^3+b^3+c^3\right)+3\ge9\Rightarrow a^3+b^3+c^3\ge3\)
\(\Rightarrow M\ge\dfrac{3}{2}+\dfrac{15}{4}-\dfrac{3}{4}=...\)

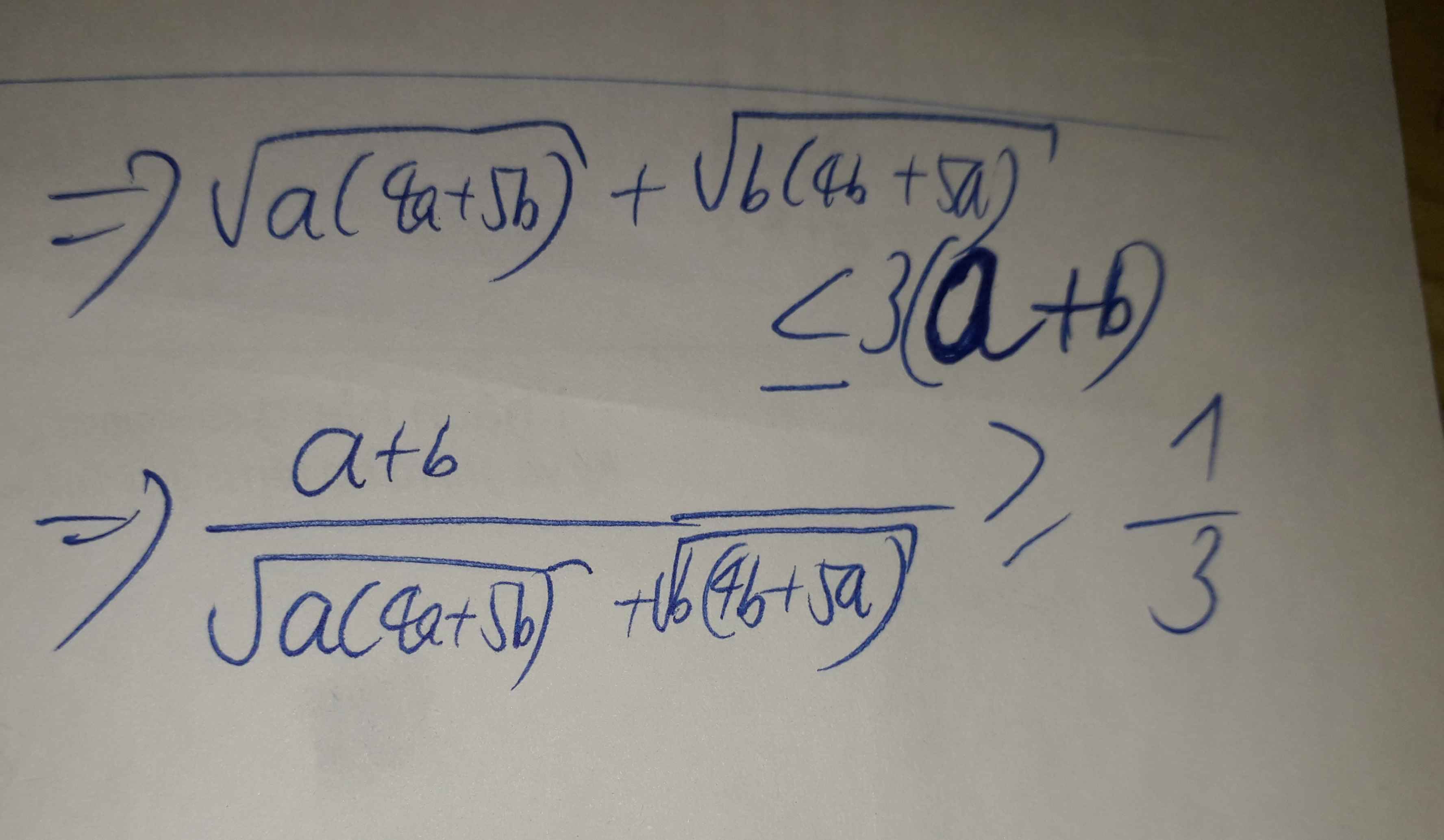

Theo BĐT Cauchy: \(\left\{{}\begin{matrix}\sqrt{ab}\le\dfrac{a}{4}+b\\\sqrt[3]{abc}\le\dfrac{1}{3}\left(\dfrac{a}{4}+b+4c\right)\end{matrix}\right.\)
\(\Rightarrow a+\sqrt{ab}+\sqrt[3]{abc}\le a+\dfrac{a}{4}+b+\dfrac{1}{3}\left(\dfrac{a}{4}+b+4c\right)=\dfrac{4}{3}\left(a+b+c\right)\)
\(\Rightarrow P\ge\dfrac{1346}{\dfrac{4}{3}\left(a+b+c\right)}-\dfrac{2019}{\sqrt{a+b+c}}=\dfrac{2019}{2\left(a+b+c\right)}-\dfrac{2019}{\sqrt{a+b+c}}\)
\(\Rightarrow\dfrac{2P}{2019}\ge\dfrac{1}{a+b+c}-\dfrac{2}{\sqrt{a+b+c}}=\left(\dfrac{1}{\sqrt{a+b+c}}\right)^2-2.\dfrac{1}{\sqrt{a+b+c}}+1-1\)
\(\Rightarrow\dfrac{2P}{2019}\ge\left(\dfrac{1}{\sqrt{a+b+c}}-1\right)^2-1\ge-1\)
\(\Rightarrow P\ge\dfrac{-2019}{2}\)
\(\Rightarrow P_{min}=\dfrac{-2019}{2}\)
Dấu "=" xảy ra khi:
\(\left\{{}\begin{matrix}\dfrac{a}{4}=b=4c\\\dfrac{1}{\sqrt{a+b+c}}-1=0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=\dfrac{16}{21}\\b=\dfrac{4}{21}\\c=\dfrac{1}{21}\end{matrix}\right.\)
bạn giỏi quá!
cảm ơn rất nhiều.