
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Câu 4:
D và F cùng nhìn AC dưới 1 góc vuông nên tứ giác ACDF nội tiếp
\(\Rightarrow\widehat{ADF}=\widehat{ACF}\) (cùng chắn AF)
Tương tự, ABDE nội tiếp \(\Rightarrow\widehat{ABE}=\widehat{ADE}\) (cùng chắn AE)
Lại có \(\widehat{ABE}=\widehat{ACF}\) (cùng phụ góc \(\widehat{A}\))
\(\Rightarrow\widehat{ADE}=\widehat{ADF}\) hay AD là phân giác góc \(\widehat{FDE}\)
./
Hoàn toàn tương tự, ta cũng có CF là phân giác \(\widehat{DFE}\Rightarrow\widehat{BFD}=\widehat{AFE}\)
Mà \(\widehat{AFE}=\widehat{BFK}\Rightarrow\widehat{BFK}=\widehat{BFD}\)
\(\Rightarrow\dfrac{BK}{BD}=\dfrac{FK}{FD}\) theo định lý phân giác
Đồng thời \(\dfrac{CK}{CD}=\dfrac{FK}{FD}\) (CF là phân giác ngoài góc \(\widehat{DFK}\))
\(\Rightarrow\dfrac{BK}{BD}=\dfrac{CK}{CD}\Rightarrow\dfrac{BK}{CK}=\dfrac{BD}{CD}\)
Qua B kẻ đường thẳng song song AC cắt AK và AD tại P và Q
Theo Talet: \(\dfrac{BK}{CK}=\dfrac{BP}{AC}\) đồng thời \(\dfrac{BD}{DC}=\dfrac{BQ}{AC}\)
\(\Rightarrow\dfrac{BP}{AC}=\dfrac{BQ}{AC}\Rightarrow BP=BQ\)
Mặt khác BP song song MF (cùng song song AC)
\(\Rightarrow\dfrac{MF}{BP}=\dfrac{AF}{AB}\) ; \(\dfrac{NF}{BQ}=\dfrac{AF}{AB}\) (Talet)
\(\Rightarrow\dfrac{MF}{BP}=\dfrac{NF}{BQ}\Rightarrow MF=NF\)

Bài 11:
a: Ta có: \(P=\dfrac{x^2-\sqrt{x}}{x+\sqrt{x}+1}\)
\(=\sqrt{x}\cdot\left(\sqrt{x}-1\right)\)
\(=x-\sqrt{x}\)
b: Để P=2 thì \(x-\sqrt{x}-2=0\)
hay x=4
Bài 10:
a: Ta có: \(A=\left(1+\dfrac{\sqrt{x}}{x+1}\right):\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{2\sqrt{x}}{x\sqrt{x}+\sqrt{x}-x-1}\right)\)
\(=\dfrac{x+\sqrt{x}+1}{x+1}:\dfrac{x-2\sqrt{x}+1}{\left(x+1\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x+\sqrt{x}+1}{x+1}\cdot\dfrac{x+1}{\sqrt{x}-1}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}-1}\)
b: Để A<0 thì \(\sqrt{x}-1< 0\)
hay x<1
Kết hợp ĐKXĐ, ta được: \(0\le x< 1\)
Để A=-1 thì \(x+\sqrt{x}+1=-\sqrt{x}+1\)
\(\Leftrightarrow x=0\)
c: Thay x=4 vào A, ta được:
\(A=\dfrac{4+2+1}{2-1}=7\)


Với m = 3 thì (d): y = 8x - 7
PTHĐGĐ của (P) và (d): \(x^2-8x+7=0\)
Có: \(a+b+c=1+\left(-8\right)+7=0\)
=> PT có 2 nghiệm phân biệt \(x_1=1;x_2=7\)
\(x_1=1\Rightarrow y_1=x_1^2=1^2=1\\ x_2=7\Rightarrow y_2=x_2^2=7^2=49\)
Tọa độ giao điểm của (P) và (d) là: \(\left(1;1\right);\left(7;49\right)\)
b)
PTHĐGĐ của (P) và (d) là:
\(x^2-2\left(m+1\right)x+3m-2=0\)
\(\Delta'=\left(m+1\right)^2-\left(3m-2\right)=m^2+2m+1-3m+2=m^2-m+3\\ =m^2-m+\dfrac{1}{4}+\dfrac{11}{4}=\left(m-\dfrac{1}{2}\right)^2+\dfrac{11}{4}>0\forall m\)
Theo vi ét: \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=3m-2\end{matrix}\right.\)
Theo đề: \(x_1^2+x_2^2=20\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=20\\ \Leftrightarrow\left(2m+2\right)^2-2\left(3m-2\right)=20\)
\(\Leftrightarrow4m^2+8m+4-6m+4=20\\ \Leftrightarrow4m^2+2m+8-20=0\\ \Leftrightarrow4m^2+2m-12=0\\ \Leftrightarrow2m^2+m-6=0\)
\(\Rightarrow\left\{{}\begin{matrix}m=-2\left(tm\right)\\m=\dfrac{3}{2}\left(tm\right)\end{matrix}\right.\)
Gọi tọa độ của \(\left(P\right),\left(d\right)\) là \(A\left(x_A;y_A\right),B\left(x_B;y_B\right)\)
\(a,m=3\)
\(\Rightarrow x^2=2\left(3+1\right)x-3.3+2\)
\(\Rightarrow x^2-8x+7=0\)
\(\Rightarrow\left\{{}\begin{matrix}x=7\\x=1\end{matrix}\right.\)
Thay \(x=7\) vào \(\left(P\right):y=x^2\Rightarrow y=7^2=49\)
Khi m = 3 thì đường thẳng \(\left(d\right):y=2\left(3+1\right)x-3.3+2=8x-7\)
Thay \(x=1\) vào \(\left(d\right):y=8x-7=8.1-7=1\)
Vậy \(A\left(7;49\right),B\left(1;1\right)\)
\(\Rightarrow y=\left(2m+2\right)x-3m+2\)
\(b,\) Vì \(\left(P\right)\) và \(\left(d\right)\) luôn cắt nhau tại 2 điểm pb A,B \(\forall m\) nên :
\(x^2=2\left(m+1\right)x-3m+2\Leftrightarrow x^2-2\left(m+1\right)x+3m-2\)
Theo Vi-ét, ta có : \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2m+2\\x_1x_2=\dfrac{c}{a}=3m-2\end{matrix}\right.\)
Ta có : \(x_1^2+x_2^2=20\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2\)
\(\Leftrightarrow\left(2m+2\right)^2-2\left(3m-2\right)=20\)
\(\Leftrightarrow4m^2+8m+4-6m+4-20=0\)
\(\Leftrightarrow4m^2+2m-12=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{3}{2}\\m=-2\end{matrix}\right.\)
Vậy \(m=\dfrac{3}{2},m=-2\) thì thỏa mãn đề bài.

a) \(A=\sqrt{1-x}+\sqrt{1+x}\)
\(\Rightarrow A^2=1-x+1+x+2\sqrt{\left(1-x\right)\left(1+x\right)}=2+2\sqrt{1-x^2}\)
Do \(-x^2\le0\Rightarrow1-x^2\le1\Rightarrow A^2=2+2\sqrt{1-x^2}\le2+2=4\)
\(\Rightarrow A\le2\)
\(maxA=2\Leftrightarrow x=0\)
Áp dụng bất đẳng thức: \(\sqrt{x}+\sqrt{y}\ge\sqrt{x+y}\)(với \(x,y\ge0\))
\(\Leftrightarrow\left(\sqrt{x}+\sqrt{y}\right)^2\ge x+y\)
\(\Leftrightarrow x+y+2\sqrt{xy}\ge x+y\Leftrightarrow2\sqrt{xy}\ge0\left(đúng\right)\)
\(A=\sqrt{1-x}+\sqrt{1+x}\ge\sqrt{1-x+1+x}=\sqrt{2}\)
\(maxA=\sqrt{2}\Leftrightarrow\)\(\left[{}\begin{matrix}1-x=0\\1+x=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)

5:
Th1: m=0
=>6x-27=0
=>x=27/6(loại)
TH2: m<>0
Δ=(6m-6)^2-4m(9m-27)
=36m^2-72m+36-36m^2+108m=36m+36
Để phương trình có hai nghiệm pb thì 36m+36>0
=>m>-1
x1+x2=x1x2
=>6(m-1)=9(m-3)
=>9m-27=6m-6
=>3m=21
=>m=7

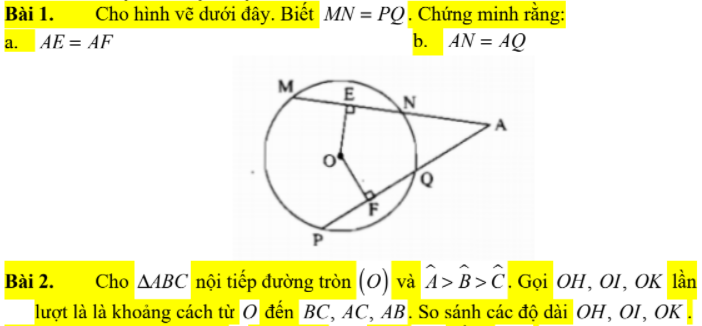
Bài 2:
Ta có: \(\widehat{A}>\widehat{B}>\widehat{C}\)
nên BC>AC>AB
hay OH<OI<OK






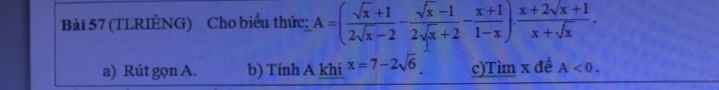



 Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!
Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!
Tứ giác AOKC nội tiếp (K và A cùng nhìn OC dưới góc vuông)
\(\Rightarrow\widehat{ACO}=\widehat{AKO}\) (cùng chắn AO) (1)
Mà \(\widehat{ACO}=\widehat{IAO}\) (cùng phụ \(\widehat{AOC}\)) (2)
\(\widehat{IAO}=\widehat{OIA}\) (\(OI=OA\) nên tam giác OIA cân tại O) (3)
(1);(2);(3) \(\Rightarrow\widehat{OIA}=\widehat{AKO}\)
Do \(\widehat{OIA}\) và \(\widehat{AKO}\) cùng chắn OA \(\Rightarrow OKIA\) nội tiếp
c. Theo cmt \(\Rightarrow\widehat{AIK}+\widehat{AOK}=180^0\)
AOKC nội tiếp (như đầu câu b đã nói) \(\Rightarrow\widehat{AOK}+\widehat{ACK}=180^0\)
\(\Rightarrow\widehat{AIK}=\widehat{ACK}\) (4)
Lại có tứ giác ACDH nội tiếp (D và H cùng nhìn AC dưới 1 góc vuông)
\(\Rightarrow\widehat{ACK}+\widehat{AHD}=180^0\) mà \(\widehat{AHD}+\widehat{MHD}=180^0\Rightarrow\widehat{ACK}=\widehat{MDH}\) (5)
(4);(5) \(\Rightarrow\widehat{AIK}=\widehat{MHD}\Rightarrow DH||IK\) (2 góc so le trong bằng nhau)
\(\Rightarrow\dfrac{DM}{KM}=\dfrac{HM}{IM}\) (định lý Talet)
Mặt khác \(CH||IB\) (cùng vuông góc AB)
\(\Rightarrow\dfrac{CM}{BM}=\dfrac{HM}{IM}\)
\(\Rightarrow\dfrac{DM}{KM}=\dfrac{CM}{BM}\Rightarrow DM.BM=KM.CM\)
tưởng anh Thịnh lên c3 rồi chứ