K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
OM
1
18 tháng 8 2023
\(C=\dfrac{-1}{5}+\left(\dfrac{1}{-5}\right)^2+\left(-\dfrac{1}{5}\right)^3+...+\left(-\dfrac{1}{5}\right)^{99}\)
=>\(5\cdot C=-1+\left(-\dfrac{1}{5}\right)+\left(-\dfrac{1}{5}\right)^2+...+\left(-\dfrac{1}{5}\right)^{98}\)
=>\(5\cdot C-C=\left(-1\right)-\left(-\dfrac{1}{5}\right)^{99}\)
=>\(4C=-1+\dfrac{1}{5^{99}}=\dfrac{-5^{99}+1}{5^{99}}\)
=>\(C=\dfrac{-5^{99}+1}{4\cdot5^{99}}\)
(x-3y)^2006+(y+4)^2008=0
=>x-3y=0 và y+4=0
=>x=3y và y=-4
=>x=3*(-4)=-12 và y=-4
KT
ÉT Ô ÉT
Câu 3: Tìm x biết:
|x + 1| + |x + 2| + |x + 2020| = 4x
Giúp mik với!!!
Mik hứa Tick cho… Pls
2
22 tháng 4 2023
TH1 : \(x< -2020\)
<=> | x + 1 | + | x + 2 | + | x + 2020 | = - ( x + 1 ) - ( x + 2 ) - ( x + 2020 ) = 4x
<=> -3x - 2023 = 4x <=> -7x = 2023 <=> x = -289
TH2 : \(-2020\le x< -2\)
<=> | x + 1 | + | x + 2 | + | x + 2020 | = - ( x + 1 ) - ( x + 2 ) + x + 2020 = 4x
<=> -x + 2017 = 4x
<=> -5x = -2017 <=> x = 2017/5 ( = 403,4 )
TH3 : \(-2\le x< -1\)
<=> | x + 1 | + | x + 2 | + | x + 2020 | = - ( x + 1 ) + x + 2 + x + 2020 = 4x
<=> x + 2021 = 4x <=> -3x = -2021 <=> x = 2021/3
TH4 : \(x>-1\)
<=> | x + 1 | + | x + 2 | + | x + 2020 | = x + 1 + x + 2 + x + 2020 = 4x
<=> 3x + 2023 = 4x
<=> -x = -2023 <=> x = 2023
Vậy...
22 tháng 4 2023
TH1: x ≥ 0
Khi đó \(\left|x+1\right|+\left|x+2\right|+\left|x+2020\right|=x+1+x+2+x+2020\)
\(=3x+2023=4x\)
Suy ra \(4x-3x=x=2023\) (thỏa mãn điều kiện)
TH2: x < 0
Khi đó 4x < 0 hay vế phải luôn là một số âm. Tuy nhiên vế trái luôn luôn có giá trị lớn hơn 0 nên luôn là 0 hoặc là một số dương, suy ra vô lí.
Tóm lại, x = 2023.
KO
1
T
8 tháng 9 2023
\(a,A=x^3+3x^2-4x-12\)
\(=x^2\left(x+3\right)-4\left(x+3\right)\)
\(=\left(x^2-4\right)\left(x+3\right)\)
\(=\left(x-2\right)\left(x+2\right)\left(x+3\right)\)
Thay \(x=2\) vào A, ta được:
\(A=\left(2-2\right)\left(2+2\right)\left(2+3\right)\)
\(=0\)
⇒ \(x=2\) là nghiệm của A
\(B=-2x^3+3x^2+4x+1\)
Thay \(x=2\) vào B, ta được:
\(B=-2\cdot2^3+3\cdot2^2+4\cdot2+1\)
\(=-16+12+8+1\)
\(=5\)
⇒ \(x=2\) không là nghiệm của B
\(b,A+B=x^3+3x^2-4x-12+\left(-2x^3\right)+3x^2+4x+1\)
\(=\left[x^3+\left(-2x^3\right)\right]+\left(3x^2+3x^2\right)+\left(-4x+4x\right)+\left(-12+1\right)\)
\(=-x^3+6x^2-11\)
\(A-B=x^3+3x^2-4x-12-\left(-2x^3+3x^2+4x+1\right)\)
\(=x^3+3x^2-4x-12+2x^3-3x^2-4x-1\)
\(=\left(x^3 +2x^3\right)+\left(3x^2-3x^2\right)+\left(-4x-4x\right)+\left(-12-1\right)\)
\(=3x^3-8x-13\)
#\(Toru \)
5 tháng 5 2022
\(a)P\left(x\right)=5x^5+3x-4x^4-2x^3+6+4x^2\)
\(P\left(x\right)=5x^5-4x^4-2x^3+4x^2+3x+6\)
\(Q\left(x\right)=2x^4-x+3x^2-2x^3+\dfrac{1}{4}-x^5\)
\(Q\left(x\right)=-x^5+2x^4-2x^3+3x^2-x+\dfrac{1}{4}\)
\(a)P\left(x\right)-Q\left(x\right)=\left(5x^5-4x^4-2x^3+4x^2+3x+6\right)+\left(-x^5+2x^4-2x^3+3x^2-x+\dfrac{1}{4}\right)\)
\(P\left(x\right)-Q\left(x\right)=5x^5-4x^4-2x^3+4x^2+3x+6+x^5-2x^4+2x^3-3x^2+x-\dfrac{1}{4}\)
\(P\left(x\right)-Q\left(x\right)=\left(5x^5+x^5\right)+\left(-4x^4-2x^4\right)+\left(-2x^3+2x^3\right)+\left(4x^2-3x^2\right)+\left(3x+x\right)+\left(6-\dfrac{1}{4}\right)\)
\(P\left(x\right)-Q\left(x\right)=6x^5-6x^4+x^2+4x+\dfrac{23}{4}\)
\(\text{c)Thay x=-1 vào biểu thức P(x),ta được:}\)
\(P\left(x\right)=5.\left(-1\right)^5-4.\left(-1\right)^4-2.\left(-1\right)^3+4.\left(-1\right)^2+3.\left(-1\right)+6\)
\(P\left(x\right)=\left(-5\right)-4-\left(-2\right)+4+\left(-3\right)+6\)
\(P\left(x\right)=\left(-9\right)-\left(-2\right)+4+\left(-3\right)+6\)
\(P\left(x\right)=\left(-7\right)+4+\left(-3\right)+6\)
\(P\left(x\right)=\left(-3\right)+\left(-3\right)+6\)
\(P\left(x\right)=\left(-6\right)+6=0\)
\(\text{Vậy giá trị của P(x) tại x=-1 là:0}\)
\(\text{Vậy =-1 là nghiệm của P(x)}\)
\(\text{Thay x=-1 vào biểu thức Q(x),ta được:}\)
\(Q\left(x\right)=\left(-1\right).5+2.\left(-1\right)^4-2.\left(-1\right)^3+3.\left(-1\right)^2-\left(-1\right)+\dfrac{1}{4}\)
\(Q\left(x\right)=\left(-5\right)+2-\left(-2\right)+3-\left(-1\right)+\dfrac{1}{4}\)
\(Q\left(x\right)=\left(-3\right)-\left(-2\right)+3-\left(-1\right)+\dfrac{1}{4}\)
\(Q\left(x\right)=\left(-5\right)+3-\left(-1\right)+\dfrac{1}{4}\)
\(Q\left(x\right)=\left(-2\right)-\left(-1\right)+\dfrac{1}{4}\)
\(Q\left(x\right)=\left(-3\right)+\dfrac{1}{4}=\dfrac{-13}{4}\)
\(\text{Vậy x=-1 không phải là nghiệm của Q(x)}\)
\(\text{d)Thay x=-1 vào biểu thức }P\left(x\right)-Q\left(x\right),\text{ta được:}\)
\(P\left(x\right)-Q\left(x\right)=6.\left(-1\right)^5-6.\left(-1\right)^4+\left(-1\right)^2+4.\left(-1\right)+\dfrac{23}{4}\)
\(P\left(x\right)-Q\left(x\right)=\left(-6\right)-6+1+\left(-4\right)+\dfrac{23}{4}\)
\(P\left(x\right)-Q\left(x\right)=\left(-12\right)+1+\left(-4\right)+\dfrac{23}{4}\)
\(P\left(x\right)-Q\left(x\right)=\left(-11\right)+\left(-4\right)+\dfrac{23}{4}\)
\(P\left(x\right)-Q\left(x\right)=\left(-15\right)+\dfrac{23}{4}=\dfrac{-37}{4}\)
\(\text{Vậy giá trị của P(x)-Q(x) tại x=-1 là:}\dfrac{-37}{4}\)
2
11 tháng 5 2022
Cho `F(x)=0`
`=>2x^2+5x+2=0`
`=>2x^2+4x+x+2=0`
`=>2x(x+2)+(x+2)=0`
`=>(x+2)(2x+1)=0`
`@TH1: x+2=0=>x=-2`
`@TH2: 2x+1=0=>x=[-1]/2`
Vậy nghiệm của `F(x)` là `x=-2` hoặc `x=[-1]/2`
11 tháng 5 2022
`2x^2 + 5x + 2`
`= 2x(x+2) + (x+2)`
`=(2x+1)(x+2) =0 `
`=> 2x + 1 = 0`
`x + 2 = 0`
`=> x = -1/2`
`x = -2`
16 tháng 3 2022
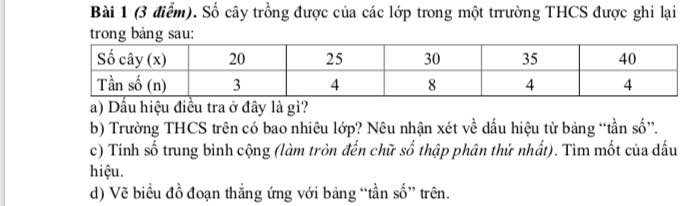
a. Dấu hiệu điều tra là số cây trồng được của lớp trong một trường THCS.
b. Có tất cả 23 lớp
NX: có 3 lớp trồng được ít nhất 20 cây
số 8 lớp trồng được nhiều nhất 30 cây
c. N = \(\dfrac{20.3+25.4+30.8+35.4+40.4}{23}\)
= \(\approx30,43\)
Mo = 30
13 tháng 1 2023
a: a vuông góc với AB
b vuông góc với AB
=>a//b
b: góc C1=75 độ
c: góc D1=góc C=75 độ
d: góc D2=180-75=105 độ
ÉT O ÉT
\(\left(1-x\right)^2=2003.\left(x-1\right)\)
\(\left(1-x\right)^2-2003\left(x-1\right)=0\)
\(\left(1-x\right)^2+2003\left(1-x\right)=0\)
\(\left(1-x\right)\left(1-x+2003\right)=0\)
\(\left(1-x\right)\left(2004-x\right)=0\)
\(TH1:1-x=0\)
\(x=1\)
\(TH2:2004-x=0\)
\(x=2004\)
vậy........