Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

A=−2x2−10y2+4xy+4x+4y+2016A=−2x2−10y2+4xy+4x+4y+2016
=−2.(x2+5y2−4xy−4x−4y)+2016=−2.(x2+5y2−4xy−4x−4y)+2016
=−2.(x2+4y2+4−4xy−4x+8y+y2−12y+36)+2.36+2016=−2.(x2+4y2+4−4xy−4x+8y+y2−12y+36)+2.36+2016
=−2.[(x−2y−2)2+(y−6)2]+2088=−2.[(x−2y−2)2+(y−6)2]+2088
Ta có: (x−2y−2)2+(y−6)2≥0(x−2y−2)2+(y−6)2≥0
⇒−2.[(x−2y−2)2+(y−6)2]≤0⇒−2.[(x−2y−2)2+(y−6)2]≤0
⇒−2.[(x−2y−2)2+(y−6)2]+2088≤2088⇒−2.[(x−2y−2)2+(y−6)2]+2088≤2088
⇒A≤2088⇒A≤2088
Vậy giá trị lớn nhất của A=2088A=2088 khi: \hept{x−2y−2=0y=6⇒\hept{x=2y+2y=6⇒\hept{x=14y=6\hept{x−2y−2=0y=6⇒\hept{x=2y+2y=6⇒\hept{x=14y=6
Thu gọn
\(A=-2\left(x^2+2xy+y^2\right)+4\left(x+y\right)-2-8y^2+2018\\ A=-2\left[\left(x+y\right)^2-2\left(x+y\right)+1\right]-8y^2+2018\\ A=-2\left(x+y-1\right)^2-8y^2+2018\le2018\\ A_{max}=2018\Leftrightarrow\left\{{}\begin{matrix}x+y=1\\y=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=0\end{matrix}\right.\)

\(Q=-x^2+6x+1=-\left(x^2-6x+9\right)+10=-\left(x-3\right)^2+10\le10\)
Dấu "=" xảy ra khi và chỉ khi x = 3
Vậy Max Q = 10 khi và chỉ khi x = 3

Có: Q=−x2+6x+1=−(x2−6x−1)=−(x2−6x+9−10)=−(x−3)2+10≤10
=> Max Q = 10
Dấu "=" <=> x=3

TXĐ: D=[-2,2]
P'=\(1-\frac{x}{\sqrt{4-x^2}}\)
P'=0<=> \(1-\frac{x}{\sqrt{4-x^2}}=0\)=>\(\hept{\begin{cases}x=\sqrt{4-x^2}\\4-x^2>0\end{cases}}\)
\(\hept{\begin{cases}x^2=4-x^2\\x\ge0\\-2< x< 2\end{cases}}\)
=> \(x=\sqrt{2}\)
P(-2)=-2
\(P\left(\sqrt{2}\right)=2\sqrt{2}\)
P(2)=2
Vậy GTLN của P=\(2\sqrt{2}\),GTNN là -2

đặt y = 1/x suy ra y <=1,
ta có P = 1 -2y+2016y^2
Tự làm tiếp nhé

\(\dfrac{3x^2-1}{x^2+2}=\dfrac{6x^2-2}{2\left(x^2+2\right)}=\dfrac{7x^2-\left(x^2+2\right)}{2\left(x^2+2\right)}=\dfrac{7x^2}{2\left(x^2+2\right)}-\dfrac{1}{2}\ge=-\dfrac{1}{2}\)
GTNN của biểu thức là \(-\dfrac{1}{2}\), xảy ra khi \(x=0\)
Biểu thức ko tồn tại GTLN

a) \(A=x^2+3x+4=\left(x+\dfrac{3}{2}\right)^2+\dfrac{7}{4}\ge\dfrac{7}{4}\)
\(minA=\dfrac{7}{4}\Leftrightarrow x=-\dfrac{3}{2}\)
b) \(B=2x^2-x+1=2\left(x-\dfrac{1}{4}\right)^2+\dfrac{7}{8}\ge\dfrac{7}{8}\)
\(minB=\dfrac{7}{8}\Leftrightarrow x=\dfrac{1}{4}\)
c) \(C=5x^2+2x-3=5\left(x+\dfrac{1}{5}\right)^2-\dfrac{16}{5}\ge-\dfrac{16}{5}\)
\(minC=-\dfrac{16}{5}\Leftrightarrow x=-\dfrac{1}{5}\)
d) \(D=4x^2+4x-24=\left(2x+1\right)^2-25\ge-25\)
\(minD=-25\Leftrightarrow x=-\dfrac{1}{2}\)
e) \(E=x^2+6x-11=\left(x+3\right)^2-20\ge-20\)
\(minE=-20\Leftrightarrow x=-3\)
f) \(G=\dfrac{1}{4}x^2+x-\dfrac{1}{3}=\left(\dfrac{1}{2}x+1\right)^2-\dfrac{4}{3}\ge-\dfrac{4}{3}\)
\(minG=-\dfrac{4}{3}\Leftrightarrow x=-2\)
\(A=x^2+3x+4=\left(x^2+3x+\dfrac{9}{4}\right)+\dfrac{7}{4}=\left(x+\dfrac{3}{2}\right)^2+\dfrac{7}{4}\)
Do \(\left(x+\dfrac{3}{2}\right)^2\ge0\forall x\)
\(\Rightarrow A=\left(x+\dfrac{3}{2}\right)^2+\dfrac{7}{4}\ge\dfrac{7}{4}\)
\(minA=\dfrac{7}{4}\Leftrightarrow x+\dfrac{3}{2}=0\Leftrightarrow x=-\dfrac{3}{2}\)
Mấy câu còn lại làm tương tự nhé em^^

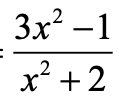
b) Sai đề minh sửu lại nha
\(\left(x^2+36y^2+12xy\right):\left(x+6y\right)\)
\(\Leftrightarrow\left(x+6y\right)^2:\left(x+6y\right)=x+6y\)
Tìm GTLN
\(P\left(x\right)=-2x^2+6x+2016=-2\left(x^2-3x+\frac{9}{4}\right)+\frac{4041}{2}=-2\left(x-\frac{3}{2}\right)^2+\frac{4041}{2}\)
Vì: \(-2\left(x-\frac{3}{2}\right)^2\le0\)
=> \(-2\left(x-\frac{3}{2}\right)^2+\frac{4041}{2}\le\frac{4041}{2}\)
Vậy GTLN của P(x) là \(\frac{4041}{2}\) khi \(x=\frac{3}{2}\)