Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(P=\left[tan\dfrac{17\pi}{4}+tan\left(\dfrac{7\pi}{2}-x\right)\right]^2+\left[cot\dfrac{13\pi}{4}+cot\left(7\pi-x\right)\right]^2\)
\(=\left[tan\dfrac{\pi}{4}+tan\left(-\dfrac{\pi}{2}-x\right)\right]^2+\left[cot\left(-\dfrac{3\pi}{4}\right)+cot\left(-\pi-x\right)\right]^2\)
\(=\left[tan\dfrac{\pi}{4}-cotx\right]^2+\left[tan\dfrac{\pi}{4}-cotx\right]^2\)
\(=2\left(1-cotx\right)^2\)

Ta có: \(\sin {70^o} = \cos {20^o};\;\cos {110^o} = - \cos {70^o} = - \sin {20^o}\)
\(\begin{array}{l} \Rightarrow A = {(\sin {20^o} + \cos {20^o})^2} + {(\cos {20^o} - \sin {20^o})^2}\\ = ({\sin ^2}{20^o} + {\cos ^2}{20^o} + 2\sin {20^o}\cos {20^o}) + ({\cos ^2}{20^o} + {\sin ^2}{20^o} - 2\sin {20^o}\cos {20^o})\\ = 2({\sin ^2}{20^o} + {\cos ^2}{20^o})\\ = 2\end{array}\)
Ta có: \(\tan {110^o} = - \tan {70^o} = - \cot {20^o};\;\cot {110^o} = - \cot {70^o} = - \tan {20^o}.\)
\( \Rightarrow B = \tan {20^o} + \cot {20^o} + ( - \cot {20^o}) + ( - \tan {20^o}) = 0\)

Thì tách bình thường thôi :)
\(A=\left[\tan\left(4\pi+\frac{\pi}{4}\right)+\tan\left(3\pi+\frac{\pi}{2}-x\right)\right]^2+\left[\cot\left(4\pi+\frac{\pi}{4}\right)+\cot\left(-x\right)\right]^2\)
\(A=\left[\tan\left(\frac{\pi}{4}\right)+\cot x\right]^2+\left[\cot\left(\frac{\pi}{4}\right)-\cot x\right]^2\)
\(A=\left(1+\cot x\right)^2+\left(1-\cot x\right)^2=...\)

a)
\(\cos\dfrac{22\pi}{3}=\cos\left(8\pi-\dfrac{2\pi}{3}\right)\\ =\cos\left(-\dfrac{2\pi}{3}\right)\\ =\cos\left(\dfrac{2\pi}{3}\right)\\ =-\cos\dfrac{\pi}{3}\\ =-\dfrac{1}{2}\)
b)
\(\sin\dfrac{23\pi}{4}=\sin\left(6\pi-\dfrac{\pi}{4}\right)\\ =\sin\left(-\dfrac{\pi}{4}\right)\\ =-\dfrac{\sqrt{2}}{2}\)
c)
\(\sin\dfrac{25\pi}{3}-\tan\dfrac{10\pi}{3}\\ =\sin\left(8\pi+\dfrac{\pi}{3}\right)-\tan\left(3\pi+\dfrac{\pi}{3}\right)\\ =\sin\dfrac{\pi}{3}-\tan\dfrac{\pi}{3}\\ =\dfrac{\sqrt{3}}{2}-\sqrt{3}\\ =\dfrac{-\sqrt{3}}{2}\)
d)
\(\cos^2\dfrac{\pi}{8}-\sin^2\dfrac{\pi}{8}\\ =\cos\dfrac{\pi}{4}\\ =\dfrac{\sqrt{2}}{2}\)
cau a: \(cos\dfrac{22\Pi}{3}=cos\dfrac{24\Pi-2\Pi}{3}=cos\left(8\Pi-\dfrac{2\Pi}{3}\right)=cos\dfrac{2\Pi}{3}=-\dfrac{1}{2}\)
câu b: \(sin\dfrac{23\Pi}{4}=sin\dfrac{24\Pi-\Pi}{4}=sin\left(6\Pi-\dfrac{\Pi}{4}\right)=-sin\dfrac{\Pi}{4}=-\dfrac{\sqrt{2}}{2}\)
cau c: \(=sin\left(8\Pi-\dfrac{\Pi}{3}\right)-tan\left(3\Pi+\dfrac{\Pi}{3}\right)=-sin\dfrac{\Pi}{3}-tan\dfrac{\Pi}{3}=-\dfrac{\sqrt{3}}{2}-\sqrt{3}=\dfrac{-3\sqrt{3}}{2}\)
cau d: \(cos^2\dfrac{\Pi}{8}-sin^2\dfrac{\Pi}{8}=cos2\left(\dfrac{\Pi}{8}\right)=cos\dfrac{\Pi}{4}=\dfrac{\sqrt{2}}{2}\)

a/ \(\frac{\pi}{6}< x< \frac{\pi}{3}\Rightarrow cosx>0\)
\(cos^2x=\frac{1}{1+tan^2x}=\frac{1}{10}\)
\(cotx=\frac{1}{tanx}=\frac{1}{3}\)
Thay số và bấm máy
b/ \(\frac{\pi}{2}< a< \pi\Rightarrow\left\{{}\begin{matrix}sina>0\\tana< 0\end{matrix}\right.\)
\(sina=\sqrt{1-cos^2a}=\frac{3}{5}\)
\(tana=\frac{sina}{cosa}=-\frac{3}{4}\)
\(A=\frac{6sina.cosa-\frac{2tana}{1-tan^2a}}{cosa-\left(2cos^2a-1\right)}\)
Thay số và bấm máy
c/ \(\frac{3\pi}{2}< x< 2\pi\Rightarrow\left\{{}\begin{matrix}cosx>0\\sinx< 0\end{matrix}\right.\)
\(cosx=\frac{1}{\sqrt{1+tan^2x}}=\frac{1}{\sqrt{5}}\)
\(sinx=cosx.tanx=-\frac{2}{\sqrt{5}}\)
\(B=\frac{cos^2x+2sinx.cosx}{\frac{2tanx}{1-tan^2x}-\left(2cos^2x-1\right)}\)
Thay số

a:
2: pi/2<a<pi
=>sin a>0 và cosa<0
tan a=-2
1+tan^2a=1/cos^2a=1+4=5
=>cos^2a=1/5
=>\(cosa=-\dfrac{1}{\sqrt{5}}\)
\(sina=\sqrt{1-\dfrac{1}{5}}=\dfrac{2}{\sqrt{5}}\)
cot a=1/tan a=-1/2
3: pi<a<3/2pi
=>cosa<0; sin a<0
1+cot^2a=1/sin^2a
=>1/sin^2a=1+9=10
=>sin^2a=1/10
=>\(sina=-\dfrac{1}{\sqrt{10}}\)
\(cosa=-\dfrac{3}{\sqrt{10}}\)
tan a=1:cota=1/3
b;
tan x=-2
=>sin x=-2*cosx
\(A=\dfrac{2\cdot sinx+cosx}{cosx-3sinx}\)
\(=\dfrac{-4cosx+cosx}{cosx+6cosx}=\dfrac{-3}{7}\)
2: tan x=-2
=>sin x=-2*cosx
\(B=\dfrac{-4cosx+3cosx}{-6cosx-2cosx}=\dfrac{1}{8}\)

Nhìn đề bài hãi quá :(
a/ \(A=3\sin\left(5.2\pi+\pi-x\right).\sin\left(2\pi+\frac{\pi}{2}-x\right)+2\sin\left(4.2\pi+\pi+x\right)\)
\(A=3\sin\left(\pi-x\right).\sin\left(\frac{\pi}{2}-x\right)+2\sin\left(\pi+x\right)\)
\(A=3\sin x.\cos x-2\sin x=\sin x\left(3\cos x-2\right)\)
b/ \(B=\sin\left(5.2.180^0+180^0+x\right)-\cos\left(90^0-x\right)+\tan\left(90^0+180^0-x\right)+\cot\left(2.180^0-x\right)\)
\(B=\sin\left(180^0+x\right)-\sin x+\tan\left(90^0-x\right)+\cot\left(-x\right)\)
\(B=-\sin x-\sin x+\cot x-\cot x=-2\sin x\)
c/ \(C=-2\sin\left(-(2\pi+\frac{\pi}{2}-x)\right)-3\cos\left(2\pi+\pi-x\right)+5\sin\left(2.2\pi-\left(\frac{\pi}{2}+x\right)\right)+\cot\left(\pi+\frac{\pi}{2}-x\right)\)
\(C=2\sin\left(\frac{\pi}{2}-x\right)-3\cos\left(\pi-x\right)-5\sin\left(\frac{\pi}{2}+x\right)+\cot\left(\frac{\pi}{2}-x\right)\)
\(2\cos x+3\cos x-5\cos x+\tan x=\tan x\)
d/ \(D=\tan\left(-\left(\pi-x\right)\right).\cos\left(-\left(\frac{\pi}{2}-x\right)\right).\left(-\cos x\right)\)
\(D=\tan\left(\pi-x\right).\cos\left(\frac{\pi}{2}-x\right).\cos x\)
\(D=-\tan x.\sin x.\cos x=-\sin^2x\)
e/ \(E=\cos\left(28.2\pi+\pi+\frac{\pi}{2}-x\right)+\sin\left(-\left(58.2\pi+\pi+\frac{\pi}{2}-x\right)\right)+\cos\left(-\left(46.2\pi+\pi+\frac{\pi}{2}-x\right)\right)+\sin\left(35.2\pi+\pi+\frac{\pi}{2}-x\right)\)
\(E=-\cos\left(\frac{\pi}{2}-x\right)+\sin\left(\frac{\pi}{2}-x\right)-\cos\left(\frac{\pi}{2}-x\right)-\sin\left(\frac{\pi}{2}-x\right)\)
\(E=-2\sin x\)
Thôi, stop ở đây, làm nữa chắc tẩu hỏa nhập ma quá :(
Mình thấy hầu hết các bài này đều có chung 1 điểm, và chắc đó cũng là điểm mà bạn thắc mắc: Đó chính là tách các hạng tử ra và biến đổi
Tách cũng đơn giản thôi, cứ gặp sin, cos thì tách sao cho về dạng 2pi+..., gặp tan, cot thì pi.
Còn tách mấy cái phân số như vầy:
Ví dụ \(\frac{7\pi}{2}\) , 7 chia 2 được 3, ta lấy \(\frac{7}{2}-3=\frac{1}{2}\) thì suy ra: \(\frac{7\pi}{2}=3\pi+\frac{\pi}{2}\)
Đó, thế là được :D

`0<α<π/2 => {(sinα>0),(cosα>0):}`
`tanα+cotα=2`
Mà `tanα.cotα=1`
`=>` \(\left\{{}\begin{matrix}tan\text{α}=1\\cot\text{α}=1\end{matrix}\right.\)
`=> α=π/4`
`=> sinα=cosα=\sqrt2/2`
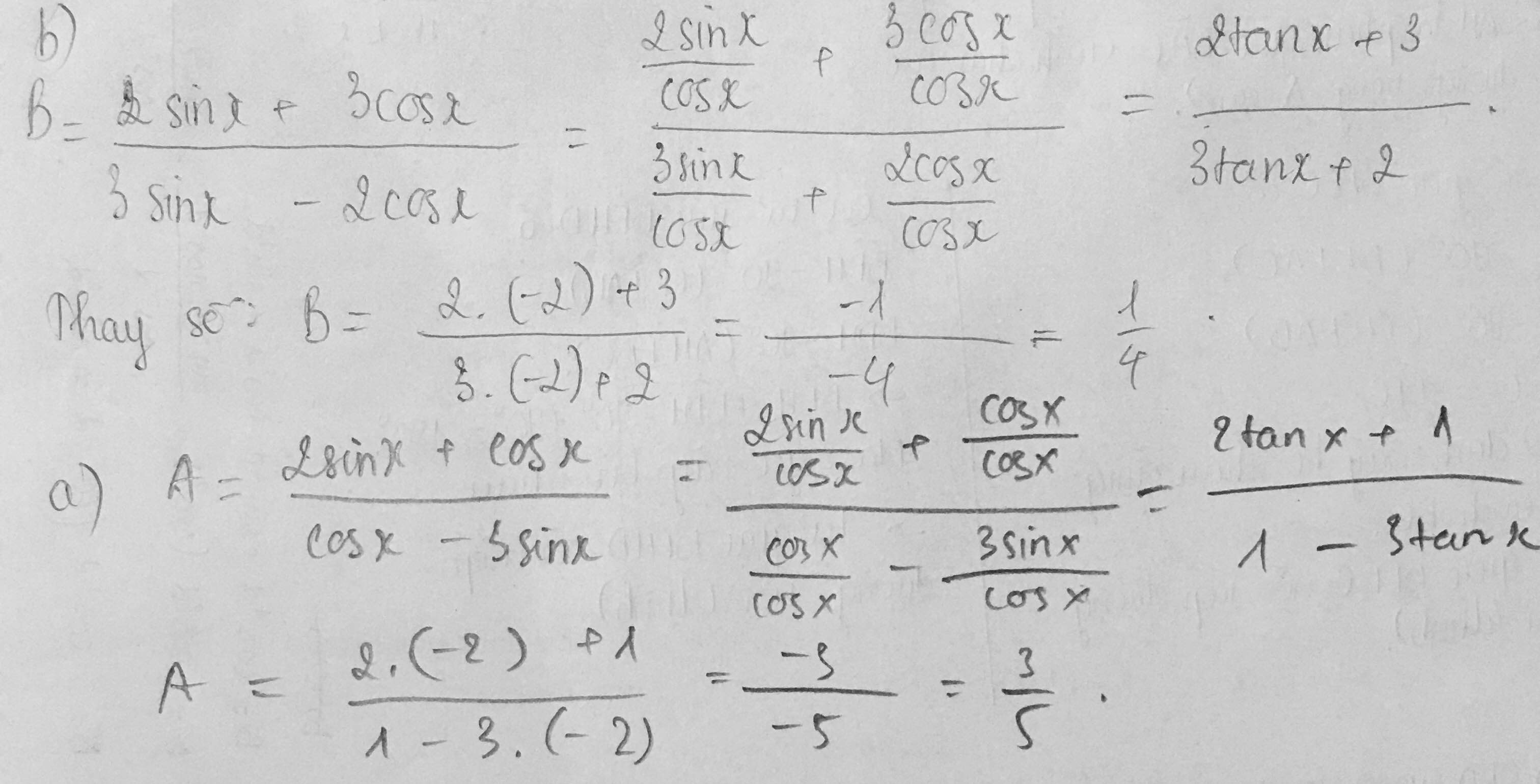
Đặt \(\frac{\pi}{8}=x\)
\(B=cotx-tanx=\frac{cosx}{sinx}-\frac{sinx}{cosx}\)
\(=\frac{cos^2x-sin^2x}{sinx.cosx}=\frac{cos2x}{\frac{1}{2}2sinxcosx}=\frac{2cos2x}{sin2x}=2cot2x\)
\(=2cot\left(\frac{2\pi}{8}\right)=2cot\frac{\pi}{4}=2\)