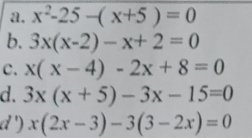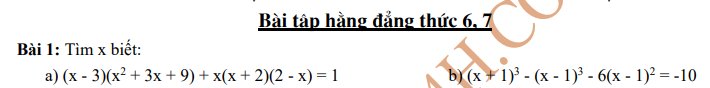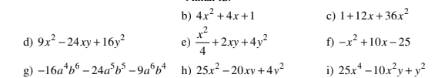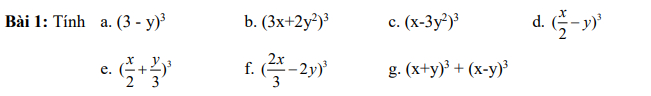K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
23 tháng 7 2023
e: =>(3x-1-x-5)(3x-1+x+5)=0
=>(2x-6)(4x+4)=0
=>x=3 hoặc x=-1
f: =>(2x-1-x+3)(2x-1+x-3)=0
=>(x+2)(3x-4)=0
=>x=4/3 hoặc x=-2
g: =>4x^2-4x+1-4x^2+1=0
=>-4x+2=0
=>x=1/2
g: =>(x^2+4)(x^2-1)=0
=>x^2-1=0
=>x=1 hoặc x=-1
m: =>(x+3)(2-x)=0
=>x=2 hoặc x=-3
l: =>(x-2)(x^2+2x+4-x+12)=0
=>(x-2)(x^2+x+16)=0
=>x-2=0
=>x=2
k: =>(2x-5)(2x+5-2x-7)=0
=>2x-5=0
=>x=5/2
NL
23 tháng 7 2023
\(a.x^2-25-\left(x+5\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-5\right)-\left(x+5\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+5=0\\x-6=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-5\\x=6\end{matrix}\right.\)
\(b.3x\left(x-2\right)-x+2=0\)
\(\Leftrightarrow3x\left(x-2\right)-\left(x-2\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\\x-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=2\end{matrix}\right.\)
\(c.x\left(x-4\right)-2x+8=0\)
\(\Leftrightarrow x\left(x-4\right)-2\left(x-4\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=4\end{matrix}\right.\)
\(d.3x\left(x+5\right)-3x-15=0\)
\(\Leftrightarrow3x\left(x+5\right)-3\left(x+5\right)=0\)
\(\Leftrightarrow\left(3x-3\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-3=0\\x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-5\end{matrix}\right.\)
\(d'.x\left(2x-3\right)-3\left(3-2x\right)=0\)
\(\Leftrightarrow x\left(2x-3\right)+3\left(2x-3\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(2x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\2x-3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=\dfrac{3}{2}\end{matrix}\right.\)
23 tháng 7 2023
a: =>(x+5)(x-5-1)=0
=>(x+5)(x-6)=0
=>x=-5 hoặc x=6
b: =>(x-2)(3x-1)=0
=>x=2 hoặc x=1/3
c: =>(x-4)(x-2)=0
=>x=4 hoặc x=2
d: =>(x+5)(3x-5)=0
=>x=5/3 hoặc x=-5
e: =>x(2x-3)+3(2x-3)=0
=>(2x-3)(x+3)=0
=>x=3/2 hoặc x=-3
H9
HT.Phong (9A5)
CTVHS
30 tháng 6 2023
a) \(\left(x-3\right)\left(x^2+3x+9\right)-x\left(x+2\right)\left(2-x\right)=1\)
\(\Leftrightarrow\left(x-3\right)\left(x^2+3x+9\right)+x\left(x+2\right)\left(x-2\right)=1\)
\(\Leftrightarrow\left(x^3-3^3\right)-x\left(x+2\right)\left(x-2\right)=1\)
\(\Leftrightarrow x^3-27-x\left(x^2-2^2\right)=1\)
\(\Leftrightarrow x^3-27-x^3+4x=1\)
\(\Leftrightarrow4x-27=1\)
\(\Leftrightarrow4x=28\)
\(\Leftrightarrow x=7\)
b) \(\left(x+1\right)^3-\left(x-1\right)^3-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow\left[\left(x+1\right)-\left(x-1\right)\right]\left[\left(x+1\right)^2+\left(x-1\right)\left(x+1\right)+\left(x-1\right)^2\right]-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow2\left[\left(x+1\right)^2+\left(x^2-1\right)+\left(x-1\right)^2\right]-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow2\left[\left(x^2+2x+1\right)+\left(x^2-1\right)+\left(x^2-2x+1\right)\right]-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow2\left(x^2+2x+1+x^2-1+x^2-2x+1\right)-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow2\left(3x^2+1\right)-6\left(x-1\right)^2=-10\)
\(\Leftrightarrow6x^2+2-6\left(x^2-2x+1\right)=-10\)
\(\Leftrightarrow6x^2+2-6x^2+12x-6=-10\)
\(\Leftrightarrow12x-4=-10\)
\(\Leftrightarrow12x=-6\)
\(\Leftrightarrow x=-\dfrac{6}{12}=-\dfrac{1}{2}\)
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
30 tháng 6 2023
\(a,\left(x-3\right)\left(x^2+3x+9\right)+x\left(x+2\right)\left(2-x\right)=1\\ \Leftrightarrow x^3-3x^2+3x^2-9x+9x-27+\left(x^2+2x\right)\left(2-x\right)-1=0\\ \Leftrightarrow x^3-3x^2+3x^2-9x+9x-27+2x^2-x^3+4x-2x^2-1=0\\ \Leftrightarrow x^3-x^3-3x^2+3x^2+2x^2-2x^2-9x+9x+4x=1+27\\ \Leftrightarrow4x=28\\ \Leftrightarrow x=7\)
2 tháng 8 2023
b: 4x^2+4x+1=(2x+1)^2
c: =(6x+1)^2
d: =(3x-4y)^2
e: =(1/2x+2y)^2
f: =-(x^2-10x+25)
=-(x-5)^2
g: =-(16a^4b^6+24a^5b^5+9a^6b^4)
=-a^4b^4(16b^2+24ab+9a^2)
=-a^4b^2(4b+3a)^2
h: =(5x)^2-2*5x*2y+(2y)^2
=(5x-2y)^2
i: =(5x^2)^2-2*5x^2*y+y^2
=(5x^2-y)^2
H9
HT.Phong (9A5)
CTVHS
2 tháng 8 2023
d) \(\left(3x+1\right)^2-4\left(x-2\right)^2\)
\(=\left[\left(3x+1\right)-2\left(x-2\right)\right]\left[\left(3x+1\right)+2\left(x-2\right)\right]\)
\(=\left(3x+1-2x+4\right)\left(3x+1+2x-4\right)\)
\(=\left(x+5\right)\left(5x-3\right)\)
c) \(9\left(2x+3\right)^2-4\left(x+1\right)^2\)
\(=\left[3\left(2x+3\right)-2\left(x+1\right)\right]\left[3\left(2x+3\right)+2\left(x+1\right)\right]\)
\(=\left(6x+9-2x-2\right)\left(6x+9+2x+2\right)\)
\(=\left(4x-7\right)\left(8x+11\right)\)
f) \(4b^2c^2-\left(b^2+c^2-a^2\right)^2\)
\(=\left[2bc-\left(b^2+c^2-a^2\right)\right]\left[2bc+\left(b^2+c^2-a^2\right)\right]\)
\(=\left(2bc-b^2-c^2+a^2\right)\left(2bc+b^2+c^2-a^2\right)\)
2 tháng 8 2023
g: =(ax+by-ay-bx)(ax+by+ay+bx)
=[a(x-y)-b(x-y)]*[a(x+y)+b(x+y)]
=(x-y)(x+y)(a-b)(a+b)
h: =(a^2+b^2-5-2ab-4)(a^2+b^2-5+2ab+4)
=[(a-b)^2-9][(a+b)^2-1]
=(a-b-3)(a-b+3)(a+b-1)(a+b+1)
i: =(4x^2-3x-18-4x^2-3x)(4x^2-3x-18+4x^2+3x)
=(-6x-18)(8x^2-18)
=-12(x+3)(4x^2-9)
=-12(x+3)(2x-3)(2x+3)
k: =(3x+3y-3)^2-(4x+6y+2)^2
=(3x+3y-3-4x-6y-2)(3x+3y-3+4x+6y+2)
=(-x-3y-5)(7x+9y-1)
i: =25-(2x-3y)^2
=(5-2x+3y)(5+2x-3y)
m: =(x-y)^2-(2m-n)^2
=(x-y-2m+n)(x-y+2m-n)
A
23 tháng 6 2023
\(a,3\left(2a-1\right)+5\left(3-a\right)\)
\(=6a-3+15-5a\)
\(=a-12\)
Thay \(a=\dfrac{-3}{2}\) vào biểu thức trên
\(a-12\)
\(=\dfrac{-3}{2}-12\)
\(=\dfrac{-27}{2}\)
\(b,25x-4\left(3x-1\right)+7\left(5-2x\right)\)
\(=25x-12x+4+35-14x\)
\(=-1x+39\)
Thay \(x=2,1\) vào biểu thức trên
\(-1x+39\)
\(=-1.2,1+39\)
\(=-2,1+39\)
\(=36,9\)
\(c,4a-2\left(10a-1\right)+8a-2\)
\(=4a-20a+2+8a-2\)
\(=-8a\)
Thay \(a=-0,2\) vào biểu thức trên
\(-8a\)
\(=-8.\left(-0,2\right)\)
\(=1,6\)
\(d,12\left(2-3b\right)+35b-9\left(b+1\right)\)
\(=24-36b+35b-9b-9\)
\(=-10b-15\)
Thay \(b=\dfrac{1}{2}\) vào biểu thức trên
\(-10b-15\)
\(=-10.\dfrac{1}{2}-15\)
\(=-20\)
23 tháng 6 2023
a: =6y^3-3y^2-y^2+2y-y+y^2-y^3
=5y^3-3y^2+y
b: =2x^2a-a-2x^2a-a-x^2-ax
=-x^2-ax-2a
c: =2p^3-p^3+1+2p^3+6p^2-3p^5
=3p^3+6p^2-3p^5+1
d: =-3a^3+5a^2+4a^3-4a^2=a^3+a^2
H9
HT.Phong (9A5)
CTVHS
2 tháng 8 2023
a) \(x^3+6x^2+12x+8\)
\(=x^3+3\cdot x^2\cdot2+3\cdot2^2\cdot x+2^3\)
\(=\left(x+2\right)^3\)
b) \(x^3-3x^2+3x-1\)
\(=x^3-3\cdot x^2\cdot1+3\cdot x\cdot1^2-1^3\)
\(=\left(x-1\right)^3\)
c) \(1-9x+27x^2-27x^3\)
\(=1^3-3\cdot3x\cdot1^2+3\cdot\left(3x\right)^2\cdot1-\left(3x\right)^3\)
\(=\left(1-3x\right)^3\)
d) \(x^3+\dfrac{3}{2}x^2+\dfrac{3}{4}x+\dfrac{1}{8}\)
\(=x^3\cdot3\cdot\dfrac{1}{2}\cdot x^2+3\cdot\left(\dfrac{1}{2}\right)^2\cdot x+\left(\dfrac{1}{2}\right)^3\)
\(=\left(x+\dfrac{1}{2}\right)^3\)
e) \(27x^3-54x^2y+36xy^2-8y^3\)
\(=\left(3x\right)^3-3\cdot\left(3x\right)^2\cdot y+3\cdot x\cdot\left(2y\right)^2-\left(2y\right)^3\)
\(=\left(3x-2y\right)^3\)
AH
Akai Haruma
Giáo viên
29 tháng 6 2023
d.
$(\frac{x}{2}-y)^3=(\frac{x}{2})^3-3(\frac{x}{2})^2y+3.\frac{x}{2}y^2-y^3$
$=\frac{x^3}{8}-\frac{3x^2y}{4}+\frac{3xy^2}{2}-y^3$
e.
$(\frac{x}{2}+\frac{y}{3})^3=(\frac{x}{2})^3+3(\frac{x}{2})^2\frac{y}{3}+3.\frac{x}{2}(\frac{y}{3})^2+(\frac{y}{3})^3$
$=\frac{x^3}{8}+\frac{x^2y}{4}+\frac{xy^2}{6}+\frac{y^3}{27}$
f.
$(\frac{2x}{3}-2y)^3=(\frac{2x}{3})^3-3(\frac{2x}{3})^2.2y+3.\frac{2x}{3}(2y)^2-(2y)^3$
$=\frac{8x^3}{27}-\frac{8x^2y}{3}+8xy^2-8y^3$
g.
$(x+y)^3+(x-y)^3=(x^3+3x^2y+3xy^2+y^3)+(x^3-3x^2y+3xy^2-y^3)$
$=2x^3+6xy^2$
AH
Akai Haruma
Giáo viên
29 tháng 6 2023
Lời giải:
a.
$(3-y)^3=3^3-3.3^2y+3.3y^2-Y63=27-27y+9y^2-y^3$
b.
$(3x+2y^2)^3=(3x)^3+3.(3x)^2(2y^2)+3.3x(2y^2)^2+(2y^2)^3$
$=8y^6+24xy^4+24x^2y^2+8x^3$
c.
$(x-3y^2)^3=x^3-3x^2(3y^2)+3x(3y^2)^2-(3y^2)^3$
$=x^3-9x^2y^2+27xy^4-27y^6$