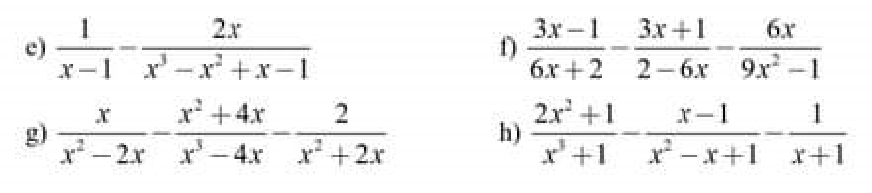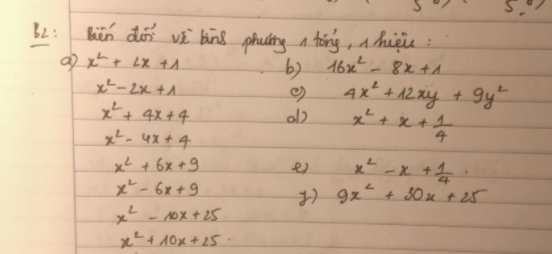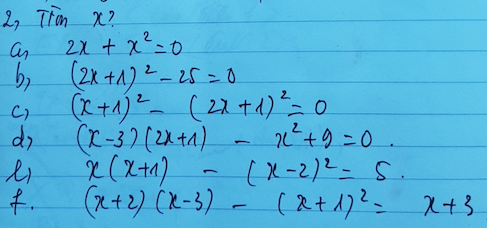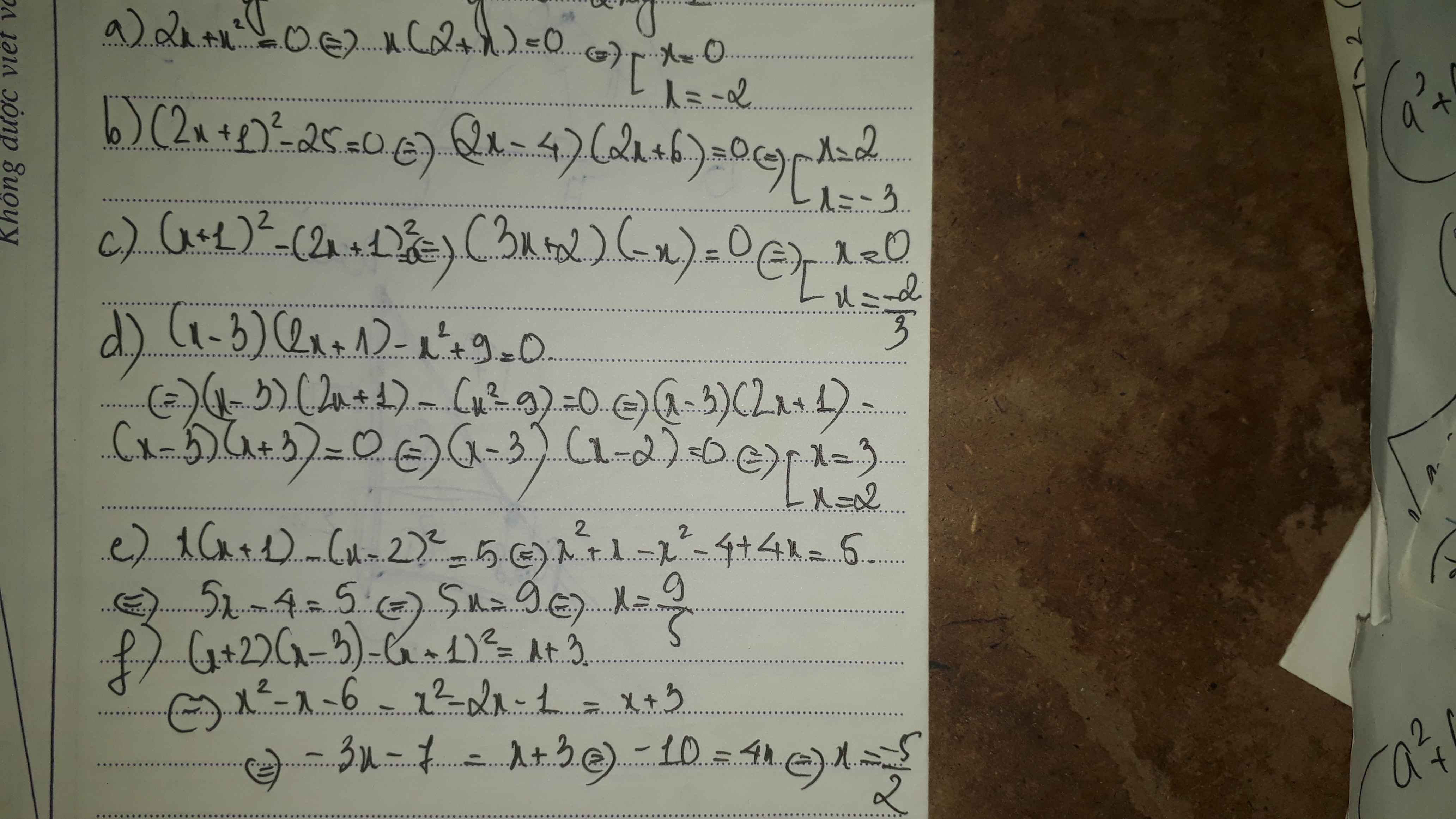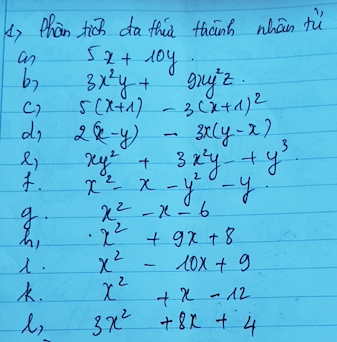Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


g: \(=\dfrac{x^2+2x-x^2-4x-2x+4}{x\left(x-2\right)\left(x+2\right)}=\dfrac{-4x+4}{x\left(x-2\right)\left(x+2\right)}\)
h: \(=\dfrac{2x^2+1-x^2+1-x^2+x-1}{\left(x+1\right)\left(x^2-x+1\right)}\)
\(=\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{1}{x^2-x+1}\)
\(e,=\dfrac{1}{x-1}-\dfrac{2x}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{x^2-2x+1}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{\left(x-1\right)^2}{\left(x^2+1\right)\left(x-1\right)}=\dfrac{x-1}{x^2+1}\\ f,=\dfrac{3x-1}{2\left(3x+1\right)}+\dfrac{3x+1}{2\left(3x-1\right)}-\dfrac{6x}{\left(3x-1\right)\left(3x+1\right)}\\ =\dfrac{9x^2-6x+1+9x^2+6x+1-12x}{2\left(3x-1\right)\left(3x+1\right)}=\dfrac{2\left(3x-1\right)^2}{2\left(3x-1\right)\left(3x+1\right)}=\dfrac{3x-1}{3x+1}\)
\(g,=\dfrac{x}{x\left(x-2\right)}-\dfrac{x^2+4x}{x\left(x-2\right)\left(x+2\right)}-\dfrac{2}{x\left(x+2\right)}\\ =\dfrac{x^2+2x-x^2-4x-2x+4}{x\left(x-2\right)\left(x+2\right)}=\dfrac{-4x+4}{x\left(x-2\right)\left(x+2\right)}\\ h,=\dfrac{2x^2+1-x^2+1-x^2+x-1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{x+1}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{1}{x^2-x+1}\)

e: \(E=\dfrac{x^2-9-x^2+4-x^2+9}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{x+2}{x+3}\)
a: \(A=\dfrac{4x^2+x^2-2x+1+x^2+2x+1}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{6x^2+2}{\left(x-1\right)\left(x+1\right)}\)

a) \(x^2+2x+1=\left(x+1\right)^2\)
\(x^2-2x+1=\left(x-1\right)^2\)
\(x^2+4x+4=\left(x+2\right)^2\)
\(x^2-4x+4=\left(x-2\right)^2\)
\(x^2+6x+9=\left(x+3\right)^2\)
\(x^2-6x+9=\left(x-3\right)^2\)
\(x^2-10x+25=\left(x-5\right)^2\)
\(x^2+10x+25=\left(x+5\right)^2\)
b) \(16x^2-8x+1=\left(4x-1\right)^2\)
c) \(4x^2+12xy+9y^2=\left(2x+3y\right)^2\)
d) \(x^2+x+\dfrac{1}{4}=\left(x+\dfrac{1}{2}\right)^2\)
e) \(x^2-x+\dfrac{1}{4}=\left(x-\dfrac{1}{2}\right)^2\)
f) \(9x^2+30x+25=\left(3x+5\right)^2\)

a) Ta có: \(2x+x^2=0\)
\(\Leftrightarrow x\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\end{matrix}\right.\)
b) Ta có: \(\left(2x+1\right)^2-25=0\)
\(\Leftrightarrow\left(2x-4\right)\left(2x+6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-3\end{matrix}\right.\)

a) \(5x+10y=5\left(x+2y\right)\)
b) \(3x^2y+9xy^2z=3xy\left(x+3yz\right)\)
g) \(x^2-x-6=\left(x-3\right)\left(x+2\right)\)
h) \(x^2+9x+8=\left(x+8\right)\left(x+1\right)\)
l) \(x^2-10x+9=\left(x-1\right)\left(x-9\right)\)
k) \(x^2+x-12=\left(x+4\right)\left(x-3\right)\)
l) \(3x^2+8x+4=\left(3x+2\right)\left(x+2\right)\)

a: Xét ΔADM và ΔCBN có
AD=CB
\(\widehat{DAM}=\widehat{BCN}\)
AM=CN
Do đó: ΔADM=ΔCBN
Suy ra: DM=BN

\(a,\left(2x+3\right).5x=10x^2.15x\)
\(b,1011^2-1010^2=\left(1011-1010\right)\left(1011+1010\right)=2021\)
\(c,x^2+3x=x\left(x+3\right)\)
\(c,x^2+2xy-x-2y=\left(x^2-x\right)+\left(2xy-2y\right)=x\left(x-1\right)+2y\left(x-1\right)=\left(x-1\right)\left(x+2y\right)\)