
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



\(a)x^2-9x+20=0 \\<=>(x-4)(x-5)=0 \\<=>x=4\ hoặc\ x=5 \\b)x^2-3x-18=0 \\<=>(x+3)(x-6)=0 \\<=>x=-3\ hoặc\ x=6 \\c)2x^2-9x+9=0 \\<=>(x-3)(2x-3)=0 \\<=>x=3\ hoặc\ x=\dfrac{3}{2}\)
d: \(\Leftrightarrow3x^2-6x-2x+4=0\)
=>(x-2)(3x-2)=0
=>x=2 hoặc x=2/3
e: \(\Leftrightarrow3x\left(x^2-2x-3\right)=0\)
=>x(x-3)(x+1)=0
hay \(x\in\left\{0;3;-1\right\}\)
f: \(\Leftrightarrow x^2-5x-2+x=0\)
\(\Leftrightarrow x^2-4x-2=0\)
\(\Leftrightarrow\left(x-2\right)^2=6\)
hay \(x\in\left\{\sqrt{6}+2;-\sqrt{6}+2\right\}\)

`2x^3 +6x^2 =x^2 +3x`
`<=> 2x^3 +6x^2 -x^2 -3x=0`
`<=> 2x^3 +5x^2 -3x=0`
`<=> x(2x^2 +5x-3)=0`
`<=> x(2x^2 +6x-x-3)=0`
`<=> x[2x(x+3)-(x+3)]=0`
`<=> x(2x-1)(x+3)=0`
\(< =>\left[{}\begin{matrix}x=0\\2x-1=0\\x+3=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=0\\x=\dfrac{1}{2}\\x=-3\end{matrix}\right.\)
b)
`(2+x)^2 -(2x-5)^2=0`
`<=> (2+x-2x+5)(2+x+2x-5)=0`
`<=> (-x+7)(3x-3)=0`
\(< =>\left[{}\begin{matrix}-x+7=0\\3x-3=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=7\\x=1\end{matrix}\right.\)
`a) 2x^3 + 6x^2 = x^2 + 3x`
`=> 2x^3 + 6x^2 - x^2 - 3x = 0`
`=> 2x^3 + 5x^2 - 3x = 0`
`=> x(2x^2 + 5x - 3) = 0`
`=> x (2x^2 + 6x - x - 3) = 0`
`=> x [(2x^2 + 6x) - (x+3)] = 0`
`=> x [2x(x+3) - (x+3)] = 0`
`=> x (2x - 1)(x+3) = 0`
`=> x = 0` hoặc `2x - 1 = 0` hoặc `x + 3 = 0`
`=> x = 0` hoặc `x = 1/2` hoặc `x = -3`
`b) (2+x)^2 - (2x-5)^2 = 0`
`=> (2+x+2x-5)(2+x-2x+5) = 0`
`=> (3x - 3)(7-x) = 0`
`=> 3x - 3 = 0` hoặc `7 - x = 0`
`=> x = 1` hoặc `x = 7`

Ta có : x4+3x3+4x2+3x+1=0
⇔ ( x4 + x3 ) + ( 2x3 + 2x2 ) + ( 2x2 + 2x ) + ( x + 1 ) = 0
⇔ x3 ( x + 1 ) + 2x2 ( x + 1 ) + 2x ( x+1 ) + ( x + 1 ) =0
⇔ ( x + 1 ) ( x3 + 2x2 + 2x + 1 ) = 0
⇔ ( x + 1 ) [ ( x3 + 1 ) + ( 2x2 + 2x ) ] = 0
⇔ ( x + 1 ) [ (x + 1 ) ( x2 - x +1 ) + 2x ( x + 1 ) ] =0
⇔ ( x +1 ) ( x + 1 ) ( x2 + x +1 ) =0
⇒ \(\left[{}\begin{matrix}x+1=0\\x^{2^{ }}+x+1=0\end{matrix}\right.\)<=> \(\left[{}\begin{matrix}x=-1\\\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}=0\left(VoLy\right)\end{matrix}\right.\)
Vậy x = -1
x4+3x3+4x2+3x+1=0
⇔(x4+2x3+x2)+(x3+2x2+1)+(x2+2x+1)=0
⇔x2(x2+2x+1)+x(x2+2x+1)+(x2+2x+1)=0
⇔x2(x+1)2+x(x+1)2+(x+1)2=0
⇔(x+1)2(x2+x+1)=0
Vì x2+x+1=x2+x+\(\dfrac{1}{4}\)+\(\dfrac{3}{4}\)=(x+\(\dfrac{1}{2}\))2+\(\dfrac{3}{4}\)>0 nên phương trình đã cho tương đương:
(x+1)2=0 ⇔(x+1)(x+1)=0 ⇔x=-1.

\(a,6x^2-5x+3=2x-3x\left(3-2x\right)\)
\(\Leftrightarrow6x^2-5x+3=2x-9x+6x^2\)
\(\Leftrightarrow6x^2-6x^2-5x-2x+9x=-3\)
\(\Leftrightarrow2x=-3\)
\(\Leftrightarrow x=-\dfrac{3}{2}\)
\(b,\left(3x-1\right)\left(4x+3\right)=2\left(3x-1\right)\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3\right)-2\left(3x-1\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\\4x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=-\dfrac{1}{4}\end{matrix}\right.\)
\(6x^2-5x+3=2x-3x\left(3-2x\right)\)
\(\Leftrightarrow6x^2-5x+3=2x-9x+6x^2\)
\(\Leftrightarrow6x^2-5x+3-2x+9x-6x^2=0\)
\(\Leftrightarrow2x+3=0\)
\(\Leftrightarrow2x=-3\)
\(\Leftrightarrow x=\dfrac{-3}{2}\)
\(\text{Vậy phương trình có tập nghiệm là }S=\left\{\dfrac{-3}{2}\right\}\)
\(\left(3x-1\right)\left(4x+3\right)=2\left(3x-1\right)\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3\right)-2\left(3x-1\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x+3-2\right)=0\)
\(\Leftrightarrow\left(3x-1\right)\left(4x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=0\\4x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=1\\4x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{3}\\x=\dfrac{1}{4}\end{matrix}\right.\)
\(\text{Vậy phương trình có tập nghiệm là }S=\left\{\dfrac{1}{3};\dfrac{1}{4}\right\}\)

1) Ta có: \(x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
hay x=2
Vậy: S={2}

2x3 + 6x2 = x2 + 3x
⇔ (2x3 + 6x2) – (x2 + 3x) = 0
⇔ 2x2(x + 3) – x(x + 3) = 0
⇔ x(x + 3)(2x – 1) = 0
(Nhân tử chung là x(x + 3))
⇔ x = 0 hoặc x + 3 = 0 hoặc 2x – 1 = 0
+ x + 3 = 0 ⇔ x = -3.
+ 2x – 1 = 0 ⇔ 2x = 1 ⇔ x = 1/2.
Vậy tập nghiệm của phương trình là 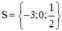

1.
a/ \(\Leftrightarrow\left(x+1\right)\left(x^2+3x+2\right)+\left(x-1\right)\left(x^2-3x+2\right)-12=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2\right)+3x\left(x+1\right)-3x\left(x-1\right)+\left(x-1\right)\left(x^2+2\right)-12=0\)
\(\Leftrightarrow2x\left(x^2+2\right)+6x^2-12=0\)
\(\Leftrightarrow x^3+3x^2+2x-6=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2+4x+6\right)=0\Rightarrow x=1\)
b/ Nhận thấy \(x=0\) ko phải nghiệm, chia 2 vế cho \(x^2\)
\(x^2+\frac{1}{x^2}+3\left(x+\frac{1}{x}\right)+4=0\)
Đặt \(x+\frac{1}{x}=t\Rightarrow x^2+\frac{1}{x^2}=t^2-2\)
\(t^2-2+3t+4=0\Rightarrow t^2+3t+2=0\Rightarrow\left[{}\begin{matrix}t=-1\\t=-2\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x+\frac{1}{x}=-1\\x+\frac{1}{x}=-2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2+x+1=0\left(vn\right)\\x^2+2x+1=0\end{matrix}\right.\) \(\Rightarrow x=-1\)
1c/
\(\Leftrightarrow x^5+x^4-2x^4-2x^3+5x^3+5x^2-2x^2-2x+x+1=0\)
\(\Leftrightarrow x^4\left(x+1\right)-2x^3\left(x+1\right)+5x^2\left(x+1\right)-2x\left(x+1\right)+x+1=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^4-2x^3+5x^2-2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^4-2x^3+5x^2-2x+1=0\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow x^4-2x^3+x^2+x^2-2x+1+3x^2=0\)
\(\Leftrightarrow\left(x^2-x\right)^2+\left(x-1\right)^2+3x^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-x=0\\x-1=0\\x=0\end{matrix}\right.\) \(\Rightarrow\) không tồn tại x thỏa mãn
Vậy pt có nghiệm duy nhất \(x=-1\)
Ta thấy x = 0 ko phải là nghiệm của pt => x khác 0
Chia cả 2 vế pt cho x^2 khác 0 ta được :
x^2-3x-6+3/x+1/x^2 = 0
<=> (x^2+1/x^2)-3.(x-1/x)-6 = 0
Đặt x-1/x = a => x^2+1/x^2 = a^2+2
pt trở thành :
a^2+2-3a-6 = 0
<=> a^2-3a-4 = 0
<=> (a^2+a)-(4a+4) = 0
<=> (a+1).(a-4) = 0
<=> a=-1 hoặc a=4
<=> x-1/x = -1 hoặc x-1/x = 4
Đến đó nhân cả 2 vế với x mà tìm x nha
Tk mk nha
x = 0 không là nghiệm của pt.
\(x\ne0\)
\(PT\Leftrightarrow x^2+\frac{1}{x^2}-3x+\frac{3}{x}+6=0\Leftrightarrow\left(x-\frac{1}{2}\right)^2-3\left(x-\frac{1}{x}\right)+8=0\)<=> PT vô nghiệm