K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
CM
16 tháng 5 2019
x 2 + (x + 2)(11x – 7) = 4
⇔ x 2 – 4 + (x + 2)(11x – 7) = 0
⇔ (x + 2)(x – 2) + (x + 2)(11x – 7) = 0
⇔ (x + 2)[(x – 2) + (11x – 7)] = 0
⇔ (x + 2)(x – 2 + 11x – 7) = 0
⇔ (x + 2)(12x – 9) = 0 ⇔ x + 2 = 0 hoặc 12x – 9 = 0
x + 2 = 0 ⇔ x = - 2
12x – 9 = 0 ⇔ x = 0,75
Vậy phương trình có hai nghiệm x = - 2 hoặc x = 0,75
PL
1
MV
8 tháng 1 2018
\(x^4+2x^3-2x^2+2x-3=0\\ \Leftrightarrow x^4+3x^3-x^3-3x^2+x^2+3x-x-3=0\\ \Leftrightarrow x^3\left(x+3\right)-x^2\left(x+3\right)+x\left(x+3\right)-\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left(x^3-x^2+x-1\right)=0\\ \Leftrightarrow\left(x+3\right)\left[x^2\left(x-1\right)+\left(x-1\right)\right]=0\\ \Leftrightarrow\left(x+3\right)\left(x-1\right)\left(x^2+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-1=0\\x^2+1=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-3\\x=1\end{matrix}\right.\left(\text{vì }x^2+1\ge1>0\right)\)
Vậy ...
\(\left(x-1\right)\left(x^2+5x-2\right)-x^3+1=0\\ \Leftrightarrow\left(x-1\right)\left(x^2+5x-2\right)-\left(x^3-1\right)=0\\ \Leftrightarrow\left(x-1\right)\left(x^2+5x-2\right)-\left(x-1\right)\left(x^2+x+1\right)=0\\ \Leftrightarrow\left(x-1\right)\left[\left(x^2+5x-2\right)-\left(x^2+x+1\right)\right]=0\\ \Leftrightarrow\left(x-1\right)\left(4x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-1=0\\4x-3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=1\\x=\dfrac{3}{4}\end{matrix}\right.\)
Vậy ...
\(x^2+\left(x+2\right)\left(11x-7\right)=4\\ \Leftrightarrow x^2-4+\left(x+2\right)\left(11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(x-2\right)+\left(11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(x-2+11x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(12x-9\right)=0\\ \Leftrightarrow3\left(x+2\right)\left(4x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+2=0\\4x-3=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{3}{4}\end{matrix}\right.\)
Vậy ...
27 tháng 2 2018
nghiệm đâu bạn ưi...nó là phương trình vô nghiệm hay vô số nghiệm vậy m :))
LY
1
D
1
18 tháng 1 2022
\(a.x^2-11x+15=-15.\Leftrightarrow x^2-11x+30=0.\)
\(\Leftrightarrow\left(x-6\right)\left(x-5\right)=0.\Leftrightarrow\left[{}\begin{matrix}x=6.\\x=5.\end{matrix}\right.\)
\(b.2x-3x+10=x.\Leftrightarrow-2x+10=0.\Leftrightarrow x=5.\)
\(c.x^3-4=4.\Leftrightarrow x^3=8.\Leftrightarrow x^3=2^3.\Rightarrow x=2.\)
\(d.x^4+x^3-x^2-x=0.\Leftrightarrow x^2\left(x^2+x\right)-\left(x^2+x\right)=0.\Leftrightarrow\left(x^2-1\right)\left(x^2+x\right)=0.\)
\(\Leftrightarrow\left(x-1\right)\left(x+1\right)x\left(x+1\right)=0.\Leftrightarrow\left(x-1\right)\left(x+1\right)^2x=0.\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0.\\x+1=0.\\x=0.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=1.\\x=-1.\\x=0.\end{matrix}\right.\)
NN
1
12 tháng 2 2023
\(\Leftrightarrow\dfrac{1}{\left(x+2\right)\left(x+3\right)}+\dfrac{1}{\left(x+3\right)\left(x+4\right)}+...+\dfrac{1}{\left(x+5\right)\left(x+6\right)}=\dfrac{1}{8}\)
=>\(\dfrac{1}{x+2}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+4}+...+\dfrac{1}{x+5}-\dfrac{1}{x+6}=\dfrac{1}{8}\)
=>1/x+2-1/x+6=1/8
=>\(\dfrac{x+6-x-2}{\left(x+2\right)\left(x+6\right)}=\dfrac{1}{8}\)
=>x^2+8x+12=32
=>x^2+8x-20=0
=>(x+10)(x-2)=0
=>x=-10 hoặc x=2
AH
Akai Haruma
Giáo viên
24 tháng 8 2023
1. Đặt $x^2+x=a$ thì pt trở thành:
$a^2+4a=12$
$\Leftrightarrow a^2+4a-12=0$
$\Leftrightarrow (a-2)(a+6)=0$
$\Leftrightarrow a-2=0$ hoặc $x+6=0$
$\Leftrightarrow x^2+x-2=0$ hoặc $x^2+x+6=0$
Dễ thấy $x^2+x+6=0$ vô nghiệm.
$\Rightarrow x^2+x-2=0$
$\Leftrightarrow (x-1)(x+2)=0$
$\Leftrightarrow x=1$ hoặc $x=-2$
AH
Akai Haruma
Giáo viên
24 tháng 8 2023
2.
$x(x-1)(x+1)(x+2)=24$
$\Leftrightarrow [x(x+1)][(x-1)(x+2)]=24$
$\Leftrightarrow (x^2+x)(x^2+x-2)=24$
$\Leftrightarrow a(a-2)=24$ (đặt $x^2+x=a$)
$\Leftrightarrow a^2-2a-24=0$
$\Leftrightarrow (a+4)(a-6)=0$
$\Leftrightarrow a+4=0$ hoặc $a-6=0$
$\Leftrightarrow x^2+x+4=0$ hoặc $x^2+x-6=0$
Nếu $x^2+x+4=0$
$\Leftrightarrow (x+\frac{1}{2})^2=\frac{1}{4}-4<0$ (vô lý - loại)
Nếu $x^2+x-6=0$
$\Leftrightarrow (x-2)(x+3)=0$
$\Leftrightarrow x-2=0$ hoặc $x+3=0$
$\Leftrightarrow x=2$ hoặc $x=-3$
NN
Nguyễn Ngọc Anh Minh
CTVHS
VIP
26 tháng 7 2022
<=> x4+3x3+x2+3x3+9x2+3x+x2+3x+1=0
<=>x2(x2+3x+1)+3x(x2+3x+1)+(x2+3x+1)=0
<=> (x2+3x+1)(x2+3x+1)=0
<=>(x2+3x+1)2=0 => x2+3x+1=0 Giải PT bậc 2 để tìm x, bạn tự làm nốt nhé
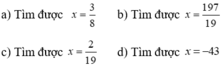
\(x^2+\left(x+2\right)\left(11x-7\right)=4\)
\(\Leftrightarrow x^2+\left(11x^2+15x-14\right)=4\)
\(\Leftrightarrow12x^2+15x-18=0\)
\(\Leftrightarrow12x^2+24x-9x-18=0\)
\(\Leftrightarrow12x\left(x+2\right)-9\left(x+2\right)=0\)
\(\Leftrightarrow\left(12x-9\right)\left(x+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}12x-9=0\\x+2=0\end{cases}\Rightarrow\orbr{\begin{cases}x=\frac{3}{4}\\x=-2\end{cases}}}\)
Vậy nghiệm của phương trình là : \(S=\left\{-2;\frac{3}{4}\right\}\)