K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
28 tháng 5 2019
x2 – 5x + 6 = 0
⇔ x2 – 2x – 3x + 6 = 0
(Tách để xuất hiện nhân tử chung)
⇔ (x2 – 2x) – (3x – 6) = 0
⇔ x(x – 2) – 3(x – 2) = 0
⇔(x – 3)(x – 2) = 0
⇔ x – 3 = 0 hoặc x – 2 = 0
+ x – 3 = 0 ⇔ x = 3.
+ x – 2 = 0 ⇔ x = 2.
Vậy tập nghiệm của phương trình là S = {2; 3}.
PT
1
CM
24 tháng 4 2017
– x 2 + 5x – 6 = 0 ⇔ - x 2 + 2x + 3x – 6 = 0
⇔ - x(x – 2) + 3(x – 2) = 0 ⇔ (x – 2)(3 – x) = 0
⇔ x – 2 = 0 hoặc 3 – x = 0
x – 2 = 0 ⇔ x = 2
3 – x = 0 ⇔ x = 3
Vậy phương trình có nghiệm x = 2 hoặc x = 3.
N
giải các Phương trình sau
a) (5x+3)(x2+1)(x-1)=0
b) (4x-1)(x-3)-(x-3)(5x+2)=0
c) (x+6)(3x-1)+x2-36 =0
1
23 tháng 2 2023
a: =>(5x+3)(x-1)=0
=>x=1 hoặc x=-3/5
b: =>(x-3)(4x-1-5x-2)=0
=>(x-3)(-x-3)=0
=>x=-3 hoặc x=3
c: =>(x+6)(3x-1+x-6)=0
=>(x+6)(4x-7)=0
=>x=7/4 hoặc x=-6
6 tháng 3 2021
1) Ta có: \(x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
hay x=2
Vậy: S={2}
5 tháng 7 2019
3x2 + 2x - 1 = 0
=> 3x2 + 3x - x - 1 = 0
=> 3x(x + 1) - (x + 1) = 0
=> (3x - 1)(x + 1) = 0
=> \(\orbr{\begin{cases}3x-1=0\\x+1=0\end{cases}}\)
=> \(\orbr{\begin{cases}x=\frac{1}{3}\\x=-1\end{cases}}\)
x2 - 5x + 6 = 0
=> x2 - 2x - 3x + 6 = 0
=> x(x - 2) - 3(x - 2) = 0
=> (x - 3)(x - 2) = 0
=> \(\orbr{\begin{cases}x-3=0\\x-2=0\end{cases}}\)
=> \(\orbr{\begin{cases}x=3\\x=2\end{cases}}\)
3x2 + 7x + 2 = 0
=> 3x2 + 6x + x + 2 = 0
=> 3x(x + 2) + (x + 2) = 0
=> (3x + 1)(x + 2) = 0
=> \(\orbr{\begin{cases}3x+1=0\\x+2=0\end{cases}}\)
=> \(\orbr{\begin{cases}x=-\frac{1}{3}\\x=-2\end{cases}}\)
5 tháng 7 2019
1, \(3x^2+2x-1=0\Leftrightarrow3x^2+3x-x-1=0\)
\(\Leftrightarrow3x\left(x+1\right)-\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(3x-1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+1=0\\3x-1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-1\\x=\frac{1}{3}\end{cases}}}\)
2, \(x^2-5x+6=0\Leftrightarrow x^2-2x-3x+6=0\)
\(\Leftrightarrow x\left(x-2\right)-3\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-2=0\\x-3=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=2\\x=3\end{cases}}}\)
3, \(3x^2+7x+2=0\Leftrightarrow3x^2+6x+x+2=0\)
\(\Leftrightarrow3x\left(x+2\right)+\left(x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(3x+1\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+2=0\\3x+1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-2\\x=-\frac{1}{3}\end{cases}}}\)
PT
1
CM
26 tháng 3 2017
2 x 2 + 5x + 3 = 0 ⇔ 2 x 2 + 2x + 3x + 3 = 0
⇔ 2x(x + 1) + 3(x + 1) = 0 ⇔ (2x + 3)(x + 1) = 0
⇔ 2x + 3 = 0 hoặc x + 1 = 0
2x + 3 = 0 ⇔ x = -1,5
x + 1 = 0 ⇔ x = -1
Vậy phương trình có nghiệm x = -1,5 hoặc x = -1
13 tháng 2 2022
i,<=>(2x - 1)(2x - 1 + 2 - x) = 0 <=> (2x - 1)(x + 1) = 0
<=> x = 1/2 hoặc x = -1
j,<=>(x - 1)(5x + 3) - (3x - 5)(x - 1) = 0
<=>(x - 1)(2x + 8) = 0 <=> x = 1 hoặc x = -4
k,<=>4(x + 5)(x - 6) = 0 <=> (x + 5)(x - 6) = 0
<=> x = -5 hoặc x = 6
m,<=>x^2(x + 1) + x + 1 = 0
<=>(x^2 + 1)(x + 1) = 0 (1)
Mà x^2 + 1 > 0 với mọi x nên (1) xảy ra <=> x + 1 = 0
<=> x = -1
PT
1
CM
30 tháng 1 2019
(x – 1)( x 2 + 5x – 2) – ( x 3 – 1) = 0
⇔ (x – 1)( x 2 + 5x – 2) – (x – 1)( x 2 + x + 1) = 0
⇔ (x – 1)[( x 2 + 5x – 2) – ( x 2 + x + 1)] = 0
⇔ (x – 1)( x 2 + 5x – 2 – x 2 – x – 1) = 0
⇔ (x – 1)(4x – 3) = 0 ⇔ x – 1 = 0 hoặc 4x – 3 = 0
x – 1 = 0 ⇔ x = 1
4x – 3 = 0 ⇔ x = 0,75
Vậy phương trình có nghiệm x = 1 hoặc x = 0,75
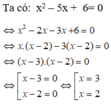
\(x^2+5x-6=0\)
\(\Leftrightarrow x^2+2x+3x-6=0\)
\(\Leftrightarrow-x\left(x-2\right)+3\left(x-2\right)=0\)
\(\Leftrightarrow\left(3-x\right)\left(x-2\right)=0\)
\(\Leftrightarrow\hept{\begin{cases}x=3\\x=2\end{cases}}\)
cảm ơn bn nha <:3