NS
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 7 2021
1.
\(\Leftrightarrow cos\left(2x+\dfrac{4\pi}{3}\right)=0\)
\(\Leftrightarrow2x+\dfrac{4\pi}{3}=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow2x=-\dfrac{5\pi}{6}+k\pi\)
\(\Leftrightarrow x=-\dfrac{5\pi}{12}+\dfrac{k\pi}{2}\)
b.
\(\Leftrightarrow2+2cos\left(2x+\dfrac{\pi}{3}\right)-3=0\)
\(\Leftrightarrow cos\left(2x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\2x+\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=-\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
c.
\(\Leftrightarrow cos\left(2x-\dfrac{\pi}{6}\right)=\dfrac{\sqrt{3}}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\dfrac{\pi}{6}=\dfrac{\pi}{6}+k2\pi\\2x-\dfrac{\pi}{6}=-\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k\pi\\x=k\pi\end{matrix}\right.\)
27 tháng 7 2021
cho em hỏi làm sao mà từ đề ra được ạ
b) \(\Leftrightarrow2+2cos\left(2x+\dfrac{\pi}{3}\right)-3=0\)
c)\(\Leftrightarrow cos\left(2x-\dfrac{\pi}{6}\right)=\dfrac{\sqrt{3}}{2}\)
25 tháng 8 2023
\(\Leftrightarrow2cos^2\left(x+\dfrac{pi}{3}\right)-1=0\)
=>\(cos\left(2x+\dfrac{2}{3}pi\right)=0\)
=>2x+2/3pi=pi/2+kpi
=>2x=-1/6pi+kpi
=>x=-1/12pi+kpi/2
mà \(x\in\left(-\dfrac{pi}{2};\dfrac{5}{6}pi\right)\)
nên \(x\in\left\{-\dfrac{1}{12}pi;\dfrac{5}{12}pi\right\}\)
NS
1
NV
Nguyễn Việt Lâm
Giáo viên
17 tháng 7 2021
\(\Leftrightarrow2\left(\dfrac{1}{2}cosx+\dfrac{\sqrt{3}}{2}sinx\right)+2cos\left(x-\dfrac{\pi}{3}\right)=2\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{3}\right)+cos\left(x-\dfrac{\pi}{3}\right)=1\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\x-\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2\pi}{3}+k2\pi\\x=k2\pi\end{matrix}\right.\)
TT
1
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 12 2020
ĐKXĐ: \(sinx\ne\pm1\)
\(\dfrac{3cos2x-2sinx+5}{2\left(1-sin^2x\right)}=0\)
\(\Leftrightarrow3\left(1-2sin^2x\right)-2sinx+5=0\)
\(\Leftrightarrow-6sin^2x-2sinx+8=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=1\left(loại\right)\\sinx=-\dfrac{4}{3}< -1\left(loại\right)\end{matrix}\right.\)
Vậy pt vô nghiệm
27 tháng 10 2023
a: \(2\cdot sin\left(x+\dfrac{\Omega}{5}\right)+\sqrt{3}=0\)
=>\(2\cdot sin\left(x+\dfrac{\Omega}{5}\right)=-\sqrt{3}\)
=>\(sin\left(x+\dfrac{\Omega}{5}\right)=-\dfrac{\sqrt{3}}{2}\)
=>\(\left[{}\begin{matrix}x+\dfrac{\Omega}{5}=-\dfrac{\Omega}{3}+k2\Omega\\x+\dfrac{\Omega}{5}=\dfrac{4}{3}\Omega+k2\Omega\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=-\dfrac{8}{15}\Omega+k2\Omega\\x=\dfrac{4}{3}\Omega-\dfrac{\Omega}{5}+k2\Omega=\dfrac{17}{15}\Omega+k2\Omega\end{matrix}\right.\)
b: \(sin\left(2x-50^0\right)=\dfrac{\sqrt{3}}{2}\)
=>\(\left[{}\begin{matrix}2x-50^0=60^0+k\cdot360^0\\2x-50^0=300^0+k\cdot360^0\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}2x=110^0+k\cdot360^0\\2x=350^0+k\cdot360^0\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=55^0+k\cdot180^0\\x=175^0+k\cdot180^0\end{matrix}\right.\)
c: \(\sqrt{3}\cdot tan\left(2x-\dfrac{\Omega}{3}\right)-1=0\)
=>\(\sqrt{3}\cdot tan\left(2x-\dfrac{\Omega}{3}\right)=1\)
=>\(tan\left(2x-\dfrac{\Omega}{3}\right)=\dfrac{1}{\sqrt{3}}\)
=>\(2x-\dfrac{\Omega}{3}=\dfrac{\Omega}{6}+k2\Omega\)
=>\(2x=\dfrac{1}{2}\Omega+k2\Omega\)
=>\(x=\dfrac{1}{4}\Omega+k\Omega\)
LL
1
HP
20 tháng 9 2021
\(cos\left(2x+\dfrac{\pi}{3}\right)+cos\left(x-\dfrac{\pi}{3}\right)=0\)
\(\Leftrightarrow2cos\dfrac{3x}{2}.cos\left(\dfrac{x}{2}+\dfrac{\pi}{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos\dfrac{3x}{2}=0\\cos\left(\dfrac{x}{2}+\dfrac{\pi}{3}\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{3x}{2}=\dfrac{\pi}{2}+k\pi\\\dfrac{x}{2}+\dfrac{\pi}{3}=\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+\dfrac{k2\pi}{3}\\x=\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
R
2
27 tháng 9 2021
Pt \(\Leftrightarrow\)\(tan\left(x+\dfrac{\pi}{3}\right)\)=\(-cot\left(\dfrac{\pi}{2}-3x\right)\)
\(\Leftrightarrow\)\(tan\left(x+\dfrac{\pi}{3}\right)\)=\(tan\left(\dfrac{\pi}{2}+\dfrac{\pi}{2}-3x\right)\)=\(tan\left(\pi-3x\right)\)
\(\Leftrightarrow\)\(x+\dfrac{\pi}{3}=\pi-3x+k\pi\)
\(\Leftrightarrow\)4\(x\)=\(\dfrac{4}{3}\pi+k\pi\)
\(\Leftrightarrow\) \(x=\) \(\dfrac{\pi}{3}+k\dfrac{\pi}{4}\)(\(k\in Z\))
HP
28 tháng 9 2021
\(pt\Leftrightarrow tan\left(x+\dfrac{\pi}{3}\right)=-cot\left(\dfrac{\pi}{2}-3x\right)\)
\(\Leftrightarrow tan\left(x+\dfrac{\pi}{3}\right)=cot\left(-\dfrac{\pi}{2}+3x\right)\)
\(\Leftrightarrow tan\left(x+\dfrac{\pi}{3}\right)=tan\left(\dfrac{\pi}{2}+\dfrac{\pi}{2}-3x\right)\)
\(\Leftrightarrow tan\left(x+\dfrac{\pi}{3}\right)=tan\left(\pi-3x\right)\)
\(\Leftrightarrow x+\dfrac{\pi}{3}=\pi-3x+k\pi\)
\(\Leftrightarrow4x=\dfrac{2\pi}{3}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+\dfrac{k\pi}{4}\)
NS
1
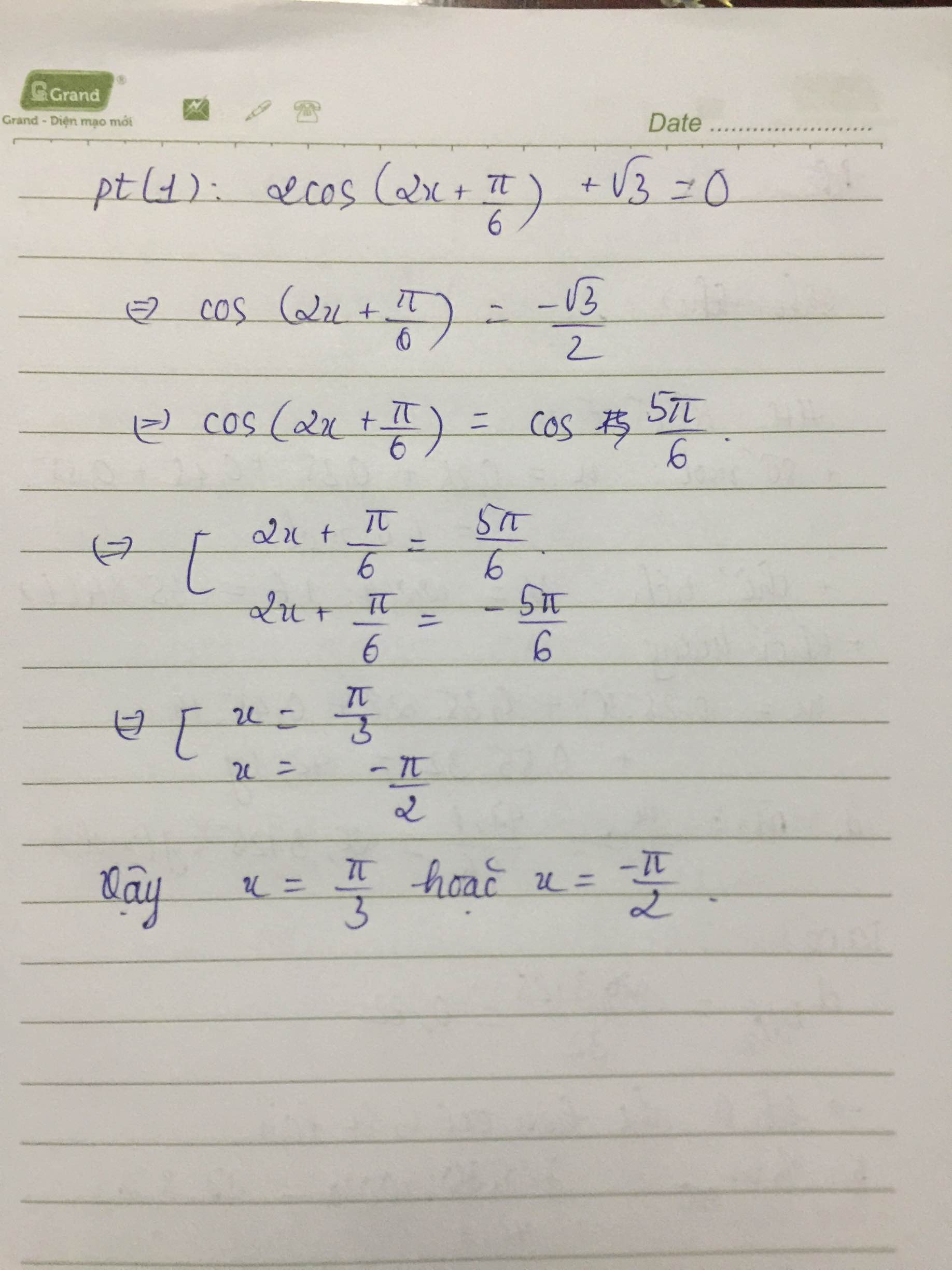
\(-1\le cosx\le1\)
Vô nghiệm nha a, \(cos\ge-1\)