K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AH
Akai Haruma
Giáo viên
22 tháng 6 2023
Bạn nên viết lại đề bài cho sáng sủa, rõ ràng để người đọc dễ hiểu hơn.
22 tháng 6 2023
f: =>4(x^2+4x-5)-x^2-7x-10=3(x^2+x-2)
=>4x^2+16x-20-x^2-7x-10-3x^2-3x+6=0
=>6x-24=0
=>x=4
e: =>8x+16-5x^2-10x+4(x^2-x-2)=4-x^2
=>-5x^2-2x+16+4x^2-4x-8=4-x^2
=>-6x+8=4
=>-6x=-4
=>x=2/3
d: =>2x^2+3x^2-3=5x^2+5x
=>5x=-3
=>x=-3/5
b: =>2x^2-8x+3x-12+x^2-7x+10=3x^2-12x-5x+20
=>-12x-2=-17x+20
=>5x=22
=>x=22/5
22 tháng 6 2023
b: =>2x^2-8x+3x-12+x^2-7x+10=3x^2-17x+20
=>-12x-2=-17x+20
=>5x=22
=>x=22/5
c: =>24x^2+16x-9x-6-4x^2-16x-7x-28=20x^2-4x+5x-1
=>-16x-34=x-1
=>-17x=33
=>x=-33/17
d: =>2x^2+3x^2-3=5x^2+5x
=>5x=-3
=>x=-3/5
e: =>8x+16-5x^2-10x+4x^2-4x-8=4-x^2
=>-6x+8=4
=>-6x=-4
=>x=2/3
f: =>4(x^2+4x-5)-x^2-7x-10=3x^2+3x-6
=>4x^2+16x-20-4x^2-10x+4=0
=>6x=16
=>x=8/3
12 tháng 7 2019
a) \(\frac{x}{x+1}=\frac{1}{2}\)
=> 2x = x + 1
=> 2x - x = 1
=> x = 1
b) \(\frac{x}{2}=\frac{x}{3}\)
=> 3x = 2x
=> 3x - 2x = 0
=> x = 0
c) \(\frac{x+1}{2}=\frac{x+1}{2017}\)
=> \(2017\left(x+1\right)=2\left(x+1\right)\)
=> 2017x + 2017 = 2x + 2
=> 2017x - 2x = 2 - 2017
=> 2015x = -2015
=> x = -2015 : 2015
=> x = -1
i) \(\frac{3}{x}=\frac{x}{2017}\)
=> x2 = 2017.3
=> x2 = 6051
=> \(\orbr{\begin{cases}x=\sqrt{6051}\\x=-\sqrt{6051}\end{cases}}\)
còn lại tự lm
12 tháng 7 2019
\(a,\frac{x}{x+1}=\frac{1}{2}\)
\(\Rightarrow x=\frac{1}{2}.\left(x+1\right)\)
\(\Rightarrow x=\frac{1}{2}x+\frac{1}{2}\)
\(\Rightarrow x-\frac{1}{2}x=\frac{1}{2}\)
\(\Rightarrow\frac{1}{2}x=\frac{1}{2}\)
\(\Rightarrow x=1\)
\(b,\frac{x}{2}=\frac{x}{3}\)
\(\Rightarrow x=\frac{x}{3}.2\)
\(\Rightarrow x=\frac{2x}{3}\)
\(\Rightarrow3x=2x\)
\(\Rightarrow x=0\)
\(c,\frac{x+1}{2}=\frac{x+1}{2017}\)
\(\Rightarrow x+1=\frac{x+1}{2017}.2\)
\(\Rightarrow x+1=\frac{2x+2}{2017}\)
\(\Rightarrow2017x+2017=2x+2\)
\(\Rightarrow2017x-2x=2-2017\)
\(\Rightarrow2015x=-2015\)
\(\Rightarrow x=-1\)
\(i,\frac{3}{x}=\frac{x}{2017}\)
\(\Rightarrow x=3:\frac{x}{2017}\)
\(\Rightarrow x=\frac{6051}{x}\)
\(\Rightarrow x^2=6051\)
\(\Rightarrow x=\sqrt{6051}\)
\(o,\frac{x}{3}=\frac{x+1}{2}\)
\(\Rightarrow x=\frac{x+1}{2}.3\)
\(\Rightarrow x=\frac{3x+3}{2}\)
\(\Rightarrow2x=3x+3\)
\(\Rightarrow-x=3\)
\(\Rightarrow x=-3\)
\(m,\frac{x+1}{2}=\frac{x+2}{3}\)
\(\Rightarrow x+1=\frac{x+2}{3}.2\)
\(\Rightarrow x+1=\frac{2x+4}{3}\)
\(\Rightarrow3x+3=2x+4\)
\(\Rightarrow x=1\)
\(p,\frac{x+1}{2}=x\)
\(\Rightarrow2x=x+1\)
\(\Rightarrow x=1\)
\(m,\frac{2}{x}=\frac{x}{8}\)
\(\Rightarrow x=2:\frac{x}{8}\)
\(\Rightarrow x=\frac{16}{x}\)
\(\Rightarrow x^2=16\)
\(\Rightarrow x=4\)
\(Q,\frac{x^2}{2}=\frac{8}{x^2}\)
\(\Rightarrow x^2=\frac{8}{x^2}.2\)
\(\Rightarrow x^2=\frac{16}{x^2}\)
\(\Rightarrow x^4=16\)
\(\Rightarrow x=2\)
\(r,\frac{x^3}{2}=\frac{32}{x}\)
\(\Rightarrow x^3=\frac{32}{x}.2\)
\(\Rightarrow x^3=\frac{64}{x}\)
\(\Rightarrow x^4=64\)
\(\Rightarrow x=\sqrt[4]{64}\)
NT
0
BC
1
H9
HT.Phong (9A5)
CTVHS
14 tháng 4 2023
1) \(x^2\left(2x^3-x^2+4x\right)\)
\(=2x^5-x^4+4x^3\)
2) \(\left(x+2\right)\left(5x^3-3x^2+x\right)\)
\(=5x^4-3x^3+x^2+10x^3-6x^2+2x\)
\(=5x^4+7x^3-5x^2+2x\)
3) \(\left(x^2-2\right)\left(x^2+2x-1\right)\)
\(=x^4+2x^3-x^2-2x^2-4x+2\)
\(=x^4+2x^3-3x^2-4x+2\)
4) \(\left(x^2+x+1\right)\left(x-1\right)\)
\(=x^3-x^2+x^2-x+x-1\)
\(=x^3-1\)
21 tháng 5 2022
a: \(\dfrac{x-6}{7}+\dfrac{x-7}{8}+\dfrac{x-8}{9}=\dfrac{x-9}{10}+\dfrac{x-10}{11}+\dfrac{x-11}{12}\)
\(\Leftrightarrow\left(\dfrac{x-6}{7}+1\right)+\left(\dfrac{x-7}{8}+1\right)+\left(\dfrac{x-8}{9}+1\right)=\left(\dfrac{x-9}{10}+1\right)+\left(\dfrac{x-10}{11}+1\right)+\left(\dfrac{x-11}{12}+1\right)\)
=>x+1=0
hay x=-1
c: |x-2|=13
=>x-2=13 hoặc x-2=-13
=>x=15 hoặc x=-11
d: \(\Leftrightarrow3\left|x-2\right|+4\left|x-2\right|=2-\dfrac{1}{3}=\dfrac{5}{3}\)
=>7|x-2|=5/3
=>|x-2|=5/21
=>x-2=5/21 hoặc x-2=-5/21
=>x=47/21 hoặc x=37/21
17 tháng 9 2023
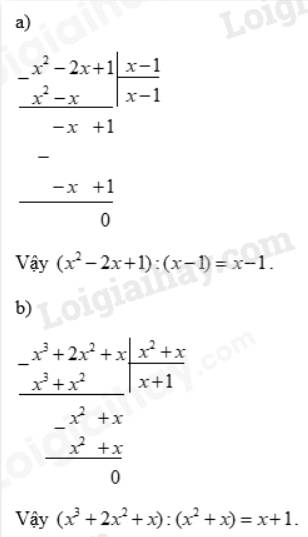
Vậy \(( - 32{x^5} + 1):( - 2x + 1) = 16{x^4} + 8{x^3} + 4{x^2} + 2x + 1\).
2 tháng 2
a: \(\left(x+\dfrac{1}{4}\right)+\left(3x-4\right)+2\left(x-3\right)=1\)
=>\(x+\dfrac{1}{4}+3x-4+2x-6=1\)
=>\(6x-\dfrac{39}{4}=1\)
=>\(6x=1+\dfrac{39}{4}=\dfrac{43}{4}\)
=>\(x=\dfrac{43}{4}:6=\dfrac{43}{24}\)
b: \(2\left(x-3\right)=3\left(x+2\right)-x+1\)
=>\(2x-6=3x+6-x+1\)
=>2x-6=2x+7
=>-6=7(vô lý)
c: \(x\left(x+3\right)+x\left(x-2\right)=2x\left(x-1\right)\)
=>\(x^2+3x+x^2-2x=2x^2-2x\)
=>3x-2x=-2x
=>3x=0
=>x=0
d: \(\left(x-1\right)\cdot3x-2\left(x+2\right)-2x=x\left(x-1\right)\)
=>\(3x^2-3x-2x-4-2x=x^2-x\)
=>\(3x^2-7x-4-x^2+x=0\)
=>\(2x^2-6x-4=0\)
=>\(x^2-3x-2=0\)
=>\(x=\dfrac{3\pm\sqrt{17}}{2}\)
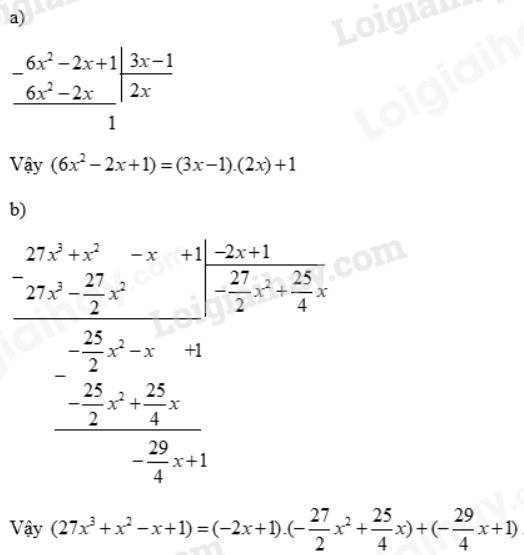
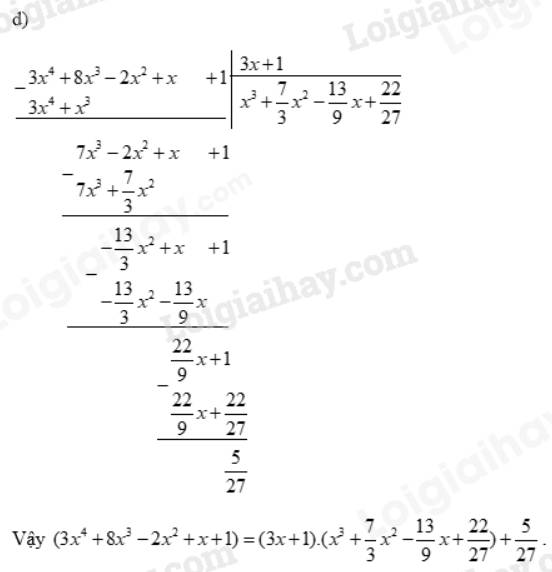
(\(x\) - 1) = 2 - \(x\)
\(x-1\) = 2 - \(x\)
\(x+x\) = 2 + 1
2\(x\) = 3
\(x=\dfrac{3}{2}\)
Vậy \(x=\dfrac{3}{2}\)