Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

(m-1)x+2m+1=y
=>(m-1)x-y+2m+1=0
\(d\left(O;d1\right)=\dfrac{\left|0\cdot\left(m-1\right)+0\cdot\left(-1\right)+2m+1\right|}{\sqrt{\left(m-1\right)^2+1}}=\dfrac{\left|2m+1\right|}{\sqrt{\left(m-1\right)^2+1}}\)
Để (d) lớn nhất thì \(\sqrt{\left(m-1\right)^2+1}_{Min}\)
=>m=1

Gọi \(M\left(2+2t;3+t\right)\)
M có tọa độ nguyên \(\Leftrightarrow t\) nguyên
\(\overrightarrow{AM}=\left(2+2t;2+t\right)\) \(\Rightarrow AM=\sqrt{\left(2+2t\right)^2+\left(2+t\right)^2}=5\)
\(\Leftrightarrow5t^2+12t-17=0\Rightarrow\left[{}\begin{matrix}t=1\\t=-\dfrac{17}{5}\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow M\left(4;4\right)\)

\(M\in d\Rightarrow M\left(3-2t;1+3t\right)\)
\(\Rightarrow\)\(\overrightarrow{AM}=\left(-1-2t;1+3t\right)\)
\(\Rightarrow AM=\sqrt{\left(-1-2t\right)^2+\left(1+3t\right)^2}=5\)
\(\Leftrightarrow13t^2+10t-23=0\Rightarrow\left[{}\begin{matrix}t=1\\t=\dfrac{-23}{13}\end{matrix}\right.\)
\(+t=1\Rightarrow M\left(1;4\right)\)
\(+t=\dfrac{-23}{13}\Rightarrow M=\left(\dfrac{85}{13};\dfrac{-56}{13}\right)\)
vậy có 2 điểm M cần tìm.

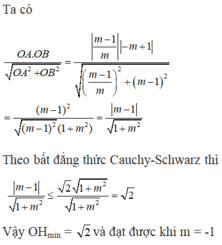

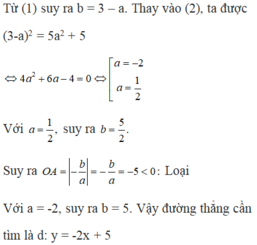
\(y=m\left(2x+1\right)-3x-3\Leftrightarrow-m\left(2x+1\right)+3x+y+3=0\)
\(\Rightarrow\) đường thẳng luôn đi qua điểm cố định \(M\left(-\frac{1}{2};-\frac{3}{2}\right)\)
Gọi H là hình chiếu vuông góc của O lên đường thẳng
\(\Rightarrow OH=d\)
Theo định lý đường xiên - đường vuông góc ta luôn có:
\(OH\le OM\Rightarrow OH_{max}=OM\) khi \(H\equiv M\)
Mà \(OM=\sqrt{\left(-\frac{1}{2}\right)^2+\left(-\frac{3}{2}\right)^2}=\frac{\sqrt{10}}{2}\Rightarrow d=\frac{\sqrt{10}}{2}\)