

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Hướng dẫn giải:
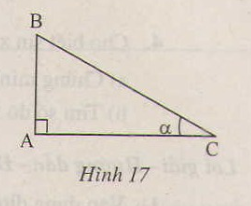
a) tgα=ABAC=AB⋅BCAC⋅BCtgα=ABAC=AB⋅BCAC⋅BC
⇒tgα=ABBC÷ACBC=sinαcosα⇒tgα=ABBC÷ACBC=sinαcosα
tgα⋅cotgα=ABAC⋅ACAB=1tgα⋅cotgα=ABAC⋅ACAB=1
cotgα=1tgα=1sinαcosα=cosαsinαcotgα=1tgα=1sinαcosα=cosαsinα
b) sin2α+cos2α=AB2BC2+AC2BC2=BC2BC2=1sin2α+cos2α=AB2BC2+AC2BC2=BC2BC2=1
Nhận xét: Ba hệ thức tgα=sinαcosαtgα=sinαcosα
cotgα=cosαsinα;sin2α+cos2α=1cotgα=cosαsinα;sin2α+cos2α=1 là những hệ thức cơ bản bạn cần nhớ để giải một số bài tập khá
a) tgα=ABAC=AB⋅BCAC⋅BCtgα=ABAC=AB⋅BCAC⋅BC
⇒tgα=ABBC÷ACBC=sinαcosα⇒tgα=ABBC÷ACBC=sinαcosα
tgα⋅cotgα=ABAC⋅ACAB=1tgα⋅cotgα=ABAC⋅ACAB=1
cotgα=1tgα=1sinαcosα=cosαsinαcotgα=1tgα=1sinαcosα=cosαsinα
b) sin2α+cos2α=AB2BC2+AC2BC2=BC2BC2=1sin2α+cos2α=AB2BC2+AC2BC2=BC2BC2=1
Nhận xét: Ba hệ thức tgα=sinαcosαtgα=sinαcosα
cotgα=cosαsinα;sin2α+cos2α=1cotgα=cosαsinα;sin2α+cos2α=1 là những hệ thức cơ bản bạn cần nhớ để giải một số bài tập khác.

Câu 1:
\(\cos a=\sqrt{1-0.28^2}=\dfrac{24}{25}\)
\(\tan a=\dfrac{0.28}{0.96}=\dfrac{7}{24}\)
\(\cot a=\dfrac{1}{\tan a}=\dfrac{24}{7}\)

\(\dfrac{\left(sina+cosa\right)^2-\left(sina-cosa\right)^2}{sina.cosa}=4\\ VT=\dfrac{sin^2a+2sinacosa+cos^2a-sin^2a+2sinacosa-cos^2a}{sinacosa}\\ =\dfrac{4sinacosa}{sinacosa}=4=VP\)
a: \(S=cos^2a\left(1+tan^2a\right)=cos^2a\cdot\dfrac{1}{cos^2a}=1\)
b: \(VP=\dfrac{1+sin2a-1+sin2a}{\dfrac{1}{2}\cdot sin2a}=\dfrac{2\cdot sin2a}{\dfrac{1}{2}\cdot sin2a}=4=VT\)

a/ \(\sin\alpha=\frac{C_đ}{C_h}\)
\(\cos\alpha=\frac{C_k}{C_h}\)
\(\Rightarrow\frac{\sin\alpha}{\cos\alpha}=\frac{\frac{C_đ}{C_h}}{\frac{C_k}{C_h}}=\frac{C_đ}{C_k}=\tan\alpha\)
b/ \(\frac{\cos\alpha}{\sin\alpha}=\frac{\frac{C_k}{C_h}}{\frac{C_đ}{C_h}}=\frac{C_k}{C_đ}=\cot\alpha\)
c/ \(\tan\alpha.\cot\alpha=\frac{C_đ}{C_k}.\frac{C_k}{C_đ}=1\)
d/ \(\sin^2\alpha=\frac{C_đ^2}{C_h^2}\)
\(\cos^2\alpha=\frac{C_k^2}{C_h^2}\)
\(\Rightarrow\sin^2\alpha+\cos^2\alpha=\frac{C_đ^2+C_k^2}{C_h^2}=\frac{C_h^2}{C_h^2}=1\)
P/s: hok trc lp 9 hay sao mà lm bài bài này?