
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: Ta có: \(\left(12x-5\right)\left(4x-1\right)+\left(3x-7\right)\left(1-16x\right)=81\)
\(\Leftrightarrow48x^2-32x+5+3x-48x^2-7+112x=81\)
\(\Leftrightarrow83x=83\)
hay x=1
b: Ta có: \(\left(4x-5\right)\left(3x-2\right)-\left(2x+6\right)\left(6x-1\right)=17\)
\(\Leftrightarrow12x^2-8x-15x+10-12x^2+2x-36x+6=17\)
\(\Leftrightarrow-57x=13\)
hay \(x=-\dfrac{13}{57}\)

đề đâu, mà giải chi tiết thì đăng 1 câu hỏi trong 1 lần đăng thôi
\(a,x^3+9x^2+27x+27=\left(x+3\right)^3\\ b,\dfrac{x^3}{8}+\dfrac{3}{4}x^2y^2+\dfrac{3}{2}xy^4+y^6=\left(\dfrac{x}{2}+y^2\right)^3\\ c,x^3+6x^2+12x+8=\left(x+2\right)^3\\ d,27x^3-54x^2y+36xy^2-8y^3=\left(3x-2y\right)^3\\ e,8x^6-12x^4+6x^2-1=\left(2x^2-1\right)^3\)

a) \(\left(5x+5\right)^2+10\left(x-3\right)\left(1+x\right)+x^2-6x+9=\left[5\left(x+1\right)\right]^2+2.5\left(x+1\right)\left(x-3\right)+\left(x-3\right)^2=\left[5\left(x+1\right)+\left(x-3\right)\right]^2=\left(5x+5+x-3\right)^2=\left(6x+2\right)^2=\left(6x\right)^2+2.6x.2+2^2=36x^2+24x+4\)
b) \(\left(\dfrac{x}{2}-2y\right)^2=\left(\dfrac{x}{2}\right)^2-2.\dfrac{x}{2}.2y+\left(2y\right)^2=\dfrac{x^2}{4}-2xy+4y^2\)
a: \(\left(5x+5\right)^2+10\left(x-3\right)\left(x+1\right)+x^2-6x+9\)
\(=\left[5\left(x+1\right)\right]^2+2\cdot5\left(x+1\right)\cdot\left(x-3\right)+\left(x-3\right)^2\)
\(=\left(5x+5+x-3\right)^2\)
\(=\left(6x+2\right)^2\)
b: \(\left(\dfrac{1}{2}x-2y\right)^2=\dfrac{1}{4}x^2-2xy+4y^2\)

ĐKXĐ: \(x\notin\left\{0;-9\right\}\)
Ta có: \(\dfrac{1}{x+9}-\dfrac{1}{x}=\dfrac{1}{5}+\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{20x}{20x\left(x+9\right)}-\dfrac{20\left(x+9\right)}{20x\left(x+9\right)}=\dfrac{4x\left(x+9\right)+5x\left(x+9\right)}{20x\left(x+9\right)}\)
Suy ra: \(4x^2+36x+5x^2+45x=20x-20x-180\)
\(\Leftrightarrow9x^2+81x+180=0\)
\(\Leftrightarrow x^2+9x+20=0\)
\(\Leftrightarrow x^2+4x+5x+20=0\)
\(\Leftrightarrow x\left(x+4\right)+5\left(x+4\right)=0\)
\(\Leftrightarrow\left(x+4\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+4=0\\x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-4\left(nhận\right)\\x=-5\left(nhận\right)\end{matrix}\right.\)
Vậy: S={-4;-5}

Gọi chiều rộng là x
Chiều dài là x+15
Theo đề, ta có phương trình:
\(\left(x+5\right)\left(x+12\right)=x\left(x+15\right)+80\)
\(\Leftrightarrow x^2+17x+60-x^2-15x=80\)
=>2x+60=80
=>x=10
Vậy: Chiều rộng là 10m
Chiều dài là 25m

Gọi độ dài quãng đường là x
Theo đề, ta có:
\(\dfrac{x}{42}-\dfrac{x}{46}=\dfrac{3}{4}\)
hay x=362,25(km)

Hướng làm:
Thấy cả tử mẫu cộng lại đều bằng 2021 → Cộng thêm 1 rồi quy đồng với mỗi phân thức
\(\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\\ \Leftrightarrow\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\\ \Leftrightarrow\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}\right)=0\\ \Leftrightarrow x+2021=0\Leftrightarrow x=-2021\)
\(< =>\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\)
\(< =>\dfrac{x+2+2019}{2019}+\dfrac{x+3+2018}{2018}=\dfrac{x+4+2017}{2017}+\dfrac{x+2021}{2021}\)
\(< =>\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\)
\(< =>\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}=\right)=0\)
\(< =>x+2021=0< =>x=-2021\)
Vậy....
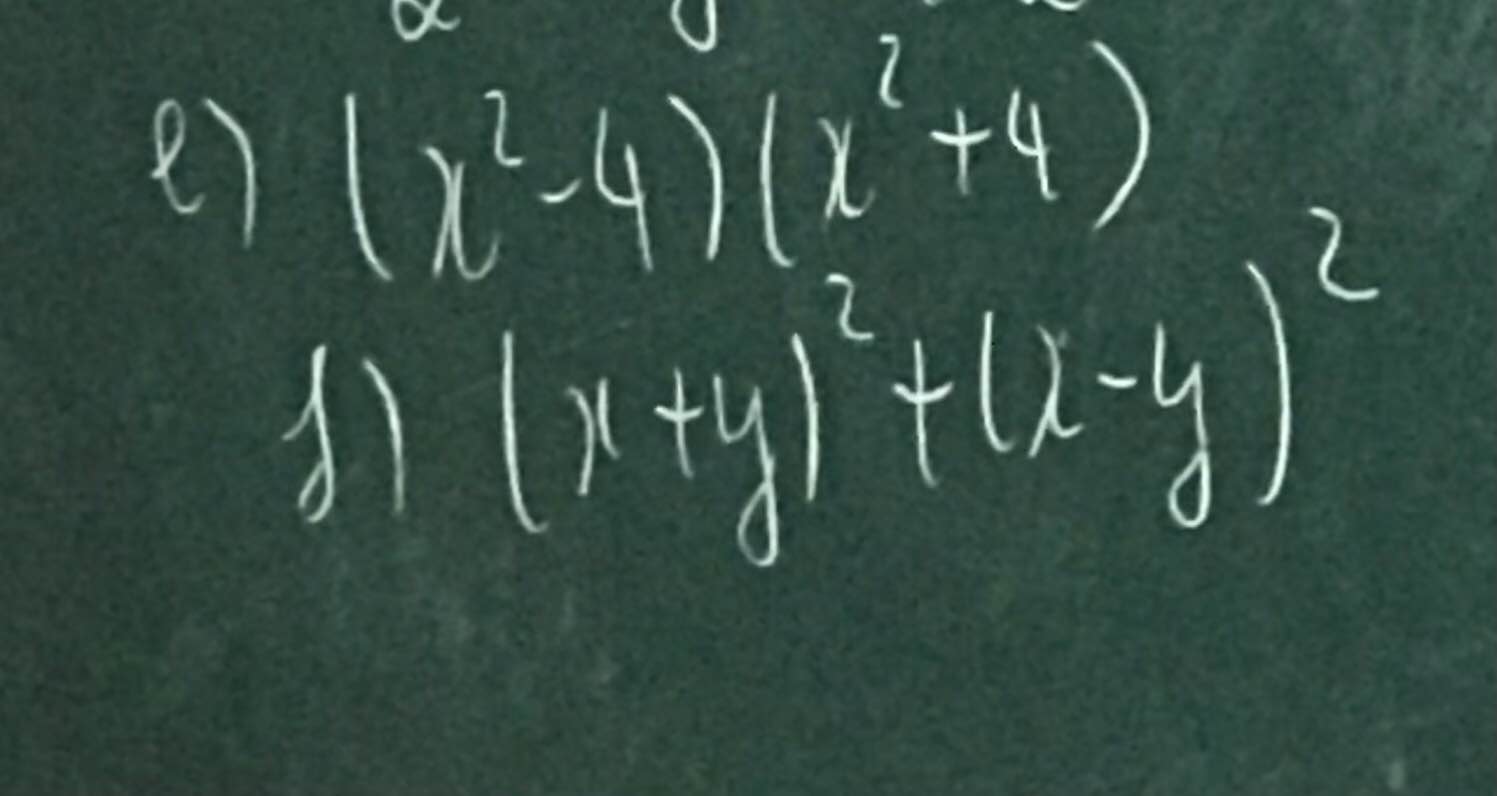
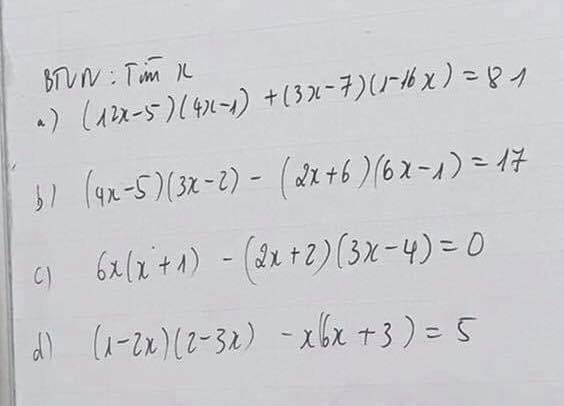


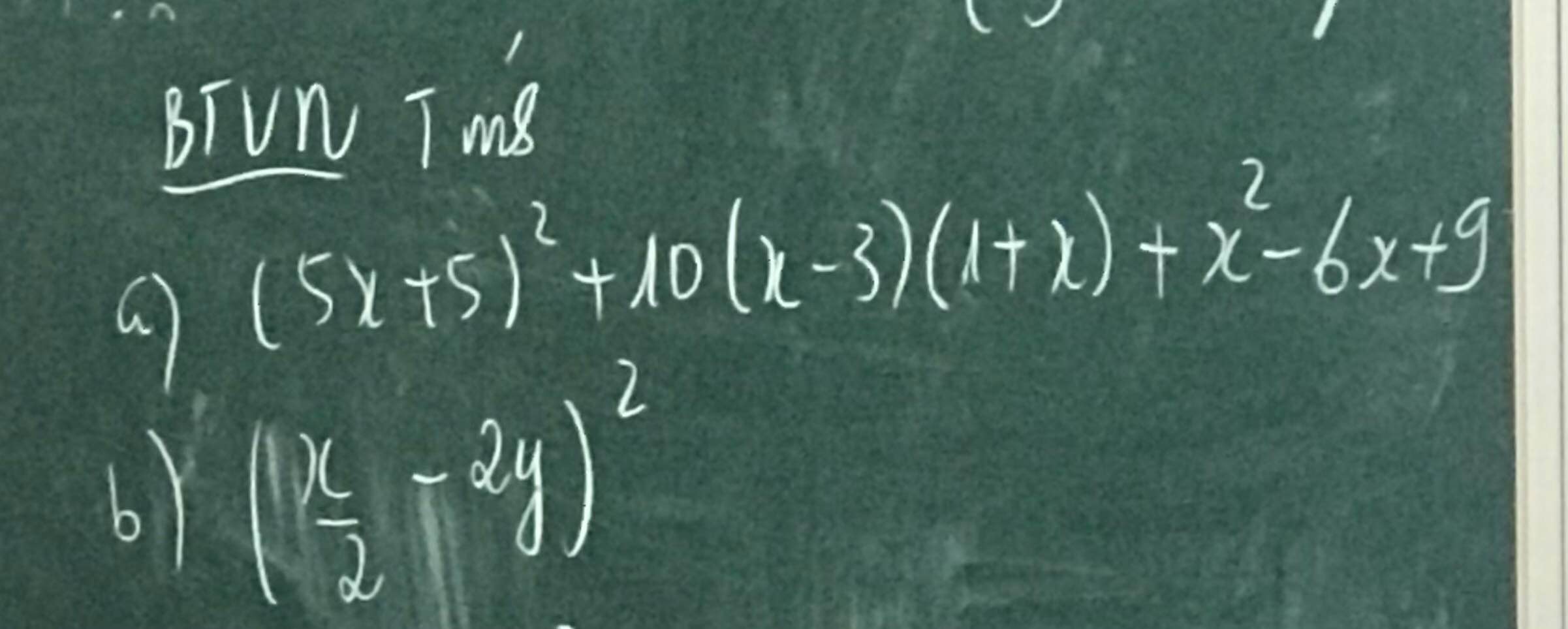
 Giải chi tiết 2 bài với ạ. Cần gấp. Cảm ơn ạ.
Giải chi tiết 2 bài với ạ. Cần gấp. Cảm ơn ạ.


e) \(\left(x^2-4\right)\left(x^2+4\right)=\left(x^2\right)^2-4^2=x^4-16\)
f) \(\left(x+y\right)^2+\left(x-y\right)^2=x^2+2xy+y^2+x^2-2xy+y^2=2x^2+2y^2\)
(x2 - 4)(x2 + 4)
Áp dụng hằng đẳng thức số 3, ta có:
<=> \(\left[\left(x^2\right)^2-4^2\right]\)
<=> (x4 - 16)
Mik làm chi tiết rồi, có gì ko hiểu bảo mik