
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\Leftrightarrow2x^4-2x^3-x^2+1\ge0\)
\(\Leftrightarrow2x^3\left(x-1\right)-\left(x+1\right)\left(x-1\right)\ge0\)
\(\Leftrightarrow\left(x-1\right)\left(2x^3-x-1\right)\ge0\)
Tớ làm tới đây rùi, bạn tự làm tiếp nha

\(a,P=5x\left(2-x\right)-\left(x+1\right)\left(x+9\right)\)
\(=10x-5x^2-\left(x^2+x+9x+9\right)\)
\(=10x-5x^2-x^2-x-9x-9\)
\(=\left(10x-x-9x\right)+\left(-5x^2-x^2\right)-9\)
\(=-6x^2-9\)
Ta thấy: \(x^2\ge0\forall x\)
\(\Rightarrow-6x^2\le0\forall x\)
\(\Rightarrow-6x^2-9\le-9< 0\forall x\)
hay \(P\) luôn nhận giá trị âm với mọi giá trị của biến \(x\).
\(b,Q=3x^2+x\left(x-4y\right)-2x\left(6-2y\right)+12x+1\)
\(=3x^2+x^2-4xy-12x+4xy+12x+1\)
\(=\left(3x^2+x^2\right)+\left(-4xy+4xy\right)+\left(-12x+12x\right)+1\)
\(=4x^2+1\)
Ta thấy: \(x^2\ge0\forall x\)
\(\Rightarrow4x^2\ge0\forall x\)
\(\Rightarrow4x^2+1\ge1>0\forall x\)
hay \(Q\) luôn nhận giá trị dương với mọi giá trị của biến \(x\) và \(y\).
#\(Toru\)

Ta có: \(P=\frac{x^4+x^3+x+1}{x^4-x^3+2x^2-x+1}=\frac{x^3\left(x+1\right)+\left(x+1\right)}{x^4-x^3+x^2+x^2-x+1}=\frac{\left(x^3+1\right)\left(x+1\right)}{x^2\left(x^2-x+1\right)+\left(x^2-x+1\right)}\)
\(=\frac{\left(x+1\right)\left(x^2-x+1\right)\left(x+1\right)}{\left(x^2-x+1\right)\left(x^2+1\right)}=\frac{\left(x+1\right)^2\left(x^2-x+1\right)}{\left(x^2+1\right)\left(x^2-x+1\right)}\)
Vì \(\hept{\begin{cases}x^2+1\ge1>0\\x^2-x+1=\left(x-\frac{1}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}>0\end{cases}}\)
Nên mẫu số luôn luôn khác 0
Do đó: \(P=\frac{\left(x+1\right)^2\left(x^2-x+1\right)}{\left(x^2+1\right)\left(x^2-x+1\right)}=\frac{\left(x+1\right)^2}{x^2+1}\)
Vì \(\hept{\begin{cases}\left(x+1\right)^2\ge0\\x^2+1>0\end{cases}\left(\forall x\right)}\) nên \(P\ge0\left(\forall x\right)\)
\(P=\frac{x^4+x^2+x+1}{x^4-x^2+2x^2-x+1}=\frac{\left(x+1\right)^2\left(x^2-x+1\right)}{\left(x^2+1\right)\left(x^2-x+1\right)}\)
Do \(\left(x^2+1\right)\left(x^2-x+1\right)\ne0\)do đó không cần điều kiện của x
Vậy \(P=\frac{\left(x+1\right)^2\left(x^2-x+1\right)}{\left(x^2+1\right)\left(x^2-x+1\right)}=\frac{\left(x+1\right)^2}{x^2+1}\)
\(\hept{\begin{cases}\left(x+1\right)^2\ge0\forall x\\x^2+1>0\forall x\end{cases}\Rightarrow P\ge0\forall x}\)

a)2x(2x+7)=4(2x+7)
2x(2x+7)-4(2x+7)=0
(2x+7)(2x-4)=0
\(\Rightarrow\orbr{\begin{cases}2x+7=0\\2x-4=0\end{cases}}\)\(\Rightarrow\orbr{\begin{cases}x=-\frac{7}{2}\\x=2\end{cases}}\)
b)Ta có:x3-4x2+ax=x3-3x2-x2+ax
=x2(x-3)-x(x-a)
Để x3-4x2+ax chia hết cho x-3 thì a=3

a) kết quả là x^2-2x+3
b) CM NÈ:
X^2-2X+3=(X^2-2X+1)+2=(X-1)^2+2
VÌ (X-1)^2>=0 VỚI MỌI X=>(X-1)^2+2>0 VỚI MỌI x=> GIÁ TRỊ BIỂU THỨC LUÔN DƯƠNG

Điều kiện x ≠ 1 và x ≠ - 1
Ta có:
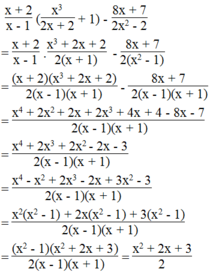
Biểu thức dương khi x 2 + 2 x + 3 > 0
Ta có: x 2 + 2 x + 3 = x 2 + 2 x + 1 + 2 = x + 1 2 + 2 > 0 với mọi giá trị của x.
Vậy giá trị của biểu thức dương với mọi giá trị x ≠ 1 và x ≠ - 1

a)\(A=x^2+x+1=\left(x^2+x+\dfrac{1}{4}\right)+\dfrac{3}{4}=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}>0\)
b) \(B=2x^2+2x+1=2\left(x^2+x+\dfrac{1}{4}\right)+\dfrac{1}{2}=2\left(x+\dfrac{1}{2}\right)^2+\dfrac{1}{2}\ge\dfrac{1}{2}>0\)

Đặt \(A=\dfrac{x^2+x+1}{-2x^2+2x-2}\)
\(x^2+x+1=x^2+2\cdot x\cdot\dfrac{1}{2}+\dfrac{1}{4}+\dfrac{3}{4}=\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}>=\dfrac{3}{4}>0\forall x\)
\(-2x^2+2x-2\)
\(=-2\left(x^2-x+1\right)\)
\(=-2\left(x^2-x+\dfrac{1}{4}+\dfrac{3}{4}\right)\)
\(=-2\left[\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\right]\)
\(=-2\left(x-\dfrac{1}{2}\right)^2-\dfrac{3}{2}< =-\dfrac{3}{2}< 0\forall x\)
Do đó: \(A=\dfrac{x^2+x+1}{-2x^2+2x-2}< 0\forall x\)
\(\dfrac{x^2+x+1}{-2x^2+2x-2}=\dfrac{x^2+x+1}{-2\left(x^2-x+1\right)}\)
Ta thấy:
\(x^2+x+1\\=x^2+2\cdot x\cdot\dfrac12+\left(\dfrac12\right)^2-\left(\dfrac12\right)^2+1\\=\left(x+\dfrac12\right)^2+\dfrac34\)
Vì \(\left(x+\dfrac{1}{2}\right)^2\ge0\forall x\)
\(\Rightarrow\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}>0\forall x\)
hay \(x^2+x+1>0\forall x\) (1)
Lại có:
\(x^2-x+1\\=x^2-2\cdot x\cdot\dfrac12+\left(\dfrac12\right)^2-\left(\dfrac12\right)^2+1\\=\left(x-\dfrac12\right)^2+\dfrac34\)
Vì \(\left(x-\dfrac{1}{2}\right)^2\ge0\forall x\)
\(\Rightarrow\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}>0\forall x\)
hay \(x^2-x+1>0\forall x\) (2)
Từ (1) và (2) \(\Rightarrow\dfrac{x^2+x+1}{x^2-x+1}>0\forall x\)
\(\Rightarrow\dfrac{x^2+x+1}{-2\left(x^2-x+1\right)}< 0\forall x\)
hay đa thức \(\dfrac{x^2+x+1}{-2x^2+2x-2}< 0\forall x\)
\(\text{#}Toru\)
Ta có :
2x4 + 1 - 2x3 - x2
= 2x3 ( x - 1 ) - ( x - 1 ) ( x + 1 )
= ( x - 1 ) ( 2x3 - x - 1 )
= ( x - 1 ) [ ( x3 - x ) + ( x3 - 1 ) ]
= ( x - 1 ) [ x ( x - 1 ) ( x + 1 ) + ( x - 1 ) ( x2 + x + 1 ) ]
= ( x - 1 )2 ( x2 + x + x2 + x + 1 )
= ( x - 1 )2 ( 2x2 + 2x + 1 )
= ( x - 1 )2 ( x2 + ( x + 1 )2 ) \(\ge\)0
Suy ra đpcm