Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


viết lại câu hỏi khác đi, đề không rõ ràng X với x rồi . lung tung, dung công cụ soạn thảo đi nha bạn

a, Thay m=0 vào pt ta có:
\(x^2-x+1=0\)
\(\Rightarrow\) pt vô nghiệm
b, Để pt có 2 nghiệm thì \(\Delta\ge0\)
\(\Leftrightarrow\left(-1\right)^2-4.1\left(m+1\right)\ge0\\ \Leftrightarrow1-4m-4\ge0\\ \Leftrightarrow-3-4m\ge0\\ \Leftrightarrow4m+3\le0\\ \Leftrightarrow m\le-\dfrac{3}{4}\)
Theo Vi-ét:\(\left\{{}\begin{matrix}x_1+x_2=1\\x_1x_2=m+1\end{matrix}\right.\)
\(x_1x_2\left(x_1x_2-2\right)=3\left(x_1+x_2\right)\\ \Leftrightarrow\left(x_1x_2\right)^2-2x_1x_2=3.1\\ \Leftrightarrow\left(m+1\right)^2-2\left(m+1\right)-3=0\\ \Leftrightarrow\left[{}\begin{matrix}m+1=3\\m+1=-1\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}m=2\left(ktm\right)\\m=-2\left(tm\right)\end{matrix}\right.\)

Δ=(-3)^2-4m^2=9-4m^2
Để phương trình có hai nghiệm thì 9-4m^2>=0
=>-2/3<=m<=2/3
x1^2-3x2+x1x2-m^2-2m-1>6-m^2
=>x1^2-x2(x1+x2)+x1x2>6-m^2+m^2+2m+1=2m+7
=>x1^2-x2^2>2m+7
=>(x1+x2)(x1-x2)>2m+7
=>(x1-x2)*3>2m+7
=>x1-x2>2/3m+7/3
\(\left(x_1-x_2\right)^2=\left(x_1+x_2\right)^2-4x_1x_2=3^2-4m^2=9-4m^2\)
=>\(x1-x2=\left|9-4m^2\right|\)
=>|9-4m^2|>2/3m+7/3
=>|4m^2-9|>2/3m+7/3
=>4m^2-9<-2/3m-7/3 hoặc 4m^2-9>2/3m+7/3
=>4m^2+2/3m-20/3<0 hoặc 4m^2-2/3m-34/3>0
=>\(\dfrac{-1-\sqrt{241}}{12}< m< \dfrac{-1+\sqrt{241}}{12}\) hoặc \(\left[{}\begin{matrix}m< \dfrac{1-\sqrt{409}}{12}\\m>\dfrac{1+\sqrt{409}}{12}\end{matrix}\right.\)
=>-2/3<=m<=2/3

Em ko ghi đc dấu căn nên em đóng ngoặc nghĩa là cả cụm đó dưới dấu căn

a: \(\Delta=\left(2m+2\right)^2-4\left(m^2-2m-3\right)\)
\(=4m^2+8m+4-4m^2+8m+12\)
=16m+16
Để phương trình luôn có nghiệm thì 16m+16>=0
hay m>=-1
b: Theo đề, ta có: \(\left(x_1+x_2\right)^2-2x_1x_2-x_1x_2=28\)
\(\Leftrightarrow\left(2m+2\right)^2-3\left(m^2-2m-3\right)=28\)
\(\Leftrightarrow4m^2+8m+4-3m^2+6m+9=28\)
\(\Leftrightarrow m^2+14m-15=0\)
=>(m+15)(m-1)=0
=>m=1
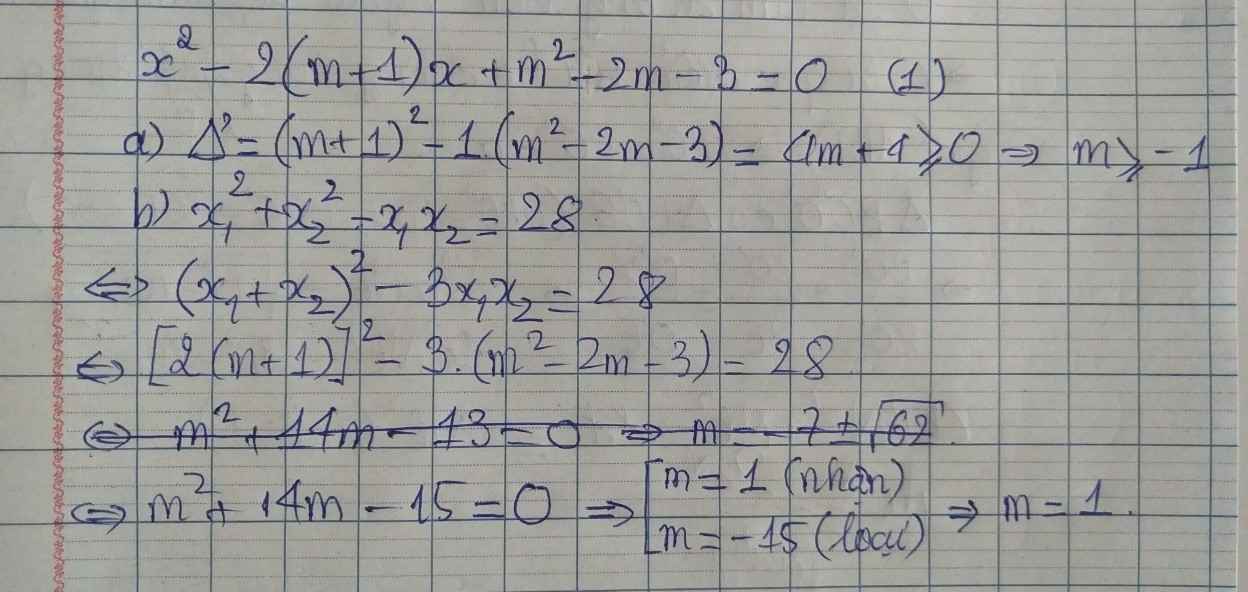
-pt:\(x^2-3x+2m-1=0\) (1)
-để pt(1) có 2 nghiệm:=)\(\Delta=\left(-3\right)^2-4\cdot\left(2m-1\right)\ge0\)
<=>\(9-8m+4\ge0\)
<=>\(-8m\ge-13\)
<=>\(m\le\frac{13}{8}\)
---theo vi-ét ta có:
\(x_1+x_2=3\)
\(x_1\cdot x_2=2m-1\)