Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

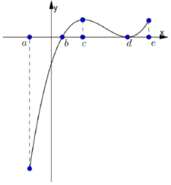
Ta có bảng biến thiên như hình vẽ sau:
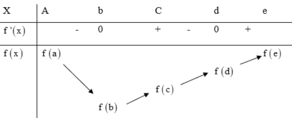
Giá trị nhỏ nhất của hàm số là f( b) nhưng giá trị lớn nhất có thể là f (a) hoặc f( e) Theo giả thiết ta có: f(a) + f( c)) = f( b) + f( d) nên f(a) - f( d)) = f( b) - f( c)< 0
Suy ra : f( a) < f( d) < f( e)
Vậy m a x [ a ; e ] f ( x ) = f ( e ) ; m i n [ a ; e ] f ( x ) = f ( b )
Chọn C.

\(F\left(x\right)=\int\left(e^x.ln\left(ax\right)+\dfrac{e^x}{x}\right)dx=\int e^xln\left(ax\right)dx+\int\dfrac{e^x}{x}dx=\int e^xlnxdx+\int\dfrac{e^x}{x}dx+\int e^x.lna.dx\)
Xét \(I=\int e^xlnxdx\)
Đặt \(\left\{{}\begin{matrix}u=lnx\\dv=e^xdx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=\dfrac{dx}{x}\\v=e^x\end{matrix}\right.\)
\(\Rightarrow I=lnx.e^x-\int\dfrac{e^x}{x}dx\)
\(\Rightarrow F\left(x\right)=e^x.lnx+e^x.lna+C\)
\(F\left(\dfrac{1}{a}\right)=e^{\dfrac{1}{a}}ln\left(\dfrac{1}{a}\right)+e^{\dfrac{1}{a}}.lna+C=0\Rightarrow C=0\)
\(F\left(2020\right)=e^{2020}ln\left(2020\right)+e^{2020}.lna=e^{2020}\)
\(\Rightarrow ln\left(2020a\right)=1\Rightarrow a=\dfrac{e}{2020}\)

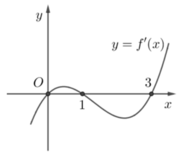
\(f'\left(x\right)=0\) có 3 nghiệm \(x=-1;0;2\)
Dấu của \(f'\left(x\right)\) trên trục số:
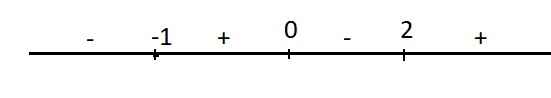
Ta thấy có 2 lần \(f'\left(x\right)\) đổi dấu từ âm sang dương nên hàm có 2 cực tiểu
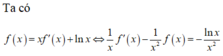
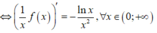
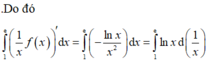
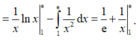

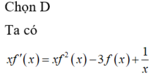
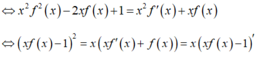
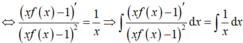
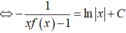
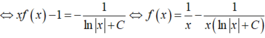
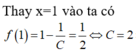

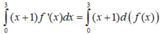
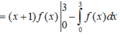
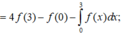
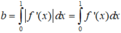
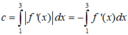
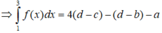
Đáp án A