Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Khi O nằm ngoài đoạn AB thì hai vec tơ và
cùng hướng và góc
(,
) = 0
cos(,
) = 1 nên
.
= a.b
![]()
b) Khi O nằm ngoài trongđoạn AB thì hai vectơ và
ngược hướng và góc
(,
) = 1800
cos(,
) = -1 nên
.
= -a.b
![]()

A B C D I K
a)
- \(\overrightarrow{BI}=\frac{1}{2}\left(\overrightarrow{BA}+\overrightarrow{BD}\right)\) (t/c trung điểm)
\(=\frac{1}{2}\left(\overrightarrow{BA}+\frac{1}{2}\overrightarrow{BC}\right)\)
\(=\frac{1}{2}\overrightarrow{BA}+\frac{1}{4}\overrightarrow{BC}\)
- \(\overrightarrow{BK}=\overrightarrow{BA}+\overrightarrow{AK}\)
\(=\overrightarrow{BA}+\frac{1}{3}\overrightarrow{AC}\)
\(=\overrightarrow{BA}+\frac{1}{3}\left(\overrightarrow{BC}-\overrightarrow{BA}\right)\)
\(=\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}-\frac{1}{3}\overrightarrow{BA}\)
\(=\frac{2}{3}\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}\)
b) Ta có: \(\overrightarrow{BK}=\frac{2}{3}\overrightarrow{BA}+\frac{1}{3}\overrightarrow{BC}=\frac{4}{3}\left(\frac{1}{2}\overrightarrow{BA}+\frac{1}{4}\overrightarrow{BC}\right)=\frac{4}{3}\overrightarrow{BI}\)
=> B,K,I thẳng hàng
c) \(27\overrightarrow{MA}-8\overrightarrow{MB}=2015\overrightarrow{MC}\)
\(\Leftrightarrow27\left(\overrightarrow{MC}+\overrightarrow{CA}\right)-8\left(\overrightarrow{MC}+\overrightarrow{CB}\right)=2015\overrightarrow{MC}\)
\(\Leftrightarrow27\overrightarrow{MC}+27\overrightarrow{CA}-8\overrightarrow{MC}-8\overrightarrow{CB}-2015\overrightarrow{MC}=\overrightarrow{0}\)
\(\Leftrightarrow-1996\overrightarrow{MC}+27\overrightarrow{CA}-8\overrightarrow{CB}=\overrightarrow{0}\)
\(\Leftrightarrow1996\overrightarrow{CM}=8\overrightarrow{CB}-27\overrightarrow{CA}\)
\(\Leftrightarrow\overrightarrow{CM}=\frac{8\overrightarrow{CB}-27\overrightarrow{CA}}{1996}\)
Vậy: Dựng điểm M sao cho \(\overrightarrow{CM}=\frac{8\overrightarrow{CB}-27\overrightarrow{CA}}{1996}\)

Do \(\overrightarrow{a}+\overrightarrow{b}=\overrightarrow{0}\) nên hai véc tơ \(\overrightarrow{a}\) và \(\overrightarrow{b}\) đối nhau.
a)
\(\overrightarrow{OA}+\overrightarrow{OB}=\overrightarrow{a}+\overrightarrow{b}=\overrightarrow{0}\) nên O là trung điểm của AB.
b) \(\overrightarrow{OB}=\overrightarrow{OA}+\overrightarrow{AB}=\overrightarrow{a}+\overrightarrow{b}=\overrightarrow{0}\) nên \(O\equiv B\).

Gọi M là trung điểm của AB
Xét ΔOAB có OM là đường trung tuyến
nên \(\overrightarrow{OA}+\overrightarrow{OB}=2\cdot\overrightarrow{OM}\)
=>Giá của vecto OA+vecto OB là đường thẳng OM
Để OM là phân giác của góc AOB thì OM vừa là đường trung tuyến vừa là đường phân giác của ΔOAB
=>ΔOAB cân tại O
=>OA=OB

Lời giải:
a) Bạn tham khảo tại đây:
Câu hỏi của Trần Thị Như Ý - Toán lớp 10 | Học trực tuyến
b)
\(|\overrightarrow{OB}+\overrightarrow{AC}-\overrightarrow{OA}|=|\overrightarrow{OB}+\overrightarrow{AO}+\overrightarrow{OC}-\overrightarrow{OA}|\)
\(=|\overrightarrow{OB}+\overrightarrow{OC}-2\overrightarrow{OA}|\)
\(=|-\overrightarrow{OA}-2\overrightarrow{OA}|=3|\overrightarrow{OA}|=3a\)

a: vecto CO
b: vecto OA+vecto OB
=vecto CO+vecto OB
=vecto CB
c: vecto OA+vecto OC+vecto OB+vecto OD
=vecto 0+vecto 0
=vecto 0
d: vecto đối là vecto BA

Ta có: \(\overrightarrow{MB}=3\overrightarrow{MC}\Rightarrow\overrightarrow{MB}=3\left(\overrightarrow{MB}+\overrightarrow{BC}\right)\)
\(\Rightarrow\overrightarrow{MB}=3\overrightarrow{MB}+3\overrightarrow{BC}\)
\(\Rightarrow-\overrightarrow{MB}=3\overrightarrow{BC}\)
\(\Rightarrow\overrightarrow{BM}=\dfrac{2}{3}\overrightarrow{BC}\). Mà \(\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}\) nên \(\overrightarrow{BM}=\dfrac{2}{3}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)\)
Theo quy tắc 3 điểm, ta có
\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}\Rightarrow\overrightarrow{AM}=\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}-\dfrac{3}{2}\overrightarrow{AB}\)
\(\Rightarrow\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{AB}+\dfrac{3}{2}\overrightarrow{AC}\) hay \(\overrightarrow{AM}=-\dfrac{1}{2}\overrightarrow{u}+\dfrac{3}{2}\overrightarrow{v}\)

câu 2 ( các kí hiệu vecto khi lm bài thỳ b tự viết nhé mk k viết kí hiệu để trả lời cho nhanh hỳ hỳ )
OA+ OB + OC = OA'+ OB' + OC'
<=> OA - OA' + OB - OB' + OC - OC' = 0
<=> A'A + B'B + C'C = 0
<=> 2 ( BA + CB + AC ) = 0
<=> 2 ( CB + BA + AC ) = 0
<=> 2 ( CA + AC ) = 0
<=> 0 = 0 ( luôn đúng )
câu 1 ( các kí hiệu vecto b cx tự viết nhá )
VT = OD + OC = OA + AD + OB + BC = OA + OB + AD + BC = BO + OB + AD + BC = 0 + AD + BC = AD + BC = VP ( đpcm)

 = 3
= 3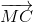 =>
=> 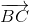 )
)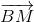 =
= 
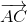 -
- 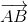 nên
nên 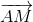 =
= 

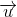 +
+ 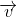
a) Giả sử véc tơ \(\overrightarrow{OA}+\overrightarrow{OB}\) nằm trên đường phân giác góc \(\widehat{AOB}\) .
Dựng hình bình hành OABD.
O A B D
Theo quy tắc hình bình hành: \(\overrightarrow{OA}+\overrightarrow{OB}=\overrightarrow{OD}\).
Theo giả thiết thì OD là tia phân giác góc \(\widehat{AOB}\).
Vì vậy hình bình hành OABD là hình thoi.
Suy ra OA = OB.
- Giả sử OA = OB.
Khi đó hình bình hành OABD có OA = OB nên tứ giác OABD là hình thoi.
Kết luận: Điều kiện cần và đủ để véc tơ \(\overrightarrow{OA}+\overrightarrow{OB}\) nằm trên đường phân giác góc \(\widehat{AOB}\) là OA = OB.