K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
6 tháng 8 2021
a,\(x^2-7x+6=x^2-x-6x+6\)
\(=x\left(x-1\right)-6\left(x-1\right)\)
\(=\left(x-6\right)\left(x-1\right)\)
6 tháng 8 2021
a) x2-7x+6=(x2-x)-(6x-6)=x(x-1)-6(x-1)=(x-6)(x-1)
b) x2-6x+3=(x2-6x+9)-6=(x-3)2-\(\sqrt{6^2}\)=(x-3-\(\sqrt{6}\))(x-3+\(\sqrt{6}\))
c) x2-4x+3=(x2-x)-(3x-3)=x(x-1)-3(x-1)=(x-3)(x-1)
d) 3x2-5x+2=(3x2-3x)-(2x-2)=3x(x-1)-2(x-1)=(3x-2)(x-1)
e) 7x2-x-6=(7x2-7x)+(6x-6)=7x(x-1)+6(x-1)=(7x+6)(x-1)
f) 3x2-5x-8=(3x2+3x)-(8x+8)=3x(x+1)-8(x+1)=(3x-8)(x+1)
g) x2-6x+5=(x2-x)-(5x-5)=x(x-1)-5(x-1)=(x-5)(x-1)
h) x2-2x-3=(x2-2x+1)-4=(x-1)2-22=(x-1-2)(x-1+2)=(x-3)(x+1)
i) x2-x-12=(x2+3x)-(4x+12)=x(x+3)-4(x+3)=(x-4)(x+3)
3 tháng 10 2021
Bài 5:
Theo đề, ta có:
\(\left(2x+5\right)^2-4x^2-12x=41\)
\(\Leftrightarrow20x-12x=41+25=66\)
hay \(x=8.25\left(m\right)\)
Chu vi là:
\(\left[\left(2\cdot8.25+5\right)^2+\left(4\cdot8.25^2+12\cdot8.25\right)\right]\cdot2=1667\left(m\right)\)
HP
24 tháng 9 2021
Bài 2:
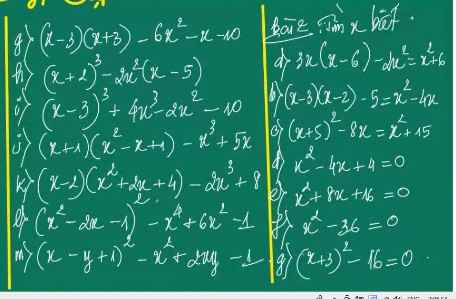
a. 3x(x - 6) - 2x2 = x2 + 6
<=> 3x2 - 18x - 2x2 - x2 - 6 = 0
<=> 3x2 - 2x2 - x2 - 18x - 6 = 0
<=> -18x - 6 = 0
<=> -18x = 6
<=> x = \(\dfrac{6}{-18}=\dfrac{-1}{3}\)
b. (x - 3)(x - 2) - 5 = x2 - 4x
<=> x2 - 2x - 3x + 6 - 5 - x2 + 4x = 0
<=> x2 - x2 - 2x - 3x + 4x + 6 - 5 = 0
<=> -x + 1 = 0
<=> -x = -1
<=> x = 1
c. (x + 5)2 - 8x = x2 + 15
<=> x2 + 10x + 25 - 8x - x2 - 15 = 0
<=> x2 - x2 + 10x - 8x + 25 - 15 = 0
<=> 2x + 10 = 0
<=> 2x = -10
<=> x = -5
d. x2 - 4x + 4 = 0
<=> x2 - 2.2.x + 22 = 0
<=> (x - 2)2 = 0
<=> x - 2 = 0
<=> x = 2
e. x2 + 8x + 16 = 0
<=> x2 + 2.x.4 + 42 = 0
<=> (x + 4)2 = 0
<=> x + 4 = 0
<=> x = -4
f. x2 - 36 = 0
<=> x2 - 62 = 0
<=> (x - 6)(x + 6) = 0
<=> \(\left[{}\begin{matrix}x-6-0\\x+6=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=6\\x=-6\end{matrix}\right.\)
g. (x + 3)2 - 16 = 0
<=> (x + 3)2 - 42 = 0
<=> (x + 3 + 4)(x + 3 - 4) = 0
<=> (x + 7)(x - 1) = 0
<=> \(\left[{}\begin{matrix}x+7=0\\x-1=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=-7\\x=1\end{matrix}\right.\)
24 tháng 9 2021
k: Ta có: \(\left(x-2\right)\left(x^2+2x+4\right)-2x^3+8\)
\(=x^3-8-2x^3+8\)
\(=-x^3\)
J
3
Y
28 tháng 6 2023
\(a,A=0,2\left(5x-1\right)-\dfrac{1}{2}\left(\dfrac{2}{3}x+4\right)+\dfrac{2}{3}\left(3-x\right)\)
\(=x-0,2-\dfrac{1}{3}x-2+2-\dfrac{2}{3}x\)
\(=\left(-0,2-2+2\right)+\left(x-\dfrac{1}{3}x-\dfrac{2}{3}x\right)\)
\(=-0,2\)
\(b,B=\left(x-2y\right)\left(x^2+2xy+4y^2\right)-\left(x^3-8y^3+10\right)\)
\(=x^3-8y^3-x^3+8y^3-10\)
\(=-10\)
\(c,C=4\left(x+1\right)^2+\left(2x-1\right)^2-8\left(x-1\right)\left(x+1\right)-4x\)
\(=4\left(x^2+2x+1\right)+\left(4x^2-4x+1\right)-8\left(x^2-1\right)-4x\)
\(=4x^2+8x+4+4x^2-4x+1-8x^2+8-4x\)
\(=13\)
H9
HT.Phong (9A5)
CTVHS
28 tháng 6 2023
a) \(A=0,2\left(5x-1\right)-\dfrac{1}{2}\left(\dfrac{2}{3}x+4\right)+\dfrac{2}{3}\left(3-x\right)\)
\(A=x-\dfrac{1}{5}-\dfrac{1}{3}x-2+2-\dfrac{2}{3}x\)
\(A=\left(x-\dfrac{1}{3}x-\dfrac{2}{3}x\right)-\left(\dfrac{1}{5}+2-2\right)\)
\(A=-\dfrac{1}{5}\)
Vậy: ...
b) \(B=\left(x-2y\right)\left(x^2+2xy+4y^2\right)-\left(x^3-8y^3+10\right)\)
\(B=\left[x^3-\left(2y\right)^3\right]-\left[x^3-\left(2y\right)^3\right]-10\)
\(B=-10\)
Vậy: ...
c) \(4\left(x+1\right)^2+\left(2x-1\right)^2-8\left(x+1\right)\left(x-1\right)-4x\)
\(=4\left(x^2+2x+4\right)+\left(4x^2-4x+1\right)-8\left(x^2-1\right)-4x\)
\(=4x^2+8x+4+4x^2-4x+1-8x^2+8-4x\)
\(=\left(4x^2+4x^2-8x^2\right)+\left(8x-4x-4x\right)+\left(4+1+8\right)\)
\(=13\)
Vậy:...
N
1
21 tháng 6 2021
\(\Leftrightarrow2\left(x+1\right)^3=56\Leftrightarrow\left(x+1\right)^3=28\Leftrightarrow\)
MA
0
15 tháng 11 2021
\(a,=x^2+x+4x+4=\left(x+1\right)\left(x+4\right)\\ b,=x^2+2x-3x-6=\left(x-3\right)\left(x+2\right)\\ c,=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\\ d,=3\left(x^2-2x+5x-10\right)=3\left(x-2\right)\left(x+5\right)\\ e,=-3x^2+6x-x+2=\left(x-2\right)\left(1-3x\right)\\ f,=x^2-x-6x+6=\left(x-1\right)\left(x-6\right)\\ h,=4\left(x^2-3x-6x+18\right)=4\left(x-3\right)\left(x-6\right)\\ i,=3\left(3x^2-3x-8x+5\right)=3\left(x-1\right)\left(3x-8\right)\\ k,=-\left(2x^2+x+4x+2\right)=-\left(2x+1\right)\left(x+2\right)\\ l,=x^2-2xy-5xy+10y^2=\left(x-2y\right)\left(x-5y\right)\\ m,=x^2-xy-2xy+2y^2=\left(x-y\right)\left(x-2y\right)\\ n,=x^2+xy-3xy-3y^2=\left(x+y\right)\left(x-3y\right)\)
29 tháng 5 2022
Câu 1: A
Câu 2: B
Câu 3: D
Câu 4: A
Câu 5: C
Câu 6: B
Câu 7: A
Câu 9: B
a: Xét tứ giác AEHF có
\(\widehat{AEH}=\widehat{AFH}=\widehat{FAE}=90^0\)
Do đó: AEHF là hình chữ nhật