Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x\notin\left\{1;\dfrac{25}{9};\dfrac{9}{4}\right\}\end{matrix}\right.\)
a: \(C=\left(\dfrac{2\sqrt{x}}{\left(\sqrt{x}-1\right)\left(2\sqrt{x}-3\right)}-\dfrac{5}{2\sqrt{x}-3}\right):\left(3-\dfrac{2}{\sqrt{x}-1}\right)\)
\(=\dfrac{2\sqrt{x}-5\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(2\sqrt{x}-3\right)}:\dfrac{3\sqrt{x}-3-2}{\sqrt{x}-1}\)
\(=\dfrac{2\sqrt{x}-5\sqrt{x}+5}{\left(\sqrt{x}-1\right)\left(2\sqrt{x}-3\right)}\cdot\dfrac{\sqrt{x}-1}{3\sqrt{x}-5}\)
\(=-\dfrac{1}{2\sqrt{x}-3}\)
b: \(x=\dfrac{2}{2-\sqrt{3}}=2\left(2+\sqrt{3}\right)=4+2\sqrt{3}\)
Khi \(x=4+2\sqrt{3}\) thì \(C=-\dfrac{1}{2\left(\sqrt{3}+1\right)-3}=\dfrac{-1}{2\sqrt{3}-1}=\dfrac{-2\sqrt{3}-1}{11}\)
c: C=-1
=>\(2\sqrt{x}-3=1\)
=>\(\sqrt{x}=2\)
=>x=4(nhận)
d: C>0
=>\(2\sqrt{x}-3< 0\)
=>\(\sqrt{x}< \dfrac{3}{2}\)
=>\(0< =x< \dfrac{9}{4}\)
Kết hợp ĐKXĐ, ta được: \(\left\{{}\begin{matrix}0< =x< \dfrac{9}{4}\\x< >1\end{matrix}\right.\)

\(\left(\sqrt{a}+\sqrt{b}+\sqrt{c}\right)^2=a+b+c+2\left(\sqrt{ab}+\sqrt{ac}+\sqrt{bc}\right)=4\)
\(\Leftrightarrow\sqrt{ab}+\sqrt{ac}+\sqrt{bc}=1\)
\(\Rightarrow a+1=a+\sqrt{ab}+\sqrt{ac}+\sqrt{bc}=\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)\)
Tương tự: \(b+1=\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{b}+\sqrt{c}\right)\)
\(c+1=\left(\sqrt{a}+\sqrt{c}\right)\left(\sqrt{b}+\sqrt{c}\right)\)
\(VT=\sum\dfrac{\sqrt{a}}{a+1}=\sum\dfrac{\sqrt{a}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)}\)
\(=\dfrac{\sqrt{a}\left(\sqrt{b}+\sqrt{c}\right)+\sqrt{b}\left(\sqrt{a}+\sqrt{c}\right)+\sqrt{c}\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)\left(\sqrt{b}+\sqrt{c}\right)}\)
\(=\dfrac{2\left(\sqrt{ab}+\sqrt{ac}+\sqrt{bc}\right)}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)\left(\sqrt{b}+\sqrt{c}\right)}=\dfrac{2}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)\left(\sqrt{b}+\sqrt{c}\right)}\)
\(VP=\dfrac{2}{\sqrt{\left(a+1\right)\left(b+1\right)\left(c+1\right)}}=\dfrac{2}{\sqrt{\left(\sqrt{a}+\sqrt{b}\right)^2\left(\sqrt{a}+\sqrt{c}\right)^2\left(\sqrt{b}+\sqrt{c}\right)^2}}\)
\(=\dfrac{2}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}+\sqrt{c}\right)\left(\sqrt{b}+\sqrt{c}\right)}\)
\(\Rightarrow VT=VP\) (đpcm)

a/ Nếu (a + b) < 0 thì bất đẳng thức đúng
Với (a + b) \(\ge0\)thì ta có
\(2a^2+ab+2b^2\ge\frac{5}{4}\left(a^2+2ab+b^2\right)\)
\(\Leftrightarrow3a^2-6ab+3b^2\ge0\)
\(\Leftrightarrow3\left(a-b\right)^2\ge0\)(đúng)
b/ Áp dụng BĐT BCS :
\(1=\left(1.\sqrt{a}+1.\sqrt{b}+1.\sqrt{c}\right)^2\le3\left(a+b+c\right)\Rightarrow a+b+c\ge\frac{1}{3}\)
Áp dụng câu a/ :
\(\sqrt{2a^2+ab+2b^2}\ge\frac{\sqrt{5}}{2}\left(a+b\right)\)
\(\sqrt{2b^2+bc+2c^2}\ge\frac{\sqrt{5}}{2}\left(b+c\right)\)
\(\sqrt{2c^2+ac+2a^2}\ge\frac{\sqrt{5}}{2}\left(a+c\right)\)
\(\Rightarrow P\ge\frac{\sqrt{5}}{2}.2\left(a+b+c\right)\ge\frac{\sqrt{5}}{3}\)
Đẳng thức xảy ra khi \(a=b=c=\frac{1}{9}\)
Vậy min P = \(\frac{\sqrt{5}}{3}\) khi a=b=c=1/9

a: \(=\dfrac{\sqrt{m}\left(m+4n-4\sqrt{mn}\right)}{\sqrt{mn}\left(\sqrt{m}-2\sqrt{n}\right)}\)
\(=\dfrac{1}{\sqrt{n}}\cdot\left(\sqrt{m}-2\sqrt{n}\right)\)
b: \(=\dfrac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}+1}\)
c: \(=\sqrt{5^2\cdot2\cdot x^2y^4\cdot xy}-\dfrac{2y^2}{x^2}\cdot4\sqrt{2}\cdot x^3\sqrt{xy}+\dfrac{3}{2}xy\cdot\sqrt{2}\cdot y\cdot\sqrt{xy}\)
\(=5xy^2\sqrt{2xy}-8\sqrt{2xy}xy^2+\dfrac{3}{2}xy^2\cdot\sqrt{2xy}\)
\(=-\dfrac{3}{2}\sqrt{2xy}\)
d: \(=\left(x+2\right)\cdot\dfrac{\sqrt{2x-3}}{\sqrt{x+2}}=\sqrt{\left(2x-3\right)\left(x+2\right)}\)

Lời giải:
\(a+b+c=\sqrt{a}+\sqrt{b}+\sqrt{c}=2\)
\(\Rightarrow (\sqrt{a}+\sqrt{b}+\sqrt{c})^2=4\)
\(\Leftrightarrow a+b+c+2(\sqrt{ab}+\sqrt{bc}+\sqrt{ac})=4\)
\(\Leftrightarrow \sqrt{ab}+\sqrt{bc}+\sqrt{ac}=\frac{4-(a+b+c)}{2}=1\)
\(\Rightarrow a+1=a+\sqrt{ab}+\sqrt{bc}+\sqrt{ac}=(\sqrt{a}+\sqrt{b})(\sqrt{a}+\sqrt{c})\)
Tương tự:
$b+1=(\sqrt{b}+\sqrt{c})(\sqrt{c}+\sqrt{a})$
$c+1=(\sqrt{c}+\sqrt{a})(\sqrt{c}+\sqrt{b})$
Khi đó:
\(A=\left[\frac{\sqrt{a}}{(\sqrt{a}+\sqrt{b})(\sqrt{a}+\sqrt{c})}+\frac{\sqrt{b}}{(\sqrt{b}+\sqrt{a})(\sqrt{b}+\sqrt{c})}+\frac{\sqrt{c}}{(\sqrt{c}+\sqrt{a})(\sqrt{c}+\sqrt{b})}\right]\sqrt{(a+1)(b+1)(c+1)}\)
\(\frac{\sqrt{a}(\sqrt{b}+\sqrt{c})+\sqrt{b}(\sqrt{c}+\sqrt{a})+\sqrt{c}(\sqrt{a}+\sqrt{b})}{(\sqrt{a}+\sqrt{b})(\sqrt{b}+\sqrt{c})(\sqrt{c}+\sqrt{a})}.\sqrt{(\sqrt{a}+\sqrt{b})^2(\sqrt{b}+\sqrt{c})^2(\sqrt{c}+\sqrt{a})^2}\)
\(=\frac{2(\sqrt{ab}+\sqrt{bc}+\sqrt{ca})}{(\sqrt{a}+\sqrt{b})(\sqrt{b}+\sqrt{c})(\sqrt{c}+\sqrt{a})}.(\sqrt{a}+\sqrt{b})(\sqrt{b}+\sqrt{c})(\sqrt{c}+\sqrt{a})\)
\(=2(\sqrt{ab}+\sqrt{bc}+\sqrt{ac})=2\)

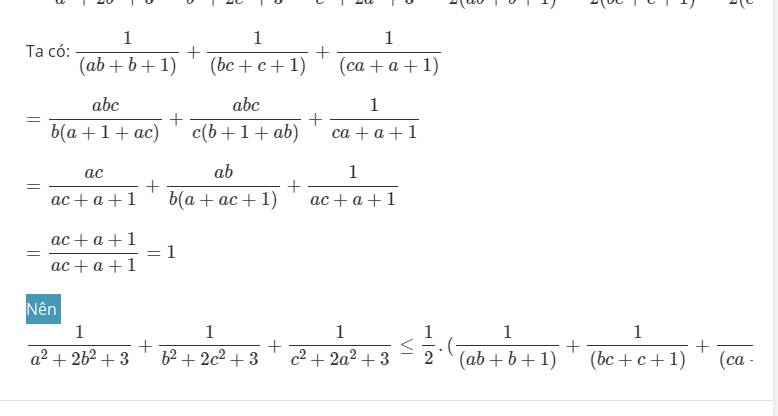


a. M= √a .√b= √a.b= √2.8= 4
b. N= √c2 -1/c= √(√5 -2)2 -1/(√5 -2)= |√5 -2| -1/(√5 -2)= √5 -2 -1/√5 -2
= (√5 -2)2-1/(√5 -2)= (√5 -3)(√5 -1)/(√5 -2)
gọi t= √5 -2
= (t-1)(t+1)/t= t2-1/t =-1/t
=-1/√5 -2= 2+√5