K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
14 tháng 9 2017
Vậy các đa thức cần điền lần lượt là 4x; x + 2.
Đáp án cần chọn là: A
KT
14 tháng 2 2018
c) \(2xy-x^2-y^2+16\)
\(=16-\left(x^2-2xy+y^2\right)\)
\(=16-\left(x-y\right)^2\)
\(=\left(4-x+y\right)\left(4+x-y\right)\)
SG
14 tháng 2 2018
c ) \(2xy - x^2 - y^2 + 16\)
\(= 16 - ( x^2 - 2xy + y^2 ) \)
\(= 16 - ( x - y ) ^2 \)
\(= ( 4 - x + y )\)
\(( 4 + x - y )\)
AH
Akai Haruma
Giáo viên
8 tháng 12 2021
Lời giải:
$2x^2+4x+2=72$
$\Leftrightarrow 2(x^2+2x+1)=72$
$\Leftrightarrow 2(x+1)^2=72$
$\Leftrightarrow (x+1)^2=36$
$\Rightarrow x+1=\pm 6$
$\Rightarrow x=5$ hoặc $x=-7$
31 tháng 10 2021
\(\Leftrightarrow\left(x^2+2x\right)^2-2\left(x^2+2x\right)-3=0\)
\(\Leftrightarrow\left(x^2+2x-3\right)\left(x^2+2x+1\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x-1\right)\left(x+1\right)^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=1\\x=-1\end{matrix}\right.\)
PT
1
DN
2
4 tháng 9 2021
\(=\left(x^2+5x+8\right)\left(x^2+4x+2x+8\right)=\left(x^2+5x+8\right)\left[x\left(x+4\right)+2\left(x+4\right)\right]\)
\(=\left(x^2+5x+8\right)\left(x+2\right)\left(x+4\right)\)
4 tháng 9 2021
\(\left(x^2+4x+8\right)^2+3x\left(x^2+4x+8\right)+2x^2=\left(x^2+4x+8\right)^2+2x\left(x^2+4x+8\right)+x\left(x^2+4x+8\right)+2x^2\)
\(=\left(x^2+4x+8\right)\left(x^2+4x+8+2x\right)+x\left(x^2+4x+8+2x\right)\)
\(=\left(x^2+4x+8\right)\left(x^2+6x+8\right)+x\left(x^2+6x+8\right)\)
\(=\left(x^2+4x+8+x\right)\left(x^2+6x+8\right)=\left(x^2+5x+8\right)\left(x^2+6x+8\right)\)
19 tháng 2 2020
\(a,\left(x-1\right)\left(5x+3\right)=\left(3x-8\right)\left(x-1\right)\)
\(\left(x-1\right)\left(5x+3-3x+8\right)=0\)
\(\left(x-1\right)\left(2x+11\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-1=0\\2x+11=0\end{cases}\Rightarrow\orbr{\begin{cases}x=1\\2x=-11\end{cases}\Rightarrow}\orbr{\begin{cases}x=1\\x=-\frac{11}{2}\end{cases}}}\)
19 tháng 2 2020
\(b,3x\left(25x+15\right)-35\left(5x+3\right)=0\)
\(15x\left(5x+3\right)-35\left(5x+3\right)=0\)
\(\left(5x+3\right).5\left(3x-7\right)=0\)
\(\Rightarrow\orbr{\begin{cases}5x+3=0\\5\left(3x-7\right)=0\end{cases}\Rightarrow\orbr{\begin{cases}5x=-3\\3x-7=0\end{cases}\Rightarrow}\orbr{\begin{cases}x=-\frac{3}{5}\\3x=7\end{cases}\Rightarrow}\orbr{\begin{cases}x=-\frac{3}{5}\\x=\frac{7}{3}\end{cases}}}\)
MN
15 tháng 3 2021
\(\left(2x^2+1\right)\left(4x-3\right)=\left(2x^2+1\right)\left(x-13\right)\)
\(\Leftrightarrow\left(2x^2+1\right)\left(4x-3-x+13\right)=0\)
\(\Leftrightarrow\left(2x^2+1\right)\left(3x+10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x^2+1=0\\3x+10=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2=-\dfrac{1}{2}\left(VN\right)\\x=-\dfrac{10}{3}\end{matrix}\right.\)
\(S=\left\{-\dfrac{10}{3}\right\}\)
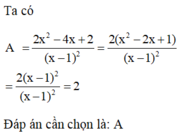
đề bài
2x2-4x+2
=2(x2-2x+1)
=2(x-1)2