Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\dfrac{1}{2}sin6x\ne0\)\(\Leftrightarrow sin6x\ne0\) \(\Leftrightarrow6x\ne k\pi\)\(\Leftrightarrow x\ne\dfrac{k\pi}{6}\)
\(\dfrac{1}{2}\ne0\) rồi nên chỉ cần \(sin6x\ne0\)

2.
\(\Leftrightarrow cos2x-cos8x-sin3x+cos5x-2sin5x.cos5x=0\)
\(\Leftrightarrow2sin5x.sin3x-sin3x+cos5x-2sin5x.cos5x=0\)
\(\Leftrightarrow sin3x\left(2sin5x-1\right)-cos5x\left(2sin5x-1\right)=0\)
\(\Leftrightarrow\left(sin3x-cos5x\right)\left(2sin5x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos5x=sin3x=cos\left(\dfrac{\pi}{2}-3x\right)\\sin5x=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=\dfrac{\pi}{2}-3x+k2\pi\\5x=3x-\dfrac{\pi}{2}+k2\pi\\5x=\dfrac{\pi}{6}+k2\pi\\5x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{16}+\dfrac{k\pi}{4}\\x=-\dfrac{\pi}{4}+k\pi\\x=\dfrac{\pi}{30}+\dfrac{k2\pi}{5}\\x=\dfrac{\pi}{6}+\dfrac{k2\pi}{5}\end{matrix}\right.\)
3.
\(\Leftrightarrow1+sinx=cosx-cos3x+2sinx.cosx+1-2sin^2x\)
\(\Leftrightarrow sinx=2sin2x.sinx+2sinx.cosx-2sin^2x\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\Rightarrow x=k\pi\\1=2sin2x+2cosx-2sinx\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow4sinx.cosx+2cosx-2sinx-1=0\)
\(\Leftrightarrow2cosx\left(2sinx+1\right)-\left(2sinx+1\right)=0\)
\(\Leftrightarrow\left(2cosx+1\right)\left(2sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\dfrac{1}{2}\\cosx=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow...\)

ĐK: `x \ne kπ`
`cot(x-π/4)+cot(π/2-x)=0`
`<=>cot(x-π/4)=-cot(π/2-x)`
`<=>cot(x-π/4)=cot(x-π/2)`
`<=> x-π/4=x-π/2+kπ`
`<=>0x=-π/4+kπ` (VN)
Vậy PTVN.




Đặt \(cosx-sinx=t\Rightarrow-\sqrt{2}\le t\le\sqrt{2}\)
\(t^2=1-2sinx.cosx\Rightarrow sinx.cosx=\dfrac{1-t^2}{2}\)
Pt trở thành:
\(t\left(1+\dfrac{1-t^2}{2}\right)+1=0\)
\(\Leftrightarrow t^3-3t-2=0\)
\(\Leftrightarrow\left(t-2\right)\left(t+1\right)^2=0\Rightarrow\left[{}\begin{matrix}t=2\left(loại\right)\\t=-1\end{matrix}\right.\)
\(\Rightarrow cosx-sinx=-1\)
\(\Leftrightarrow\sqrt[]{2}cos\left(x+\dfrac{\pi}{4}\right)=-1\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{4}\right)=-\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{4}\right)=cos\left(\dfrac{3\pi}{4}\right)\)
\(\Leftrightarrow...\)

3:
a: CD vuông góc AD
CD vuông góc SA
=>CD vuông góc (SAD)
b: BC vuông góc AB
BC vuông góc SA
=>BC vuông góc (SAB)
=>(SBC) vuông góc (SAB)

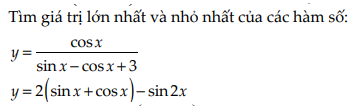
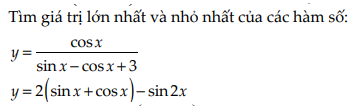


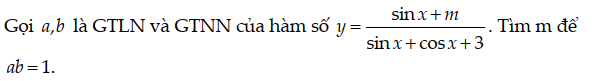

Câu 2 á mn
\(IM=\dfrac{1}{4}IB\Rightarrow IM=\dfrac{1}{5}BM\Rightarrow\overrightarrow{MI}=\dfrac{1}{5}\overrightarrow{MB}=-\dfrac{1}{10}\left(\overrightarrow{BC}+\overrightarrow{BD}\right)\)
\(\Rightarrow\overrightarrow{DI}=\overrightarrow{DM}+\overrightarrow{MI}=\dfrac{1}{2}\overrightarrow{DC}-\dfrac{1}{10}\left(\overrightarrow{BC}+\overrightarrow{BD}\right)=\dfrac{1}{2}\overrightarrow{DB}+\dfrac{1}{2}\overrightarrow{BC}-\dfrac{1}{10}\overrightarrow{BC}-\dfrac{1}{10}\overrightarrow{BD}\)
\(\Rightarrow\overrightarrow{DI}=\dfrac{2}{5}\overrightarrow{BC}-\dfrac{3}{5}\overrightarrow{BD}\)
\(\overrightarrow{DJ}=\overrightarrow{DC}+\overrightarrow{CJ}=\overrightarrow{DB}+\overrightarrow{BC}+x.\overrightarrow{CB}=\left(1-x\right)\overrightarrow{BC}-\overrightarrow{BD}\)
D; I; J thẳng hàng \(\Rightarrow\dfrac{1-x}{\dfrac{2}{5}}=\dfrac{1}{\dfrac{3}{5}}\Rightarrow x=\dfrac{1}{3}\)
\(\Rightarrow CJ=\dfrac{1}{3}CB\Rightarrow BJ=\dfrac{2}{3}BC\Rightarrow\dfrac{BJ}{BC}=\dfrac{2}{3}\)
Gọi N là trung điểm AD \(\Rightarrow\dfrac{BG}{BN}=\dfrac{2}{3}\) (theo t/c trọng tâm)
\(\Rightarrow\dfrac{BJ}{BC}=\dfrac{BG}{BN}\Rightarrow JG||CN\)
\(\Rightarrow\widehat{\left(JG;CD\right)}=\widehat{\left(CN;CD\right)}=\widehat{NCD}=30^0\) (do tam giác ACD đều)