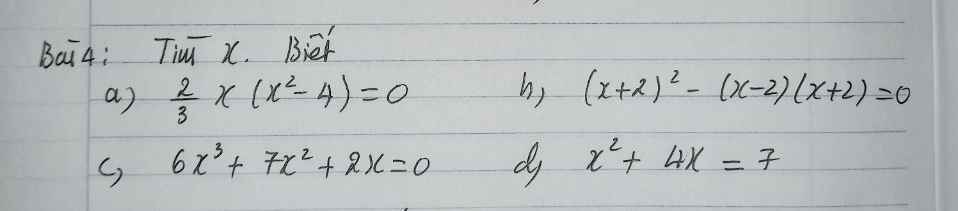
Cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




thay x=1/2 vào phương trình, ta được:
(1/2+a)/(a-1/2)+(1/2-a)/(a+1/2) =(a.(5a+10))/(a2-(1/2)2) (a khác +- 1/2)
<=>((1/2+a)2)/(a2-(1/2)2) +((1/2-a).(a-1/2))/(a2-(1/2)2) -(a.(5a+10))/(a2-(1/2)2) =0
<=> a2+a+1/4+a/2-a2-1/4+a/2-5a2+a=0
<=>2a+2a/2-5a2 =0
<=>4a+2a-10a2=0
<=>6a-10a2=0
<=> 2a(3-5a)=0
<=>a=0 hoặc a=3/5(tmđk)
vậy a=0 hoặc a=3/5

a: \(\dfrac{2}{3}x\left(x^2-4\right)=0\)
=>\(x\left(x-2\right)\left(x+2\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x-2=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\\x=-2\end{matrix}\right.\)
b: \(\left(x+2\right)^2-\left(x-2\right)\left(x+2\right)=0\)
=>\(\left(x+2\right)\left(x+2-x+2\right)=0\)
=>4(x+2)=0
=>x+2=0
=>x=-2
c: \(6x^3+7x^2+2x=0\)
=>\(x\left(6x^2+7x+2\right)=0\)
=>\(x\left(6x^2+4x+3x+2\right)=0\)
=>\(x\left(3x+2\right)\left(2x+1\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\3x+2=0\\2x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{2}{3}\\x=-\dfrac{1}{2}\end{matrix}\right.\)
d: \(x^2+4x=7\)
=>\(x^2+4x+4=11\)
=>\(\left(x+2\right)^2=11\)
=>\(\left[{}\begin{matrix}x+2=\sqrt{11}\\x+2=-\sqrt{11}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{11}-2\\x=-\sqrt{11}-2\end{matrix}\right.\)