Giúp mình bài này nhé, nhanh nhé, sắp đến giờ mik hc r:
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1) \(A=\frac{2x+1}{x^2+2}\)
\(=\frac{\frac{1}{2}\left(x^2+4x+4\right)-\frac{1}{2}\left(x^2+2\right)}{x^2+2}\)
\(=\frac{\left(x+2\right)^2}{2\left(x^2+2\right)}-\frac{1}{2}\ge-\frac{1}{2}\)
Dấu "=" xảy ra \(\Leftrightarrow x+2=0\Leftrightarrow x=-2\)
Vậy GTNN của \(A=-\frac{1}{2}\)khi x = -2

Lời giải:
a. $y=\sqrt{x^2+x-2}\geq 0$ (tính chất cbh số học)
Vậy $y_{\min}=0$. Giá trị này đạt tại $x^2+x-2=0\Leftrightarrow x=1$ hoặc $x=-2$
b.
$y^2=6+2\sqrt{(2+x)(4-x)}\geq 6$ do $2\sqrt{(2+x)(4-x)}\geq 0$ theo tính chất căn bậc hai số học
$\Rightarrow y\geq \sqrt{6}$ (do $y$ không âm)
Vậy $y_{\min}=\sqrt{6}$ khi $x=-2$ hoặc $x=4$
$y^2=(\sqrt{2+x}+\sqrt{4-x})^2\leq (2+x+4-x)(1+1)=12$ theo BĐT Bunhiacopxky
$\Rightarrow y\leq \sqrt{12}=2\sqrt{3}$
Vậy $y_{\max}=2\sqrt{3}$ khi $2+x=4-x\Leftrightarrow x=1$
c. ĐKXĐ: $-2\leq x\leq 2$
$y^2=(x+\sqrt{4-x^2})^2\leq (x^2+4-x^2)(1+1)$ theo BĐT Bunhiacopxky
$\Leftrightarrow y^2\leq 8$
$\Leftrightarrow y\leq 2\sqrt{2}$
Vậy $y_{\max}=2\sqrt{2}$ khi $x=\sqrt{2}$
Mặt khác:
$x\geq -2$
$\sqrt{4-x^2}\geq 0$
$\Rightarrow y\geq -2$
Vậy $y_{\min}=-2$ khi $x=-2$

a) Ta có:\(|2x-4|\ge0\forall x\)
\(\Rightarrow|2x-4|+13\ge13\forall x\)
hay A\(\ge13\forall x\)
Dấu "=" \(\Leftrightarrow|2x-4|=0\)
<=> 2x-4=0
<=> 2x=4
<=>x=2
Vậy Min A=13 đạt được khi x=2
b) Làm tương tự câu a)
c) \(C=\left(x-5\right)^2+25\)
Ta có: \(\left(x-5\right)^2\ge0\forall x\)
\(\Rightarrow\left(x-5\right)^2+25\ge25\forall x\)
hay C \(\ge25\)
Dấu "=" <=> (x-5)2 =0
<=> x-5=0
<=> x=5
Vậy Min C=25 đạt được khi x=5
d) Làm tương tự c)
a) Vì \(\left|2x-4\right|\ge0\)
\(\Rightarrow\left|2x-4\right|+13\ge13\)
\(\Rightarrow A_{min} =13\)
b) Vì \(\hept{\begin{cases}\left|x+5\right|\ge0\\\left|2y-16\right|\ge0\end{cases}}\)
\(\Rightarrow\left|x+5\right|+\left|2y-16\right|+2015\ge0\)
\(\Rightarrow B_{min}=2015\)
Các phần sau làm tương tự như thế ^_^
Chúc bạn học tốt

1/ B = (x+y)((x+y)2 - 3xy)+(x+y)2 - 2xy = 2 - 5xy = 2 - 5x(1-x)=5x2 - 5x + 2 = (x√5 - √5 /2)2 +3/4 >= 3/4
Đạt GTNN là 3/4 khi x=y=1/2
2/ P = xy = x(6-x)=-x2 +6x = 9 - (x-3)2 <=9
GTLN là 9 khi x=y=3

Từ gt ta có x^2+y^^2=xy+1
=>P=(x^2+y^2)^2-2x^2y^2-x^2y^2
=(xy+1)2-2x2y2-x2y2
=x2y2+xy+1-3x2y2=-2x2y2+xy+1
=......
\(1=x^2+y^2-xy\ge2xy-xy=xy\Rightarrow xy\le1\)
\(1=x^2+y^2-xy\ge-2xy-xy=-3xy\Rightarrow xy\ge-\dfrac{1}{3}\)
\(\Rightarrow-\dfrac{1}{3}\le xy\le1\)
\(P=\left(x^2+y^2\right)^2-2\left(xy\right)^2-\left(xy\right)^2=\left(xy+1\right)^2-3\left(xy\right)^2=-2\left(xy\right)^2+2xy+1\)
Đặt \(xy=t\in\left[-\dfrac{1}{3};1\right]\)
\(P=f\left(t\right)=-2t^2+2t+1\)
\(f'\left(t\right)=-4t+2=0\Rightarrow t=\dfrac{1}{2}\)
\(f\left(-\dfrac{1}{3}\right)=\dfrac{1}{9}\) ; \(f\left(\dfrac{1}{2}\right)=\dfrac{3}{2}\) ; \(f\left(1\right)=1\)
\(\Rightarrow P_{max}=\dfrac{3}{2}\) ; \(P_{min}=\dfrac{1}{9}\)
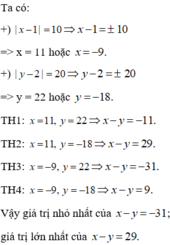
\(x^2+2>=2\forall x\)
=>\(\left(x^2+2\right)^2>=4\forall x\)
=>\(-\left(x^2+2\right)^2< =-4\forall x\)
mà \(-\left(y^2-16\right)^4< =0\forall y\)
nên \(-\left(x^2+2\right)^2-\left(y^2-16\right)^4< =-4\forall x,y\)
=>\(B=-\left(x^2+2\right)^2-\left(y^2-16\right)^4+20< =-4+20=16\forall x,y\)
Dấu '=' xảy ra khi \(\left\{{}\begin{matrix}x=0\\y^2-16=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y\in\left\{4;-4\right\}\end{matrix}\right.\)