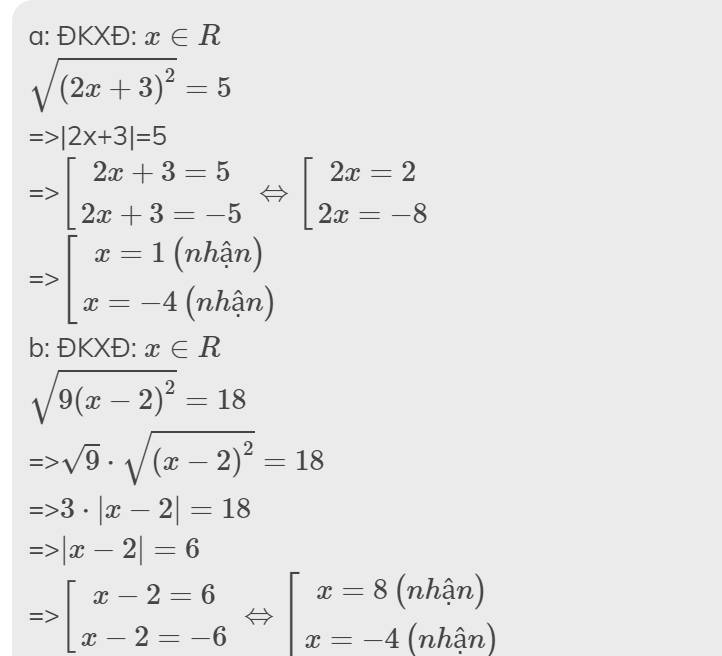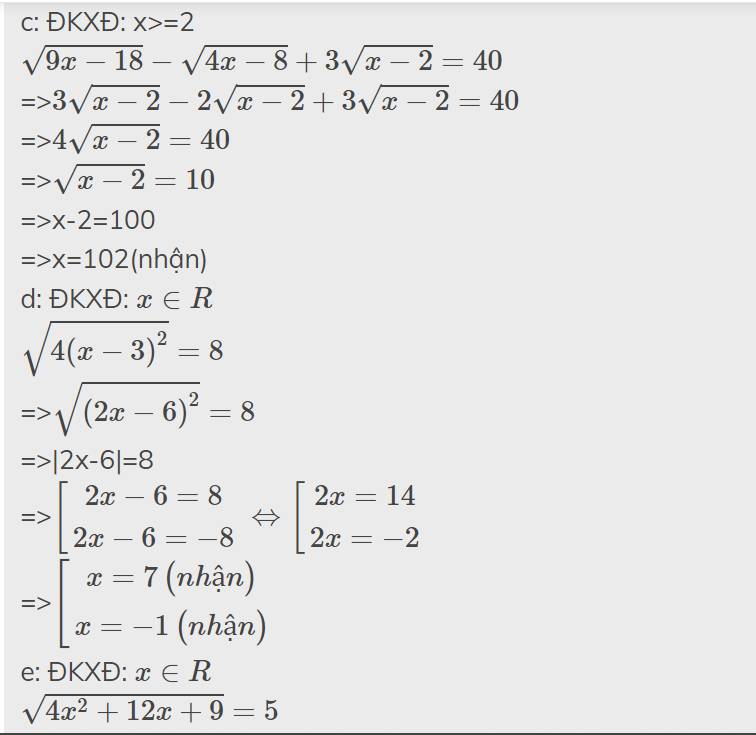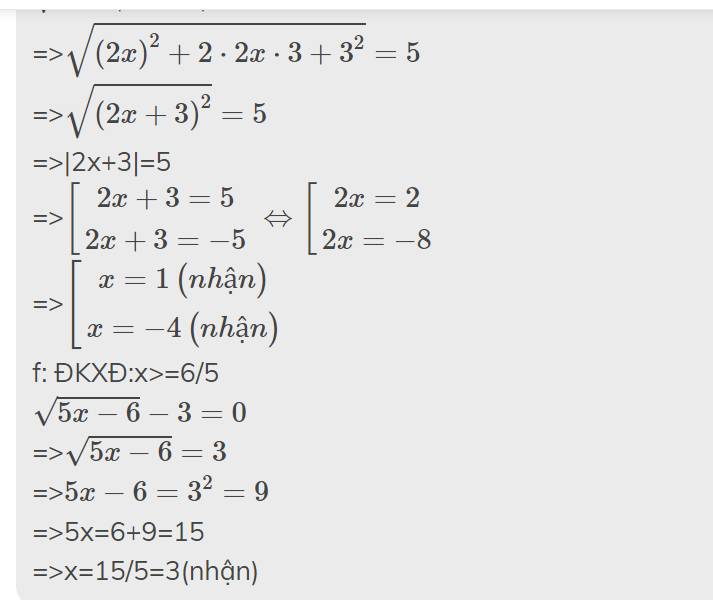giải phương trình \(\left(x+3\sqrt{x}+2\right)\left(x+9\sqrt{x}+18\right)=168x\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TN
1
29 tháng 10 2017
\(\Leftrightarrow\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)\left(\sqrt{x}+3\right)\left(\sqrt{x}+6\right)=168x\\ \Leftrightarrow\left(x+7\sqrt{x}+6\right)\left(x+5\sqrt{x}+6\right)-168x=0\\ \Leftrightarrow\left(x+6\sqrt{x}+6\right)^2-\left(13\sqrt{x}\right)^2=0\\ \left(x-7\sqrt{x}+6\right)\left(x+19\sqrt{x}+6\right)=0 \\ \left(\sqrt{x}-1\right)\left(\sqrt{x}-6\right)=0\)
30 tháng 7 2021
a) ĐKXĐ: \(x\ge0\)
Ta có: \(\left(x+3\sqrt{x}+2\right)\left(x+9\sqrt{x}+18\right)=168x\)
\(\Leftrightarrow\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)\left(\sqrt{x}+3\right)\left(\sqrt{x}+6\right)=168x\)
\(\Leftrightarrow\left(x+6\right)^2+12\sqrt{x}\left(x+6\right)-133=0\)
\(\Leftrightarrow\left(x+6\right)^2+19\sqrt{x}\left(x+6\right)-7\sqrt{x}\left(x+6\right)-133=0\)
\(\Leftrightarrow\left(x+6\right)\left(x+19\sqrt{x}+6\right)-7\sqrt{x}\left(x+19\sqrt{x}+6\right)=0\)
\(\Leftrightarrow\left(x-7\sqrt{x}+6\right)=0\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)\left(\sqrt{x}-6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=36\end{matrix}\right.\)
NT
30 tháng 7 2021
Dòng thứ 2 qua dòng thứ 3 anh làm chậm lại được không ạ, tại tắt quá e không hiểu
CD
0
HT
2
9 tháng 5 2016
Dễ thấy \(x=0\) không là nghiệm của phương trình. Ta có "
\(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+6\right)=168x^2\Leftrightarrow\left(x^2+7x+6\right)\left(x^2+5x+6\right)=168x^2\)
\(\Leftrightarrow\left(x+\frac{6}{x}+7\right)\left(x+\frac{6}{x}+5\right)=168\)
Đặt \(t=x+\frac{6}{x}\) ta được :
\(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+6\right)=168x^2\Leftrightarrow\left(t+7\right)\left(t+5\right)=168\)
\(\Leftrightarrow t^2+12t-133=0\Leftrightarrow\left[\begin{array}{nghiempt}t=7\\t=-19\end{array}\right.\)
Do vậy :
\(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+6\right)=168x^2\Leftrightarrow\begin{cases}x+\frac{6}{x}=7\\x+\frac{6}{x}=-19\end{cases}\)
\(\Leftrightarrow\begin{cases}x^2-7x+6=0\\x^2+19x+6=0\end{cases}\)
\(\Leftrightarrow\begin{cases}x=1\\x=6\\x=\frac{-19\pm\sqrt{337}}{2}\end{cases}\)
Vậy phương trình đã cho có tập nghiệm :
\(\left\{1;6;\frac{-19-\sqrt{337}}{2};\frac{-19+\sqrt{337}}{2}\right\}\)
9 tháng 5 2016
\(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+6\right)=168x^2\)
<=>\(\left(x+1\right)\left(x+6\right)\left(x+2\right)\left(x+3\right)=168x^2\)
<=>\(\left(x^2+7x+6\right)\left(x^2+5x+6\right)=168x^2\)(1)
Đặt t=x2+5x+6
PT (1) trở thành: (t+2x)t=168x2
<=>t2+2tx-168x2=0
<=>t2-12tx+14tx-168x2=0
<=>t.(t-12x)+14x.(t-12x)=0
<=>(t-12x)(t+14x)=0
<=>t-12x=0 hoặc t+14x=0
*t-12x=0 (thích giải denta cũng được)
<=>x2-7x+6=0
<=>x2-x-6x+6=0
<=>x.(x-1)-6.(x-1)=0
<=>(x-1)(x-6)=0
<=>x=1 hoặc x=6
*t+14x=0
<=>x2+19x+6=0
Giải denta là vừa tại số lớn lắm tự làm típ ..............
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 7 2021
a. Đề bài sai, phương trình không giải được
b.
ĐKXĐ: \(x\ge-\dfrac{2}{3}\)
\(\left(2x+10\right)\left(\dfrac{1-\left(3+2x\right)}{1+\sqrt{3+2x}}\right)^2=4\left(x+1\right)^2\)
\(\Leftrightarrow\dfrac{\left(2x+10\right)4.\left(x+1\right)^2}{\left(1+\sqrt{3+2x}\right)^2}=4\left(x+1\right)^2\)
\(\Leftrightarrow\left[{}\begin{matrix}4\left(x+1\right)^2=0\Rightarrow x=-1\\2x+10=\left(1+\sqrt{3+2x}\right)^2\left(1\right)\end{matrix}\right.\)
Xét (1)
\(\Leftrightarrow2x+10=2x+4+2\sqrt{2x+3}\)
\(\Leftrightarrow\sqrt{2x+3}=3\)
\(\Leftrightarrow x=3\)