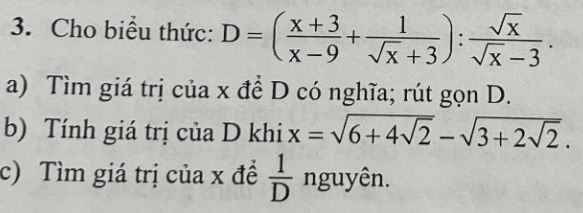
Mình cần gấp ạ!!! Cảm ơn!
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




a) \(7^2-7\left(13-x\right)=14\)
\(7\left(13-x\right)=49-14=35\)
\(13-x=5\)
\(x=13-5=8\)
b) \(5x-5^2=10\)
\(5x=10+25=35\)
\(x=7\)
c) \(4\left(x-5\right)-2^3=2^4.3=48\)
\(4\left(x-5\right)=48+8=56\)
\(x-5=14\)
\(x=19\)
4:
a: \(\Leftrightarrow49+7\left(x-13\right)=14\)
=>7(x-13)=35
=>x-13=5
=>x=18
b: \(5x-5^2=10\)
=>\(5x=10+25=35\)
=>x=7
c: \(4\left(x-5\right)-2^3=2^4\cdot3\)
=>\(4\left(x-5\right)=16\cdot3+8=56\)
=>x-5=14
=>x=19

Câu 4 :
\(n_{Fe2O3}=\dfrac{24}{160}=0,15\left(mol\right)\)
Pt : \(Fe_2O_3+3H_2SO_4\rightarrow Fe_2\left(SO_4\right)_3+3H_2O|\)
1 3 1 3
0,15 0,15
a) \(n_{Fe2\left(SO4\right)3}=\dfrac{0,15.1}{1}=0,15\left(mol\right)\)
⇒ \(m_{Fe2\left(SO4\right)3}=0,15.400=60\left(g\right)\)
b) \(C_{M_{Fe2\left(SO4\right)3}}=\dfrac{0,15}{0,5}=0,3\left(M\right)\)
Chúc bạn học tốt
a,\(n_{Fe_2O_3}=\dfrac{24}{160}=0,15\left(mol\right)\)
PTHH: Fe2O3 + 3H2SO4 → Fe2(SO4)3 + 3H2O
Mol: 0,15 0,45 0,15
\(m_{Fe_2\left(SO_4\right)_3}=0,15.400=60\left(g\right)\)
b,\(C_{M_{ddFe_2\left(SO_4\right)_3}}=\dfrac{0,15}{0,5}=0,3\left(mol\right)\)


Câu 9:
a) Ta có: \(9x^2-16=0\)
\(\Leftrightarrow\left(3x-4\right)\left(3x+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{4}{3}\\x=-\dfrac{4}{3}\end{matrix}\right.\)
b) Ta có: \(4x^2=13\)
\(\Leftrightarrow x^2=\dfrac{13}{4}\)
\(\Leftrightarrow x\in\left\{\dfrac{\sqrt{13}}{2};-\dfrac{\sqrt{13}}{2}\right\}\)
c) Ta có: \(2x^2+9=0\)
\(\Leftrightarrow2x^2=-9\)(Vô lý)
d) Ta có: \(-x^2+324=0\)
\(\Leftrightarrow x^2=324\)
\(\Leftrightarrow\left[{}\begin{matrix}x=18\\x=-18\end{matrix}\right.\)


a: ĐKXĐ: x>0; x<>9
\(D=\dfrac{x+3+\sqrt{x}-3}{x-9}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}}\)
\(=\dfrac{x+\sqrt{x}}{\sqrt{x}}\cdot\dfrac{1}{\sqrt{x}+3}=\dfrac{\sqrt{x}+1}{\sqrt{x}+3}\)
b: x=2+căn 2-căn 2-1=1
Khi x=1 thì \(D=\dfrac{1+1}{1+3}=\dfrac{2}{4}=\dfrac{1}{2}\)
c: P=1:D=(căn x+3)/(căn x+1)
P nguyên
=>căn x+1+2 chia hết cho căn x+1
=>căn x+1 thuộc {1;2}
=>x=0(loại) hoặc x=1(nhận)
a) D có nghĩa khi: \(\left\{{}\begin{matrix}x\ge0\\x\ne9\end{matrix}\right.\)
\(D=\left(\dfrac{x+3}{x-9}-\dfrac{1}{\sqrt{x}+3}\right):\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
\(D=\left(\dfrac{x+3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}-\dfrac{\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right):\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
\(D=\left(\dfrac{x+3+\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right):\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
\(D=\dfrac{\left(x+\sqrt{x}\right)\cdot\left(\sqrt{x}-3\right)}{\sqrt{x}\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(D=\dfrac{x+\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+3\right)}=\dfrac{x+\sqrt{x}}{x+3\sqrt{x}}\)
\(D=\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}+3}\)
b) \(x=\sqrt{6+4\sqrt{2}}-\sqrt{3+2\sqrt{2}}\)
\(x=\sqrt{2^2+2\cdot2\cdot\sqrt{2}+\left(\sqrt{2}\right)^2}-\sqrt{\left(\sqrt{2}\right)^2+2\cdot\sqrt{2}\cdot1+1^2}\)
\(x=\sqrt{\left(2+\sqrt{2}\right)^2}-\sqrt{\left(\sqrt{2}+1\right)^2}\)
\(x=\left|2+\sqrt{2}\right|-\left|\sqrt{2}+1\right|\)
\(x=2+\sqrt{2}-\sqrt{2}-1=1\)
Thay \(x=1\) vào D ta được:
\(D=\dfrac{\sqrt{1}+1}{\sqrt{1}+3}=\dfrac{2}{4}=\dfrac{1}{2}\)
c) Cho \(A=\dfrac{1}{D}=1:\left(\dfrac{\sqrt{x}+1}{\sqrt{x}+3}\right)=\dfrac{\sqrt{x}+3}{\sqrt{x}+1}=\dfrac{\sqrt{x}+1+2}{\sqrt{x}+1}=\dfrac{\sqrt{x}+1}{\sqrt{x}+1}+\dfrac{2}{\sqrt{x}+1}=1+\dfrac{2}{\sqrt{x}+1}\)
A nguyên khi \(2⋮\sqrt{x}+1\)
\(\Rightarrow\sqrt{x}+1\inƯ\left(2\right)=\left\{1;2;-1;-2\right\}\)
Mà: \(\sqrt{x}>0\)
\(\Rightarrow\sqrt{x}+1\in\left\{1;2\right\}\)
\(\Rightarrow\left\{{}\begin{matrix}x=0\left(ktm\right)\\x=1\left(tm\right)\end{matrix}\right.\)