Cho đa thức
M(x)=1+x+x2+x3+x4+...+x100
N(x)=x2+x4+x6+x8+...+x100
Tính M(x)-N(x) tại x=-1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
28 tháng 7 2023
A(x)=F(x)-G(x)
=1+x+x^2+...+x^100-x^2-x^4-...-x^100
=1+x+x^3+...+x^99
Số số lẻ từ 1 đến 99 là (99-1):2+1=50(số)
A(-1)=1+(-1)+(-1)^3+...+(-1)^99
=1-50*1=1-50=-49
PT
1
CM
10 tháng 1 2017
Thay x = -1 và đa thức, ta có:
(-1)2 + (-1)4 + (-1)6 + … + (-1)100 = 
Vậy giá trị đa thức bằng 50 tại x = -1.
CM
19 tháng 4 2017
Ta đặt và thực hiện phép tính P(x) + Q(x) và P(x) – Q(x) có
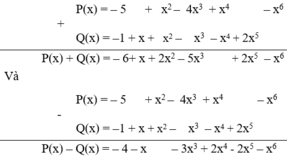
Vậy: P(x) + Q(x) = – 6 + x + 2x2 – 5x3 + 2x5 – x6
P(x) – Q(x) = – 4 – x – 3x3 + 2x4 - 2x5 – x6
CM
21 tháng 8 2018
P(x) = 3x2 – 5 + x4 – 3x3 – x6 – 2x2 – x3
= – x6 + x4 + (– 3x3 – x3) + (3x2 – 2x2) – 5
= – x6 + x4 – 4x3 + x2 – 5.
= – 5+ x2 – 4x3 + x4 – x6
Và Q(x) = x3 + 2x5 – x4 + x2 – 2x3 + x –1
= 2x5 – x4 + (x3 – 2x3) + x2 + x –1
= 2x5 – x4 – x3 + x2 + x –1.
= –1+ x + x2 – x3 – x4 + 2x5
AH
Akai Haruma
Giáo viên
25 tháng 10 2021
a.
$x^2-y^2-2x+2y=(x^2-y^2)-(2x-2y)=(x-y)(x+y)-2(x-y)=(x-y)(x+y-2)$
b.
$x^2(x-1)+16(1-x)=x^2(x-1)-16(x-1)=(x-1)(x^2-16)=(x-1)(x-4)(x+4)$
c.
$x^2+4x-y^2+4=(x^2+4x+4)-y^2=(x+2)^2-y^2=(x+2-y)(x+2+y)$
d.
$x^3-3x^2-3x+1=(x^3+1)-(3x^2+3x)=(x+1)(x^2-x+1)-3x(x+1)$
$=(x+1)(x^2-4x+1)$
AH
Akai Haruma
Giáo viên
25 tháng 10 2021
e.
$x^4+4y^4=(x^2)^2+(2y^2)^2+2.x^2.2y^2-4x^2y^2$
$=(x^2+2y^2)^2-(2xy)^2=(x^2+2y^2-2xy)(x^2+2y^2+2xy)$
f.
$x^4-13x^2+36=(x^4-4x^2)-(9x^2-36)$
$=x^2(x^2-4)-9(x^2-4)=(x^2-9)(x^2-4)=(x-3)(x+3)(x-2)(x+2)$
g.
$(x^2+x)^2+4x^2+4x-12=(x^2+x)^2+4(x^2+x)-12$
$=(x^2+x)^2-2(x^2+x)+6(x^2+x)-12$
$=(x^2+x)(x^2+x-2)+6(x^2+x-2)=(x^2+x-2)(x^2+x+6)$
$=[x(x-1)+2(x-1)](x^2+x+6)=(x-1)(x+2)(x^2+x+6)$
h.
$x^6+2x^5+x^4-2x^3-2x^2+1$
$=(x^6+2x^5+x^4)-(2x^3+2x^2)+1$
$=(x^3+x^2)^2-2(x^3+x^2)+1=(x^3+x^2-1)^2$
13 tháng 11 2021
2: \(=\dfrac{\left(x-y\right)\left(x+y\right)\left(x^2+y^2\right)}{-\left(x-y\right)\left(x^2+xy+y^2\right)}=\dfrac{-\left(x+y\right)\left(x^2+y^2\right)}{x^2+xy+y^2}\)
BN
27 tháng 4 2017
a) \(M\left(x\right)=-x^6+x^4-4x^3+x^2-5\)
\(N\left(x\right)=2x^5-x^4-x^3+x^2+x-1\)
b)\(M\left(x\right)+N\left(x\right)=-x^6+x^4-4x^3+x^2-5+2x^5-x^4-x^3+x^2+x-1\) \(=-x^6+2x^5-5x^3+2x^2+x-6\)
Vậy...
\(M\left(x\right)-N\left(x\right)=-x^6+x^4-4x^3+x^2-5-\left(2x^5+x^4-x^3+x^2-x-1\right)\)\(=-x^6+x^4-4x^3+x^2-5-2x^5+x^4+x^3-x^2+x+1\) \(=-x^6-2x^5+2x^4-3x^3+x-4\) Vậy...
c) -N(x)+M(x)=.........
HC
14 tháng 4 2017
a. M(x) = 3x2- 5+ x4- 3x3- x6- 2x2- x3
= - 5+ (3x2- 2x2)+(-3x3- x3)+ x4- x6
= - 5 +x2+ (-4x3) +x4 -x6
N(x) = x3 + 2x5 -x4 +x2 -2x3 +x-1
= -1+x+x2+(x3- 2x3) -x4+ 2x5
= -1+ x+ x2+ (-x3) -x4+ 2x5
b. M(x) +N(x)= (- 5 +x2 -4x3 +x4 -x6) + (-1+ x+ x2 -x3 -x4+ 2x5)
= - 5 +x2 -4x3 +x4 -x6 -1+ x+ x2 -x3 -x4+ 2x5
= (-5-1) + x +( x2 +x2) +(-4x3-x3) +(x4-x4) +2x5 -x6
= -6 +x +2x2 +(-5x3) +0 +2x5-x6
= -6 +x +2x2 +(-5x3) +2x5-x6
M(x) -N(x)= (- 5 +x2 -4x3 +x4 -x6) - (-1+ x+ x2 -x3 -x4+ 2x5)
= - 5 +x2 -4x3 +x4 -x6 -1-x -x2 +x3 +x4 -2x5
= (-5-1) +x +(x2-x2) +(-4x3+x3) +(x4+x4) -2x5 -x6
= -6 +x +0 +(-3x3) +2x4 -2x5 -x6
= -6 +x +(-3x3) +2x4 -2x5 -x6
\(M\left(x\right)=1+\left(-1\right)+\left(-1\right)^2+\left(-1\right)^3+\left(-1\right)^4+...+\left(-1\right)^{100}\)
\(=1+\left(-1\right)+\left(-1\right)^2+\left(-1\right)^3+\left(-1\right)^4+\left(-1\right)^5+...+\left(-1\right)^{98}+\left(-1\right)^{99}+\left(-1\right)^{100}\)
\(=1+\left(-1\right)+1+\left(-1\right)+1+\left(-1\right)+...+1+\left(-1\right)+1\)
\(=1\)
\(N\left(-1\right)=\left(-1\right)^2+\left(-1\right)^4+\left(-1\right)^6+\left(-1\right)^8+...+\left(-1\right)^{100}\)
\(=1+1+1+1+...+1\)
\(=50.1=50\)
\(M\left(-1\right)-N\left(-1\right)=1-50=-49\)
thak nhìu nhắm :D