Cho phương trình
x^2-3x+1
Có hai nghiệm là x1;x2
Đặt An=x1n+x2n(n>0)
a)CMR An+2=3An+1 - An
b)CMR An là số nguyên
c)CMR An-2 = {(√5+12 )n−(√5−12 )n}2
d) Tìm n để An-2 Là số chính phương
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(HPT\Leftrightarrow\left\{{}\begin{matrix}6x-2y=4m+6\\x+2y=3m+1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}7x=7m+7\\x+2y=3m+1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=m+1\\m+1+2y=3m+1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=m+1\\y=m\end{matrix}\right.\)
\(x^2+y^2=5\Leftrightarrow m^2+2m+1+m^2=5\\ \Leftrightarrow2m^2+2m-4=0\\ \Leftrightarrow m^2+m-2=0\\ \Leftrightarrow\left[{}\begin{matrix}m=1\\m=-2\end{matrix}\right.\)

Đáp án: A
![]()
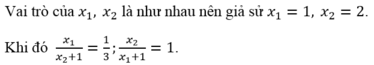
Ta tìm phương trình có 2 nghiệm là ![]() và 1. Ta có thể thử nghiệm vào từng phương trình xem phương trình nào thỏa mãn hoặc giải từng phương trình rồi so sánh nghiệm.
và 1. Ta có thể thử nghiệm vào từng phương trình xem phương trình nào thỏa mãn hoặc giải từng phương trình rồi so sánh nghiệm.

⇒ Chọn đáp án A.

1, Với x >= 0 ; x khác 1
\(P=\dfrac{\sqrt{x}\left(x-1\right)+2\sqrt{x}\left(\sqrt{x}-1\right)-\left(3x+1\right)\left(\sqrt{x}+1\right)}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x\sqrt{x}+2x-3\sqrt{x}-3x\sqrt{x}-3x-\sqrt{x}-1}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-2x\sqrt{x}-x-4\sqrt{x}-1}{\left(x-1\right)\left(\sqrt{x}+1\right)}\)
mình sửa đề câu 2 nhé
a, \(x^2+mx-1=0\)
\(\Delta=m^2-4\left(-1\right)=m^2+4>0\)
Vậy pt luôn có 2 nghiệm pb
b, Theo Vi et : \(\left\{{}\begin{matrix}x_1+x_2=-m\\x_1x_2=-1\end{matrix}\right.\)
Ta có : \(\left(x_1+x_2\right)^2-2x_1x_2=7\)
Thay vào ta được : \(m^2+2=7\Leftrightarrow m^2=5\Leftrightarrow m=\pm\sqrt{5}\)

a: Khi m=-5 thì pt sẽ là x^2-5x-6=0
=>x=6 hoặc x=-1
b:
Δ=(-5)^2-4(m-1)=25-4m+4=-4m+29
Để pt có hai nghiệm thì -4m+29>=0
=>m<=29/4
x1-x2=3
=>(x1-x2)^2=9
=>(x1+x2)^2-4x1x2=9
=>5^2-4(m-1)=9
=>4(m-1)=25-9=16
=>m-1=4
=>m=5(nhận)
c: 2x1-3x2=5 và x1+x2=5
=>x1=4 và x2=1
x1*x2=m-1
=>m-1=4
=>m=5(nhận)

Để pt: \(x^2-3x+m-2=0\) có hai nghiệm : \(x_1;x_2\) điều kiện là:
\(\Delta=9-4\left(m-2\right)\ge0\)
<=> \(m\le\frac{17}{4}\)( @@)
Áp dụng định lí viet ta có:
\(\hept{\begin{cases}x_1+x_2=3\\x_1.x_2=m-2\end{cases}}\)=> \(\left(x_1-x_2\right)^2=\left(x_1+x_2\right)^2-4x_1x_2=9-4\left(m-2\right)=17-4m\ge0\)
=> \(x_1-x_2=\sqrt{17-4m}\)
Ta có:
\(x_1^3-x_2^3+9x_1x_2=\left(x_1-x_2\right)^3+3\left(x_1-x_2\right)x_1x_2+9x_1x_2\)
\(=\sqrt{\left(17-4m\right)^3}+3\sqrt{17-4m}\left(m-2\right)+9\left(m-2\right)\)
Theo bài ra ta có phương trình:
\(\sqrt{\left(17-4m\right)^3}+3\sqrt{17-4m}\left(m-2\right)+9\left(m-2\right)=81\)
<=> \(\left(\sqrt{17-4m}\right)^3-3^3+3\left(m-2\right)\left(\sqrt{17-4m}-3\right)=0\)
<=> \(\left(\sqrt{17-4m}-3\right)\left(17-4m+3\sqrt{17-4m}+9+3\left(m-2\right)\right)=0\)
<=> \(\left(\sqrt{17-4m}-3\right)\left(20-m+3\sqrt{17-4m}\right)=0\)
TH1: \(\sqrt{17-4m}-3=0\Leftrightarrow17-4m=9\Leftrightarrow m=2\left(tm@@\right)\)
TH2: \(20-m+3\sqrt{17-4m}=0\)
<=> \(3\sqrt{17-4m}=m-20\)=> \(m-20\ge0\)=> \(m\ge20\) vô lí với (@@)
Vậy m = 2.

\(x^2-2x-1=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=-1\end{matrix}\right.\)
Đặt \(\left\{{}\begin{matrix}u=x_1+\left(x_2\right)^2\\v=x_2+\left(x_1\right)^2\end{matrix}\right.\)
\(\Rightarrow\)\(\left\{{}\begin{matrix}u+v=\left(x_1+x_2\right)+\left(x_2+x_1\right)^2-2x_1x_2\\uv=2x_1x_2+x_1^3+x_2^3=2x_1x_2+\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u+v=8\\uv=12\end{matrix}\right.\)
=>u và v là nghiệm của pt \(t^2-8t+12=0\)