Cho phương trình \(x^2-mx-2\left(m^2+8\right)=0\). Tìm m để phương trình có hai nghiệm thỏa mãn \(x_1^2+x_2^2=52\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
25 tháng 2 2022
a, bạn tự làm
b, Thay x = 3 vào pt trên ta được
\(9-3m-3=0\Leftrightarrow6-3m=0\Leftrightarrow m=2\)
Thay m = 2 vào ta được \(x^2-2x-3=0\)
Ta có a - b + c = 1 + 2 - 3 = 0
vậy pt có 2 nghiệm x = -1 ; x = 3
c, \(\Delta=m^2-4\left(-3\right)=m^2+12>0\)
vậy pt luôn có 2 nghiệm pb
\(x_1x_2+5\left(x_1+x_2\right)-1997=0\)
\(\Rightarrow-3+5m-1997=0\Leftrightarrow5m-2000=0\Leftrightarrow m=400\)
HP
12 tháng 8 2021
b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)
18 tháng 8 2021
a: Để phương trình có hai nghiệm trái dấu thì \(\left(m^2-m-6\right)\cdot1< 0\)
\(\Leftrightarrow\left(m-3\right)\left(m+2\right)< 0\)
\(\Leftrightarrow-2< m< 3\)
6 tháng 5 2022
cái biêủ thức đề bài biến đổi để kết hợp với pt tổng trong Viet ra hệ pt tìm ra x1 x2 ròi that vào pt tích trong viet
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 3 2022
\(\Delta'=\left(m-1\right)^2+2m=m^2+1>0;\forall m\)
\(\Rightarrow\) Pt luôn có 2 nghiệm pb với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=-2m\end{matrix}\right.\)
Cộng vế với vế: \(x_1x_2+x_1+x_2=-2\) (1)
\(x_1^2+x_1-x_2=5-2m\)
\(\Leftrightarrow x_1^2+x_1-x_2=5+x_1x_2\) (2)
Cộng vế với vế (1) và (2):
\(\Rightarrow x_1^2+2x_1=3\)
\(\Leftrightarrow x_1^2+2x_1-3=0\Rightarrow\left[{}\begin{matrix}x_1=1\Rightarrow x_2=-\dfrac{3}{2}\\x_1=-3\Rightarrow x_2=-\dfrac{1}{2}\end{matrix}\right.\) (thế \(x_1\) vào (1) để tính ra \(x_2\))
Thế vào \(x_1x_2=-2m\Rightarrow m=-\dfrac{x_1x_2}{2}\Rightarrow m=\pm\dfrac{3}{4}\)
DH
2 tháng 3 2021
Ta có: \(\Delta=\left(m-4\right)^2-4m.2m=m^2-8m+16-8m^2=-7m^2-8m+16\)
Để phương trình có nghiệm thì \(\Delta>0\Rightarrow\dfrac{-4-8\sqrt{2}}{7}< x< \dfrac{-4+8\sqrt{2}}{7}\)
Áp dụng định lý Vi-et ta có:
\(x_1+x_2=\dfrac{\left(m-4\right)}{m};x_1.x_2=2\) (1)
Mặt khác ta lại có: \(2\left(x_1^2+x_2^2\right)-5x_1x_2=0\\ \Rightarrow2\left(x_1+x_2\right)^2-7x_1x_2=0\)(2)
Thay (1) vào (2) ta được
\(2\left(\dfrac{m-4}{m}\right)^2-7.2=0\)
\(\Rightarrow\left[{}\begin{matrix}m=\dfrac{4}{1-\sqrt{7}}\\m=\dfrac{4}{1+\sqrt{7}}\end{matrix}\right.\) (Loại)
Do đó không có giá trị m thỏa mãn
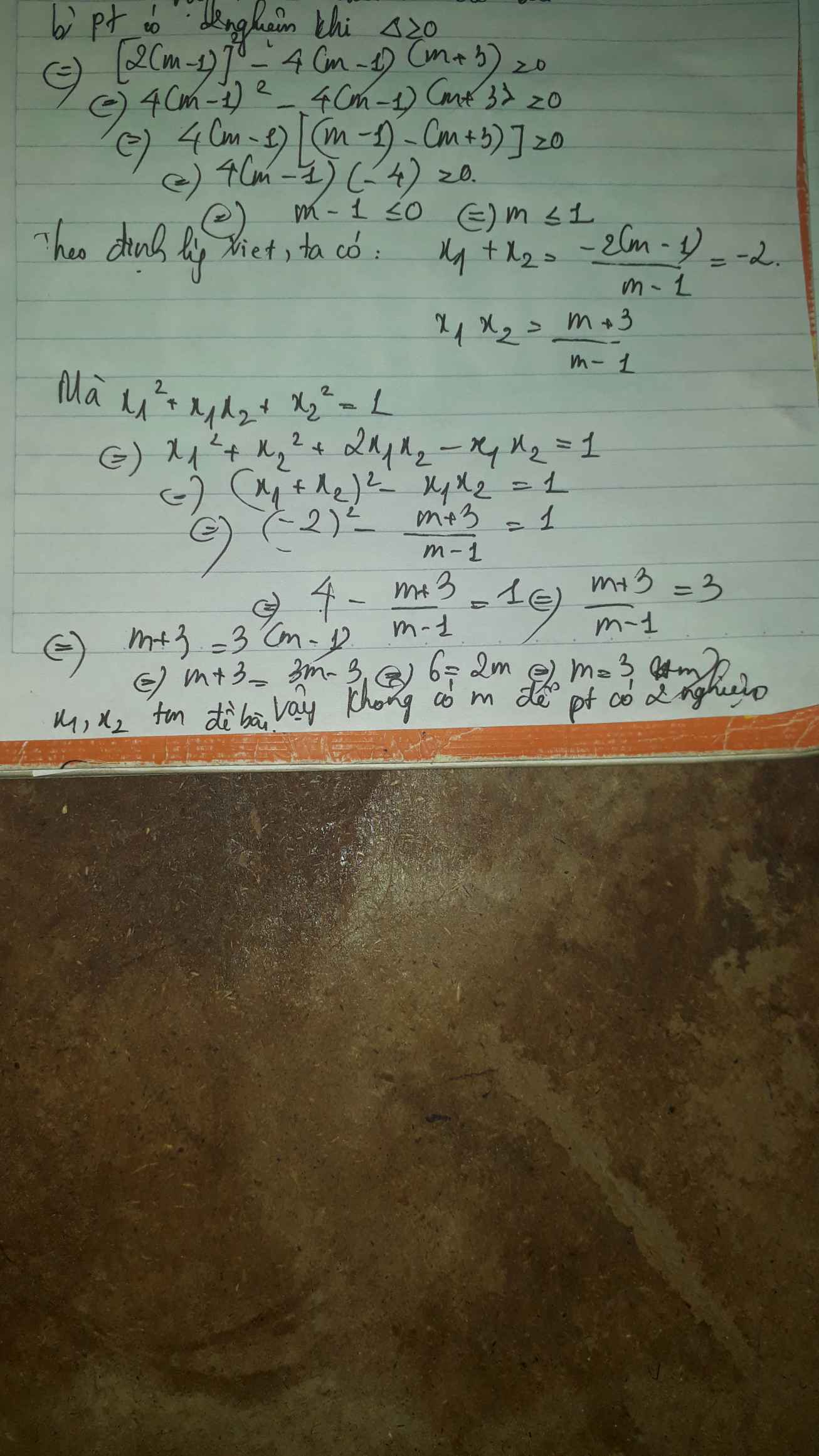
x2
−mx−2(m2
+8)=0
a= 1; b= -m; c= -2(m2+8)
+) Xét: Δ=b2-4ac=m2+4.2(m2+8)=m2+8m2+64=9m2+64
Vì 9m2+64>0 nên PT luôn có 2 nghiệm phân biệt.
+) Theo Viet ta có:
S=..=m và P=...=-2(m2+8)
Mà ta có: x12+x22=52
Hay: (x1+x2)2-2x1.x2=52 (1)
Hay m2- 2[-2(m2+8)]=52 (Thay S=..=m và P=...=-2(m2+8) vào (1))
⇔m2+4m2+32=52 ⇔5m2=20⇔m2=4⇔m=\(\pm\)2 (thỏa mãn)
Vậy với m =\(\pm\)2 thì PT có 2 nghiệm thỏa mãn đề bài
m=±2