Tìm giá trị nhỏ nhất của hàm số y = x 2 ln x trên đoạn 1 e ; e
A. m i n 1 e ; e y = - 1 e 2
B. m i n 1 e ; e y = - 1 2 e
C. m i n 1 e ; e y = - e
D. m i n 1 e ; e y = - 1 e
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

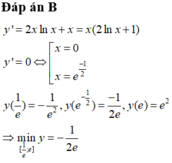

Đáp án A
Ta có: y ' = 1 − 1 x = 0 ⇔ x − 1 x = 0 ⇔ x = 1 . Ta có y 1 2 = 1 2 + ln 2 ; y 1 = 1 ; y e = e − 1
⇒ M a x y = e − 1 ; M i n y = 1

Ta có :
\(f'\left(x\right)=2x\ln x-x=x\left(2\ln x-1\right)=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=0\\\ln x=\frac{1}{2}\ln\sqrt{e}\end{array}\right.\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=0\notin\left[\frac{1}{e};e^2\right]\\x=\sqrt{e}\in\left[\frac{1}{e};e^2\right]\end{array}\right.\)
Mà : \(\begin{cases}f\left(\frac{1}{e}\right)=-\frac{1}{e^2}\\f\left(e\right)=\frac{e}{2}\\f\left(e^2\right)=2e^4\end{cases}\) \(\Rightarrow\begin{cases}Max_{x\in\left[\frac{1}{e};e^2\right]}f\left(x\right)=2e^4;x=e^2\\Min_{x\in\left[\frac{1}{e};e^2\right]}f\left(x\right)=\frac{-1}{e^2};x=\frac{1}{e}\end{cases}\)

Ta có :
\(f'\left(x\right)=\frac{-\frac{\frac{1}{x}}{2\sqrt{\ln x}}}{\ln x}=-\frac{1}{2x\ln x\sqrt{\ln x}}< 0\) với mọi \(x\in\left[e;e^2\right]\Rightarrow\) hàm số nghịch biến với mọi \(x\in\left[e;e^2\right]\)
\(e\le x\le e^2\Rightarrow f\left(e\right)\ge f\left(x\right)\ge f\left(e^2\right)\Leftrightarrow1\ge f\left(x\right)\ge\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow\begin{cases}Max_{x\in\left[e;e^2\right]}f\left(x\right)=1;x=e\\Min_{x\in\left[e;e^2\right]}f\left(x\right)=\frac{\sqrt{2}}{2};x=e^2\end{cases}\)
\(f\left(x\right)=\left(\ln x\right)^{-\frac{1}{2}}\Rightarrow f'\left(x\right)=-\frac{1}{2}\left(\ln x\right)^{-\frac{3}{2}}.\frac{1}{x}=-\frac{1}{2x\ln x\sqrt{\ln x}}\)
Ta có : \(\begin{cases}f\left(e\right)=1\\f\left(e^2\right)=\frac{\sqrt{2}}{2}\end{cases}\)
\(\Leftrightarrow\begin{cases}Max_{x\in\left[e;e^2\right]}f\left(x\right)=1;x=e\\Min_{x\in\left[e;e^2\right]}f\left(x\right)=\frac{\sqrt{2}}{2};x=e^2\end{cases}\)
