Tìm số hạng đầu và công sai của các cấp số cộng sau, biết: u 7 - u 3 = 8 u 2 . u 7 = 75
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
27 tháng 10 2023
Theo đề, ta có: \(S_n=3003\)
=>\(n\cdot\dfrac{\left[2u1+\left(n-1\right)\cdot d\right]}{2}=3003\)
=>\(\dfrac{n\left[2+\left(n-1\right)\right]}{2}=3003\)
=>n(n+1)=6006
=>n^2+n-6006=0
=>(n-77)(n+78)=0
=>n=77(nhận) hoặc n=-78(loại)
Vậy: n=77
CM
3 tháng 7 2017
Ta có : u3 = u1 + 2d ;
u5 = u1 + 4d ;
u6 = u1 + 5d
Theo đề bài ta có :
3 tháng 11 2023
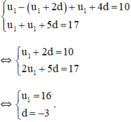
a) \(\left\{{}\begin{matrix}u_2-u_3+u_5=10\\u_4+u_6=26\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1+d-u_1-2d+u_1+4d=10\\u_1+3d+u_1+5d=26\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1+3d=10\\2u_1+8d=26\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1=1\\d=3\end{matrix}\right.\)
b)\(\left\{{}\begin{matrix}u_2-u_6+u_4=-7\\u_8-2u_7=2u_4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1+d-u_1-5d+u_1+3d=-7\\u_1+7d-2\left(u_1+6d\right)=2\left(u_1+3d\right)\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1-d=-7\\-3u_1-11d=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1=\dfrac{-11}{2}\\d=\dfrac{3}{2}\end{matrix}\right.\)
c)\(\left\{{}\begin{matrix}u_7-u_3=8\\u_2.u_7=75\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1+6d-u_1-2d=8\\\left(u_1+d\right)\left(u_1+6d\right)=75\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4d=8\\\left(u_1+d\right)\left(u_1+6d\right)=75\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}d=2\\\left(u_1+2\right)\left(u_1+12\right)=75\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}d=2\\u_1^2+14u_1+24=75\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}d=2\\\left[{}\begin{matrix}u_1=3\\u_1=-17\end{matrix}\right.\end{matrix}\right.\)
HQ
Hà Quang Minh
Giáo viên
21 tháng 9 2023
a) Dãy số trên là cấp số cộng
Ta có:
\(\begin{array}{l}{u_n} = {u_1} + \left( {n - 1} \right)d \Rightarrow {u_1} + \left( {n - 1} \right)d = 3 - 2n\\ \Leftrightarrow {u_1} + nd - d = 3 - 2n\\ \Leftrightarrow \left\{ \begin{array}{l}{u_1} - d = 3\\nd = - 2n\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}{u_1} = 1\\d = - 2\end{array} \right.\end{array}\)
b) Dãy số trên là cấp số cộng
Ta có:
\(\begin{array}{l}{u_n} = {u_1} + \left( {n - 1} \right)d \Rightarrow {u_1} + \left( {n - 1} \right)d = \frac{{3n + 7}}{5}\\ \Leftrightarrow {u_1} + nd - d = \frac{{3n}}{5} + \frac{7}{5}\\ \Leftrightarrow \left\{ \begin{array}{l}{u_1} - d = \frac{7}{5}\\nd = \frac{3}{5}n\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}{u_1} = 2\\d = \frac{3}{5}\end{array} \right.\end{array}\)
c) Dãy số đã cho không là cấp số cộng
Ta có: \( u_{n+1} = 3^{n+1} = 3.3^n \)
Xét hiệu \( u_{n+1} – u_n = 3.3^n – 3^n = 2.3^n \) với n ∈ ℕ*
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
9 tháng 9 2023
1, Dãy a nha với d= 2
2,
\(u_1=3.1+1=4\\ u_2=3.2+1=7\\ d=u_2-u_1=7-4=3\)
CM
12 tháng 5 2019
Chọn C
- Theo đầu bài ta có: u 1 = - 15 ; u 8 = 69 .
- Ta có:
28 tháng 10 2023
\(\left\{{}\begin{matrix}S_{15}=585\\u_1^3+\left(u_2\right)^3=302094\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}15\cdot\dfrac{2\cdot u_1+14d}{2}=585\\u_1^3+\left(u_1+d\right)^3=302094\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u_1+7d=39\\u_1^3+\left(u_1+d\right)^3=302094\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u_1=39-7d\\\left(39-7d\right)^3+\left(39-7d+d\right)^3=302094\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u_1=39-7d\\59319-31941d^2+5733d-343d^3+59319-18252d^2+2808d-216d^3=302094\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u_1=39-7d\\118638-50193d+8541d^2-559d^3=302094\end{matrix}\right.\)
=>d=-2,46(loại)
Vậy: Không có bộ số số hạng đầu và công sai nào thỏa mãn đề bài
28 tháng 10 2023
\(\left\{{}\begin{matrix}u2-3u8=-20\\u_3\cdot u_4=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1+d-3\left(u1+7d\right)=-20\\u3\cdot u4=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-2u1-20d=-20\\u3\cdot u4=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1+10d=10\\\left(u1+2d\right)\cdot\left(u1+3d\right)=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1=10-10d\\\left(10-10d+2d\right)\left(10-10d+3d\right)=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1=10-10d\\\left(-8d+10\right)\left(-7d+10\right)=24\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u1=10-10d\\56d^2-150d+126=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}d\in\varnothing\\u1=10-10d\end{matrix}\right.\)
=>Không có số hạng đầu và công sai nào thỏa mãn yêu cầu đề bài
Ta có: u7 = u1 + 6d ; u3 = u1 + 2d ; u2 = u1 + d
Do đó theo đề bài ta có: