cho x + 1/x = 3 . tính giá trị biểu thức A = x^3 - 1/x^3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
TM
26 tháng 1 2022
1. ĐKXĐ: \(x\ne\pm1\)
2. \(A=\left(\dfrac{x+1}{x-1}-\dfrac{x+3}{x+1}\right)\cdot\dfrac{x+1}{2}\)
\(=\dfrac{\left(x+1\right)^2-\left(x-3\right)\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{x+1}{2}\)
\(=\dfrac{x^2+2x+1-x^2+4x-3}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{x+1}{2}\)
\(=\dfrac{6x-2}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{x+1}{2}\)
\(=\dfrac{2\left(x-3\right)\left(x+1\right)}{2\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x-3}{x-1}\)
3. Tại x = 5, A có giá trị là:
\(\dfrac{5-3}{5-1}=\dfrac{1}{2}\)
4. \(A=\dfrac{x-3}{x-1}\) \(=\dfrac{x-1-3}{x-1}=1-\dfrac{3}{x-1}\)
Để A nguyên => \(3⋮\left(x-1\right)\) hay \(\left(x-1\right)\inƯ\left(3\right)=\left\{1;-1;3;-3\right\}\)
\(\Rightarrow\left\{{}\begin{matrix}x-1=1\\x-1=-1\\x-1=3\\x-1=-3\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=2\left(tmđk\right)\\x=0\left(tmđk\right)\\x=4\left(tmđk\right)\\x=-2\left(tmđk\right)\end{matrix}\right.\)
Vậy: A nguyên khi \(x=\left\{2;0;4;-2\right\}\)
NT
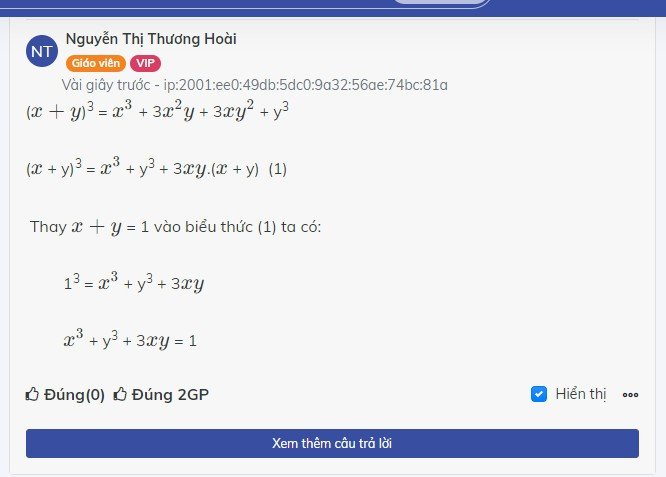
a) cho x+y=1. Tính giá trị biểu thức x^3+ y^3+ 3xy
b) cho x-y=1. Tính giá trị biểu thức x^3- y^3- 3xy
2
BT
30 tháng 6 2018
x^3+ y^3+ 3xy
=(x+y)(x^2 -xy + y^2 ) + 3xy
=x^2 -xy + y^2 + 3xy
=x^2 + 2xy + y^2
=(x+y)^2 =1
=> x^3+ y^3+ 3xy=1
18 tháng 4 2022
a: Ta có: |x+4|=1
=>x+4=1 hoặc x+4=-1
=>x=-3(loại) hoặc x=-5
Khi x=-5 thì \(A=\dfrac{\left(-5\right)^2-5}{3\left(-5+3\right)}=\dfrac{20}{3\cdot\left(-2\right)}=\dfrac{-10}{3}\)
b: \(B=\dfrac{x-1+x+1-3+x}{\left(x-1\right)\left(x+1\right)}=\dfrac{3x-3}{\left(x-1\right)\left(x+1\right)}=\dfrac{3}{x+1}\)
1) Cho biểu thức A =
7 tháng 9 2021
Bài 5:
a: Thay \(x=4+2\sqrt{3}\) vào E, ta được:
\(E=\dfrac{\sqrt{3}+1-1}{\sqrt{3}+1-3}=\dfrac{\sqrt{3}}{\sqrt{3}-2}=-3-2\sqrt{3}\)
b: Để E<1 thì E-1<0
\(\Leftrightarrow\dfrac{\sqrt{x}-1-\sqrt{x}+3}{\sqrt{x}-3}< 0\)
\(\Leftrightarrow\sqrt{x}-3< 0\)
hay x<9
Kết hợp ĐKXĐ, ta được: \(\left\{{}\begin{matrix}0\le x< 9\\x\ne1\end{matrix}\right.\)
c: Để E nguyên thì \(4⋮\sqrt{x}-3\)
\(\Leftrightarrow\sqrt{x}-3\in\left\{-2;1;2;4\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{4;5;7\right\}\)
hay \(x\in\left\{16;25;49\right\}\)
NT
7 tháng 9 2021
Câu 2:
a) Ta có \(x=4-2\sqrt{3}\Rightarrow\sqrt{x}=\sqrt{\left(\sqrt{3}-2\right)^2}=\sqrt{3}-2\)
Thay \(x=\sqrt{3}-1\) vào \(B\), ta được
\(B=\dfrac{\sqrt{3}-1-2}{\sqrt{3}-1+1}=\dfrac{\sqrt{3}-3}{\sqrt{3}}=1-\sqrt{3}\)
b) Để \(B\) âm thì \(\dfrac{\sqrt{x}-2}{\sqrt{x}+1}< 0\) mà \(\sqrt{x}+1\ge1>0\forall x\) \(\Rightarrow\sqrt{x}-2< 0\Rightarrow\sqrt{x}< 2\Rightarrow x< 4\)
c) Ta có \(B=\dfrac{\sqrt{x}-2}{\sqrt{x}+1}=1-\dfrac{3}{\sqrt{x}+1}\)
Với mọi \(x\ge0\) thì \(\sqrt{x}\ge0\Rightarrow\sqrt{x}+1\ge1\Rightarrow\dfrac{3}{\sqrt{x}+1}\le3\Rightarrow B=1-\dfrac{3}{\sqrt{x}+1}\ge-2\)
Dấu "=" xảy ra khi \(\sqrt{x}+1=1\Leftrightarrow x=0\)
Vậy \(B_{min}=-2\) khi \(x=0\)
MN
8 tháng 4 2020
a) Thay x = -1 và y = 3 vào A, ta được :
A = 2.(-1)[(-1) + 3] - (-1) + 7 - 3
A = -2.2 + 1 + 4
A = -4 + 5
A = 1
b) |y| = 3 => \(\orbr{\begin{cases}y=3\\y=-3\end{cases}}\)
*Thay x =-1 và y = 3 vào biểu thức :
Phần này bạn sẽ làm ý như câu a vậy :33
*Thay x = -1 và y =-3 vào A, ta được :
A = 2.(-1).[(-1) + (-3)] - (-1) + 7 - (-3)
A = -2.(-4) + 1 + 7 + 3
A = 8 + 11
A = 19
TV
1
9 tháng 11 2021
\(A=\left(x+\dfrac{1}{x}\right)^3-3x\cdot\dfrac{1}{x}\left(x+\dfrac{1}{x}\right)=a^3-3\cdot1\cdot a=a^3-3a\)
NT
2
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
12 tháng 7
b; 13 = (\(x-y\))3 = \(x^3\) - 3\(x^2\).y + 3\(xy^2\) - y3 = \(x^3\) - y3 - 3\(xy\)(\(x-y\))
1 = \(x^3\) - y3 - 3\(xy\)
4 tháng 12 2021
a: \(A=\left(\dfrac{x}{x^2-4}+\dfrac{4}{x-2}+\dfrac{1}{x+2}\right):\dfrac{3x+3}{x^2+2x}\)
\(=\dfrac{x+4x+8+x-2}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{x\left(x+2\right)}{3\left(x+1\right)}\)
\(=\dfrac{6\left(x+1\right)\cdot x\left(x+2\right)}{3\left(x+1\right)\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2x}{x-2}\)
ta có \(\frac{x+1}{x}=3\Rightarrow3x=x+1\)
\(\Rightarrow3x-x=1\)
\(\Leftrightarrow2x=1\)
\(\Rightarrow x=\frac{1}{2}\)
Thay \(x=\frac{1}{2}\)vào biểu thức A ta có :
\(A=\frac{\left(\frac{1}{2}\right)^3-1}{\left(\frac{1}{2}\right)^3}=\frac{\frac{1}{8}-1}{\frac{1}{8}}=\frac{\frac{-7}{8}}{\frac{1}{8}}=-7\)
Vậy A= -7
Chúc bn hk tốt nha ^_^