Phân tích đa thức thành nhân tử:\(x\sqrt{x}-3x+4\sqrt{x}-2\)\(\left(x>0\right)\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
4
HM
2
21 tháng 8 2018
\(2x^2-3x\sqrt{x+3}+\left(x+3\right)\)
\(=2x^2-2x\sqrt{x+3}-x\sqrt{x+3}+\left(\sqrt{x+3}\right)^2\)
\(=2x\left(x-\sqrt{x+3}\right)-\sqrt{x+3}\left(x-\sqrt{x+3}\right)\)
\(=\left(2x-\sqrt{x+3}\right)\left(x-\sqrt{x+3}\right)\)
21 tháng 8 2018
\(2x^2-3x\sqrt{x+3}+\left(x+3\right)\)
\(=2x^2-x\sqrt{x+3}-2x\sqrt{x+3}+\left(\sqrt{x+3}\right)^2\)
\(=x\left(2x-\sqrt{x+3}\right)-\sqrt{x+3}\left(2x-\sqrt{x+3}\right)\)
\(=\left(x-\sqrt{x+3}\right)\left(2x-\sqrt{x+3}\right)\)
HT
2
MW
31 tháng 10 2017
\(=x+2\sqrt{xy}+y-9\)
\(=\left(\sqrt{x}+\sqrt{y}\right)^2-3^2\)
\(=\left(\sqrt{x}+\sqrt{y}-3\right)\left(\sqrt{x}+\sqrt{y}+3\right)\)
PT
0
19 tháng 7 2021
a) \(x^3+9x^2+27x+27=\left(x+3\right)^3\)
b) \(3\sqrt{3x^3}+18x^2+12\sqrt{3x}+8=\left(\sqrt{3x}+2\right)^3\)
c) \(\dfrac{1}{4}-x^2=\left(\dfrac{1}{2}-x\right)\left(\dfrac{1}{2}+x\right)\)
DA
1
AH
Akai Haruma
Giáo viên
28 tháng 9
Lời giải:
$x\sqrt{x}-3x+4\sqrt{x}-2=(x\sqrt{x}-x)-(2x-2\sqrt{x})+(2\sqrt{x}-2)$
$=x(\sqrt{x}-1)+2\sqrt{x}(\sqrt{x}-1)+2(\sqrt{x}-1)$
$=(\sqrt{x}-1)(x+2\sqrt{x}+2)$
14 tháng 10 2020
Đến đây là PT tích r còn gì, \(x\in\left\{5;-10;-\sqrt{3}\right\}\)
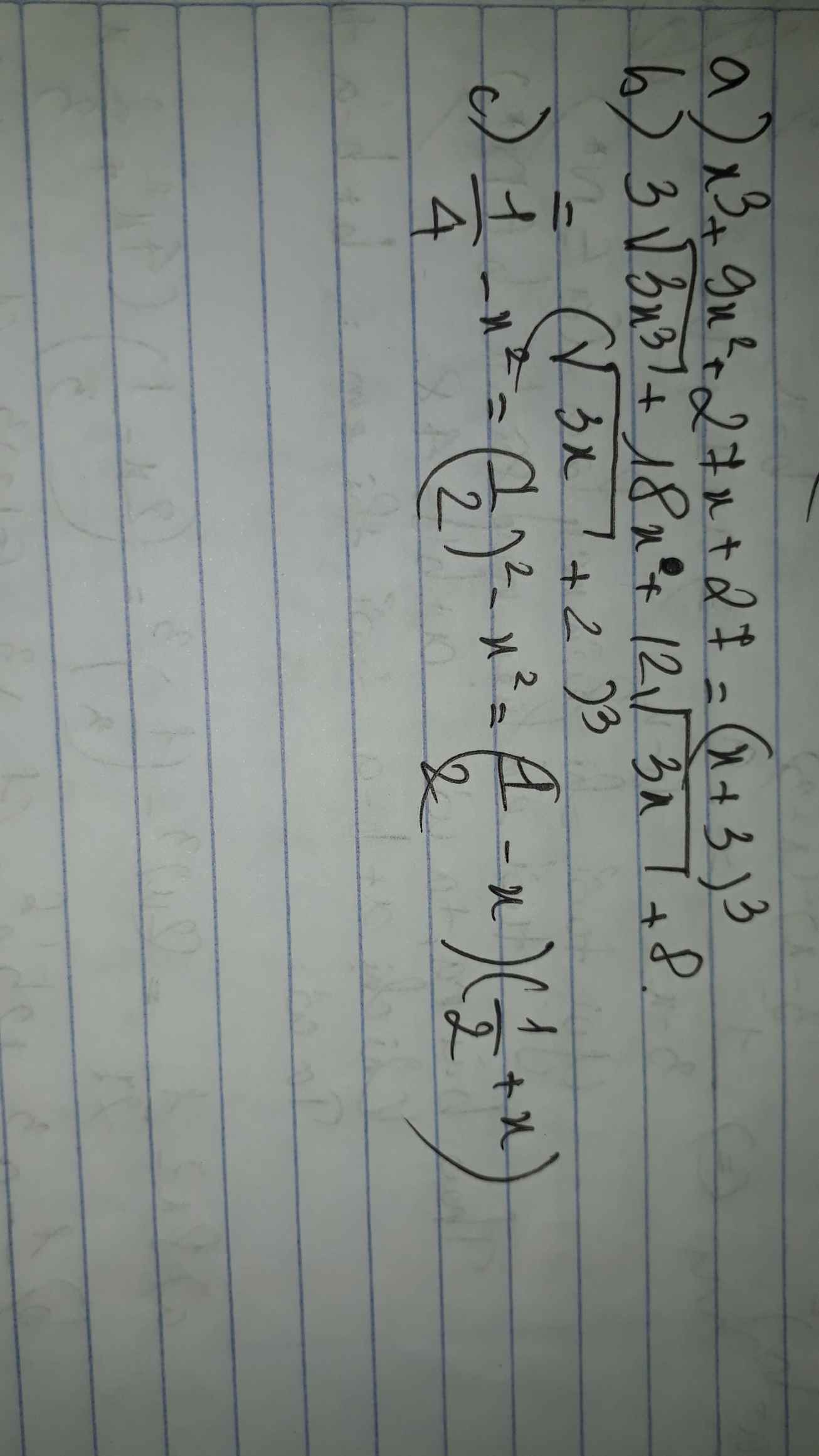
\(x\sqrt{x}-3x+4\sqrt{x}-2=x\sqrt{x}-x-2x+2\sqrt{x}+2\sqrt{x}-2\)
\(=x\left(\sqrt{x}-1\right)-2\sqrt{x}\left(\sqrt{x}-1\right)+2\left(\sqrt{x}-1\right)\)
\(=\left(\sqrt{x}-1\right)\left(x-2\sqrt{x}+2\right)\)